
Question and Answers Forum
AllQuestion and Answers: Page 1637





Pg 1632 Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638 Pg 1639 Pg 1640 Pg 1641
|
Question and Answers Forum |
AllQuestion and Answers: Page 1637 |
| Find the value of k if (2,k) ,(3,4) and (6,4) are collinear. hence find the equation on the line 3i − j with the above points |
| calculate ∫_0 ^∞ (dt/(1+t^(4 ) +t^6 )) |
| Find the value of k if (d/dx)(f(x))= 6 when x = −1 and f(x) = 3x^3 − kx^2 + 1 if f(2) = 8 find one factor of f(x) and hence Evaluate lim_(x→∞) (f(x)). |

|
| F(x) = x^3 −9x^2 +24x+c=0 has three real and distinct roots α , β & γ . Q.1 → Possible value of c is : Q.2 → If [α]+[β]+[γ]= 8 then c is : Q.3 → If [α]+[β]+[γ]=7 then c is : Options for the above 3 Q. → a) (−20,−16) b) (−20,−18) c) (−18,−16) d) none of these. [.] = greatest integer function. |
| calculate A(λ) = ∫_0 ^λ ((ln(x+(√(1+x^2 ))))/(√(1+x^2 ))) dx 2) calculate ∫_0 ^1 ((ln(x+(√(1+x^2 ))))/(√(1+x^2 )))dx |
| Given the lines l_1 : x + y = 5 and l_2 : y = 4x and l_3 ; 4x + y − 1 =0 show that l_(2 ) is perpendicular to l_3 . find the point coordinates if x + 2y = 5 is colliner to l_1 |
| f(x) = 3x^3 − 2x + k has factor (x − 1) find the value of k. with these value evaluate a) (d/(dx ))(f(x)_ ) b) ∫_5 ^2 (f(x)) |
| Find domain of (1+(1/x))^x ? Also prove thatL_(x→0^+ ) (1+(1/x))^x = 1 ? |
| let A_n = ∫_1 ^n (([(√(1+x^2 ))] −[x])/x^2 ) dx (n integr ≥1) 1) calculate A_n 2) find lim_(n→+∞) A_n |
| calculate ∫_(−∞) ^(+∞) ((x^2 cos(4x))/((x^2 +1)^2 ))dx |

|

|
| Without using l′hopital find lim_(x→3) ((√(9−x^2 ))/(x−3)) |

|

|
|
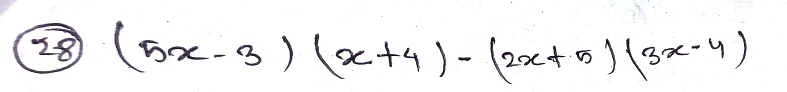
|

|
| find F(x) = ∫_0 ^π ln(x^2 −2x sin(2θ) +1)dθ . |
| let f(x) =(1/(1+∣sinx∣)) (2π periodic even) developp f at fourier serie . |
| let f(z) = (z/(z^2 −z+2)) developp f at integr serie. |
| calculate F(x)=∫_0 ^(2π) ((cos(4t))/(x^2 −2x cost +1)) dt |
| find f(t) =∫_0 ^∞ sin(x)e^(−t [x]) dx with t>0 |
| calculate interms of n A_n = ∫_0 ^(2π) ((cos(nx))/(cosx +sinx))dx and B_n = ∫_0 ^(2π) ((sin(nx))/(cosx +sinx))dx . |
| calculate ∫_(−∞) ^(+∞) ((xsin(2x))/((1+x^2 )^2 ))dx |
Pg 1632 Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638 Pg 1639 Pg 1640 Pg 1641 |