
Question and Answers Forum
AllQuestion and Answers: Page 1638



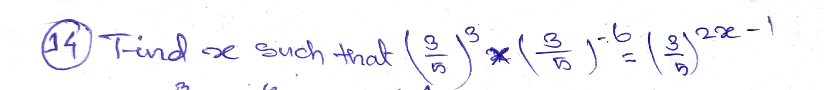
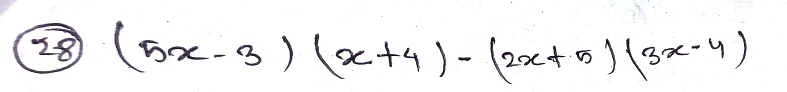

Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638 Pg 1639 Pg 1640 Pg 1641 Pg 1642
|
Question and Answers Forum |
AllQuestion and Answers: Page 1638 |

|

|
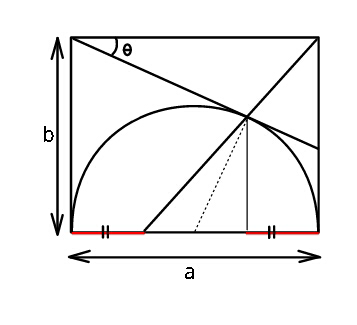
| Without using l′hopital find lim_(x→3) ((√(9−x^2 ))/(x−3)) |

|

|
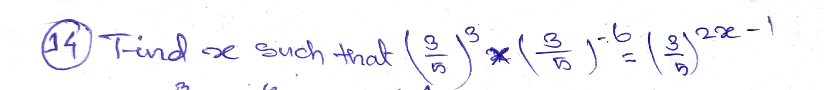
|
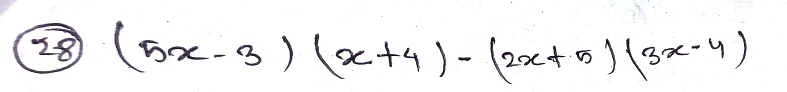
|

|
| find F(x) = ∫_0 ^π ln(x^2 −2x sin(2θ) +1)dθ . |
| let f(x) =(1/(1+∣sinx∣)) (2π periodic even) developp f at fourier serie . |
| let f(z) = (z/(z^2 −z+2)) developp f at integr serie. |
| calculate F(x)=∫_0 ^(2π) ((cos(4t))/(x^2 −2x cost +1)) dt |
| find f(t) =∫_0 ^∞ sin(x)e^(−t [x]) dx with t>0 |
| calculate interms of n A_n = ∫_0 ^(2π) ((cos(nx))/(cosx +sinx))dx and B_n = ∫_0 ^(2π) ((sin(nx))/(cosx +sinx))dx . |
| calculate ∫_(−∞) ^(+∞) ((xsin(2x))/((1+x^2 )^2 ))dx |
| x+y=3 x=2 y=? |
| 1) calculate A=cos((π/7)).cos(((2π)/7)).cos(((3π)/7)) 2) calculate B =tan((π/7)).tan(((2π)/7)).tan(((3π)/7)). |
| find the roots of 8x^3 −4x−1 =0 |
| let f(x)= ((cos(αx))/(cosx)) (2π periodic even) developp f at fourier serie. |
| find the value of I = ∫_0 ^1 ((arctan(2x))/(√(1+4x^2 ))) dx |
| let g(x)= ∫_(−∞) ^(+∞) ((arctan(x(1+t^2 )))/(1+t^2 ))dt with x>0 find a simple form of g(x) . |
| let p(x)= (1+e^(iθ) x)^n −(1−e^(iθ) x)^n with n integr natural 1) find the roots of p(x) 2) fctorize inside C[x] p(x) 3) factorize inside R[x] p(x). θ ∈R |
| calculate A_n = ∫_0 ^1 sin(narctanx)dx with n integr natural. 2) find nature of Σ_n A_n |
| calculate ∫_0 ^1 ((ln(1+(√(x^2 +1))))/(√(x^2 +1))) dx |
| calculate ∫ (dx/((x^2 +1)(x^2 +2)(x^2 +3))) 1) find the value of ∫_0 ^∞ (dx/((x^2 +1)(x^2 +2)(x^2 +3))) |
| find nature of Σ_(n=0) ^∞ (((−1)^([x]) )/(2+cos(n[x]))) |
Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638 Pg 1639 Pg 1640 Pg 1641 Pg 1642 |