
Question and Answers Forum
AllQuestion and Answers: Page 1666




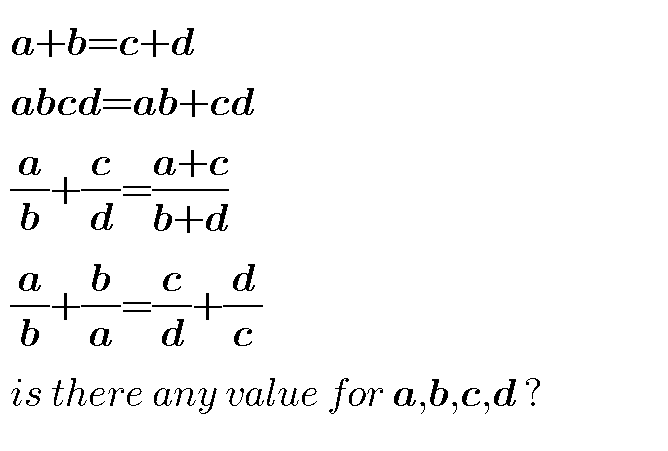

Pg 1661 Pg 1662 Pg 1663 Pg 1664 Pg 1665 Pg 1666 Pg 1667 Pg 1668 Pg 1669 Pg 1670
|
Question and Answers Forum |
AllQuestion and Answers: Page 1666 |

|
| In an organic Compound Carbon = 85.7% Hydrogen = 14.3% and RMM= 56 find the molecular formular. |
| decompose inside C(x) the fraction F(x)= (1/((x^2 +1)^n )) with n integr nstural. |
| let p(x) =x^n −e^(inα) with n integr and α fromR 1) find the roots of p(x) 2) factorize p(x) inside C[x] . |

|

|
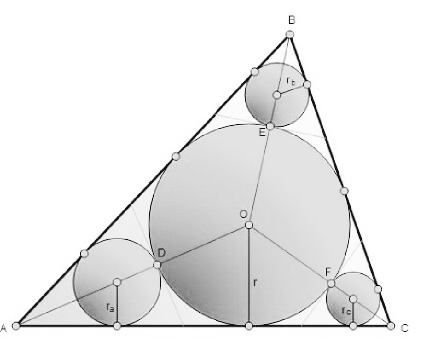
| y=(((x−3)(x+5)^2 )/(x^2 (x+2))) Find local maxima and minima; hence draw the graph.Also find radius of circle touching all three sections of the curve that results. |
| prove (1+(1/1^2 ))(1+(1/2^2 ))(1+(1/3^2 ))...upto infinity =(1/Π)sinhΠ |
| find f(x)= ∫_0 ^∞ arctan(xt^2 )dt with x>0 |
| find f(t) = ∫_0 ^1 arctan(tx^2 )dx with t≥0 developp f at integr serie |
| find the perimeter of a right angle triangle 4xcm high and 5xcm base |
| Find domain and range of the function f(x)=((x^2 −6x+8)/(x−5)) . Also draw the graph. |

|
| find the image of (−2,3) after the reflection on the a) x−axis b) y−axis |
| Find the angle between the lines l_1 :y − x −4 = 0 and l_2 :2x − y = 7 and hence the perpendicular distance from one point on l_1 to l_2 . |
| Consider a particle in a uniformly charge electric field, if the particle has a charge of 2C and is place 3m away from the charge plate. calculate the work needed to move the 2C particle to a distance of 1m from the plate. |

|
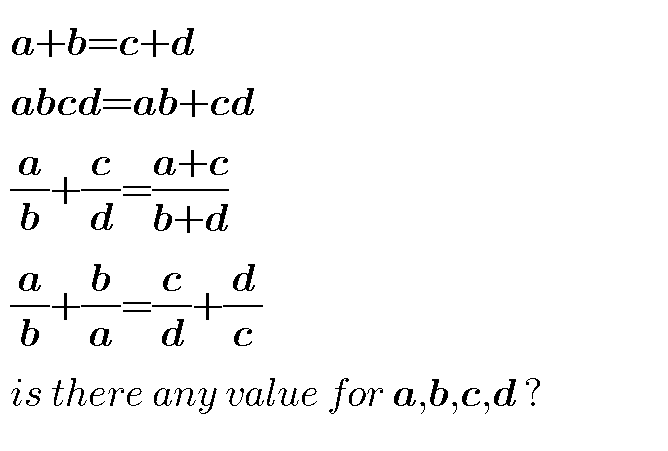
|
| What is the absolute value of ( −10)? |
| the opposite side of a triangle is x + y,and the hypotenuse is 3x+y Given that that A is an acute angle find the value of Cos A |

|
| 1×10^(7 ) electrons pass through a conductor in 1.0μs.Find the current in Amperes flowing through the conductor. electronic charge=1.6×10^(−19) C |
| a(b+(1/b))=−1 ....(i) b−(1/b)=a^2 ....(ii) Solve simultaneously for a, and b. |
| in question 34992 this had to be solved: t^4 +8t^3 +2t^2 −8t+1=0 here another concept worked (I tried some) t_1 =a+b+c+d t_2 =a+b−c−d t_3 =a−b+c−d t_3 =a−b−c+d (t−t_1 )(t−t_2 )(t−t_3 )(t−t_4 )=0 leads to t^4 −4at^3 +2(3a^2 −b^2 −c^2 −d^2 )t^2 + +4(a(−a^2 +b^2 +c^2 +d^2 )−2bcd)t+ +a^4 +b^4 +c^4 +d^4 −2(a^2 (b^2 +c^2 +d^2 )+b^2 (c^2 +d^2 )+c^2 d^2 ) 1. −4a=8 2. 2(3a^2 −b^2 −c^2 −d^2 )=2 3. 4(a(−a^2 +b^2 +c^2 +d^2 )−2bcd)=−8 4. a^4 +b^4 +c^4 +d^4 −2(a^2 (b^2 +c^2 +d^2 )+b^2 (c^2 +d^2 )+c^2 d^2 )=1 1. a=−2 2. b^2 +c^2 +d^2 =11 3. b^2 +c^2 +d^2 +bcd=5 4. ... 3. −2. bcd=−6 now I tried by chance if there are easy real solutions b^2 =p, c^2 =q, d^2 =r, 0≤p≤q≤r p+q+r=11 this leads to p=2, q=3, p=6 ∣b∣=(√2), ∣c∣=(√3), ∣d∣=(√6) with one or three out of a, b, c negative because of bcd=−6 because of the nature of t_1 , t_2 , t_3 , t_4 these lead to the same solutions I took b=−(√2), c=−(√3), d=−(√6) t_1 =−2−(√2)−(√3)−(√6) t_2 =−2−(√2)+(√3)+(√6) t_3 =−2+(√2)−(√3)+(√6) t_4 =−2+(√2)+(√3)−(√6) |
| proof that 2((√n) − 1) < 1 + (1/((√2) )) + (1/(√3))+...+ (1/(√n)) < 2(√n) |
Pg 1661 Pg 1662 Pg 1663 Pg 1664 Pg 1665 Pg 1666 Pg 1667 Pg 1668 Pg 1669 Pg 1670 |