
Question and Answers Forum
AllQuestion and Answers: Page 1746



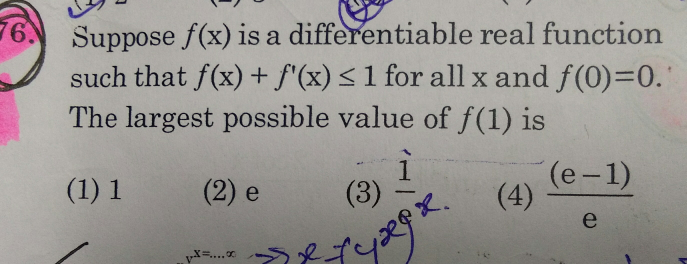

Pg 1741 Pg 1742 Pg 1743 Pg 1744 Pg 1745 Pg 1746 Pg 1747 Pg 1748 Pg 1749 Pg 1750
|
Question and Answers Forum |
AllQuestion and Answers: Page 1746 |
| find Σ_(k=0) ^n cos(kx) and Σ_(k=0) ^n sin(kx) . |
| θ ∈]0,π[ find he values of Σ_(n=1) ^∞ (1/n)cos(nθ) and Σ_(n=1) ^∞ (1/n)sin(nθ) . |
| give the developpement at integr series for f(x)=((ln(1+x)−ln(1−x))/x) 2)find lim_(x→0) f(x). |
| find lim_(x→0) ((tanx −x−(1/3)x^3 )/x^5 ) . |
| let give f_α (t)=cos(αt) 2π periodic with t ∈[−π,π]and α∈ R−Z 1) developp f_α at fourier serie and prove that cotan(απ)= (1/(απ)) +Σ_(n=1) ^∞ ((2α)/(π(α^2 −n^2 ))) 2)let x∈]0,π[ ant g(t)=cotant −(1/t) if t∈]0,x]andg(0)=0 prove that g is continue in[0,x] and find ∫_0 ^x g(t)dt 3)prove that ∀ t∈[0,x] g(t)=2t Σ_(n=1) ^∞ (1/(t^2 −n^2 π^(24) )) 4) chow that Π_(n=1) ^∞ (1−(x^2 /(n^2 π^2 )))= ((sinx)/x) and for x∈]−π,π[ sinx=x Π_(n=1) ^∞ (1−(x^2 /(n^2 π^2 ))) . |
| prove that ∀ x∈]0,1[ (1/(Γ(x).Γ(1−x)))=x Π_(n=1) ^∞ (1−(x^2 /n^2 )). |
| prove that ∀ x∈]0,1[ Γ(x).Γ(1−x)= (π/(sin(πx))) (compliments formula). |
| ∫(1/(1+sinx))dx=? |
| find Π_(n=1) ^∞ (1−(x^2 /(n^2 π^2 ))). |
| find Π_(n=1) ^∞ (1−(1/(4n^2 ))). |
| let give T_n (x)=cos(n arcosx) with x∈[−1,1] 1) prove that T_n is a polynomial and T_n ∈Z[x] 2)calculate T_1 , T_2 , T_3 ,and T_4 3) prove that T_(n+2) (x)=2x T_(n+1) (x)−T_n (x) 4)find the roots of T_n and factorize T_n (x). |
| let give u_n = Σ_(q=1) ^n (1/(n^2 +q)) find lim_(n→+∞) (1−nu_n )n. |
| let give f(x)=−x +2 +((√(x+1))/x) 1) study the variation of and give the graph C_f 2)give the equation of tangent at C_f in point A(1,f(1)) |
| find (1/(cos^4 ((π/9)))) +(1/(cos^4 (((3π)/9)))) + (1/(cos^4 (((5π)/9)))) +(1/(cos^4 (((7π)/9)))) . |
| find cos^4 ((π/8)) +cos^4 (((3π)/8)) +cos^4 (((5π)/8)) +cos^4 (((7π)/8)). |
| p is a polynomial having n roots x_i with x_i ≠x_j for i≠j prove that Σ_(i=1) ^n ((p^(′′) (x_i ))/(p^′ (x_i )))=0 |
| ((sin 16x)/(sin x)) ?pls help. |

|
| f(x)=(x+a_1 )(x+a_2 )(x+a_3 )...(x+a_n ) find the coefficient of term x^k (0≤k≤n) |
| 4, 8, 16, 31, 57, 99, 163, T_8 ,T_9 , .... Find T_8 , T_9 . |
| Fluids: |
| let give f(x)= (1/(1+x^2 )) 1)prove that prove that f^((n)) (x)=((p_n (x))/((1+x^2 )^(n+1) )) with p_n is a polynomial 2) prove that p_(n+1) (x)=(1+x^2 )p_n ^′ (x) −2(n+1)p_n (x) 3) calculate p_0 (x) ,p_1 (x) ,p_2 (x) ,p_3 (x) . |

|

|
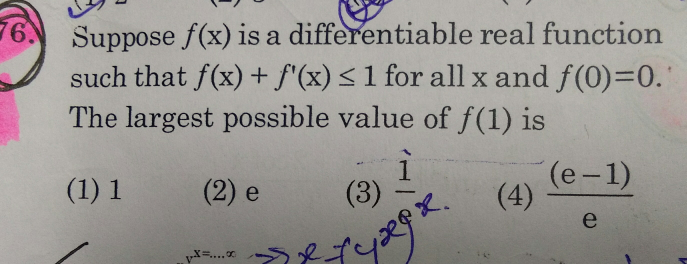
|

|
Pg 1741 Pg 1742 Pg 1743 Pg 1744 Pg 1745 Pg 1746 Pg 1747 Pg 1748 Pg 1749 Pg 1750 |