
Question and Answers Forum
AllQuestion and Answers: Page 373




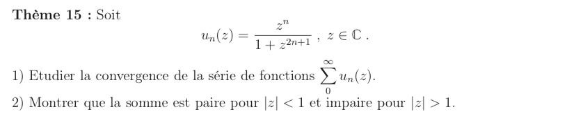

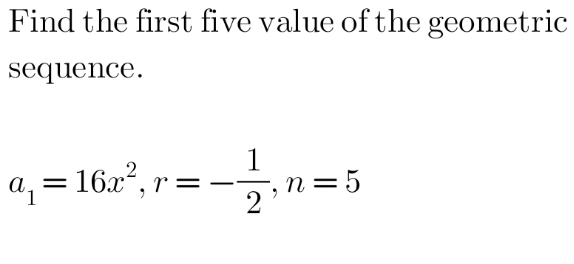


Pg 368 Pg 369 Pg 370 Pg 371 Pg 372 Pg 373 Pg 374 Pg 375 Pg 376 Pg 377
|
Question and Answers Forum |
AllQuestion and Answers: Page 373 |
| lim_(x→0) ((sin^2 (x)−sin (x^2 ))/(x^2 (cos^2 (x)−cos (x^2 ))))=? |

|
| { ((p^3 +q^3 =r^2 )),((p^3 +r^3 =q^2 )),((q^3 +r^3 =p^2 )) :} ⇒20pqr =? |

|

|
| look the anser |

|
| 𝛗=∫_0 ^( 1) (( ( tanh^( −1) (x))^2 )/((1+x )^( 2) )) dx = ? ≺ solution ≻ note : tanh^( −1) (x)=− (1/2) ln(((1−x)/(1+x))) 𝛗= (1/4)∫_0 ^( 1) (( ln^( 2) (((1−x)/(1+x)) ))/((1+x )^( 2) )) dx =^(((1−x)/(1+x)) = t) (1/8)∫_0 ^( 1) ln^( 2) (t )dt =(1/8_ ) { [t.ln^( 2) (t)]_0 ^( 1) −2∫_0 ^( 1) ln(t)dt} =− (1/4) ∫_0 ^( 1) ln(t)dt= (1/4) ◂ m.n ▶ |
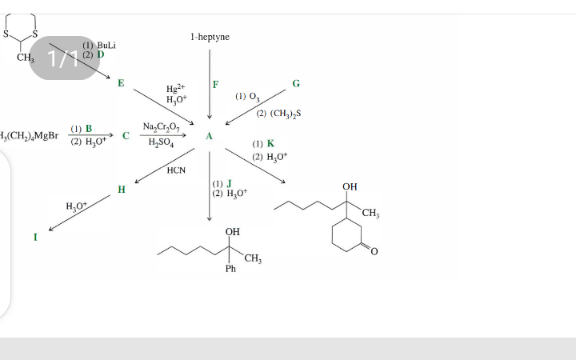
| Solve the equation: 2^x + 3^x − 4^x + 6^x − 9^x = 1 |
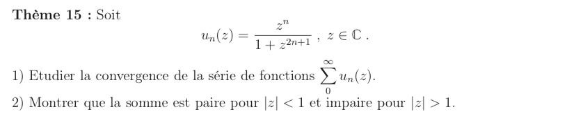
|
| x^3 +(1/x^3 )=1 (((x^5 +(1/x^5 ))^3 −1)/(x^5 +(1/x^5 )))=? Q#176387 reposted for a new answer. |
| Given { ((sin a+sin b=((√2)/2))),((cos a+cos b=((√6)/2))) :} for a,b real numbers. Evaluate sin (a+b). (A)((√3)/2) (D) −((√3)/2) (B) (2/( (√3))) (E)−(2/( (√3))) (C) ((√3)/4) |
| 4^(x^2 −2x+2) −2^(x^2 −2x+3) +2=2^(x^2 −2x+2) x=? |
| lim_(x→1) ((lnx)/(1+lnx−1))=? |
| (1) ∫^(π/2) _(π/3) ((1+sinx)/(cosx)) dx=? |
| −−−− calculate: Φ = Σ_(n=0) ^( ∞) (( 1)/((2n+1 ).e^( 4n+2) )) = ? where ” e ” is euler number. ≺ solution ≻ Φ = Σ_(n=0) ^∞ (1/e^( 4n+2) ) ∫_0 ^( 1) x^( 2n) dx = (1/e^( 2) ) ∫_0 ^( 1) Σ_(n=0) ^∞ ((( x^2 )/e^( 4) ) )^( n) dx = (1/e^( 2) ) ∫_0 ^( 1) (( 1)/(1− ((x/e^( 2) ) )^( 2) )) dx=(1/(2e^( 2) )) ∫_0 ^( 1) (1/(1−(x/e^( 2) ))) +(1/(1+(x/e^( 2) )))dx = (1/2) ln ( ((1+(1/e^( 2) ))/(1−(1/e^( 2) ))) ) = tanh^( −1) ((( 1)/e^( 2) ) ) ∴ Φ = coth^( −1) ( e^( 2) ) ■ m.n |

|
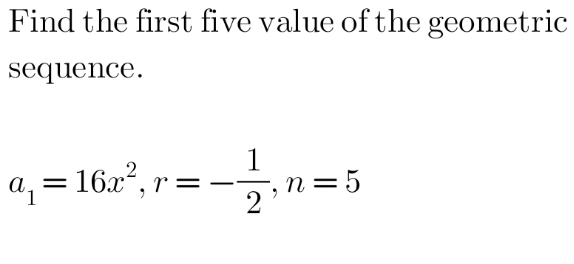
|
| is there an iOS version for this app? please |
| ABCD−convex quadrilateral M∈Int(ABCD) , F−area , s−semiperimetr a , b , c−sides. Prove that: ((MA^4 )/b) + ((MB^4 )/c^4 ) + ((MC^4 )/d) + ((MD^4 )/a) ≥ ((2F^2 )/s) |
| x + (1/x) =ϕ (Golden ratio) then x^( 2000) +(( 1)/x^( 2000) ) = ? |

|
| donner la forme trigonometrique de 1/4(cosΠ/9+isinΠ/9) |

|

|
| Ω = ∫_0 ^( 1) (( x.tanh^( −1) (x))/((1+x)^( 2) ))dx= (1/(24)) (π^( 2) −6) |
Pg 368 Pg 369 Pg 370 Pg 371 Pg 372 Pg 373 Pg 374 Pg 375 Pg 376 Pg 377 |