
Question and Answers Forum
AllQuestion and Answers: Page 383
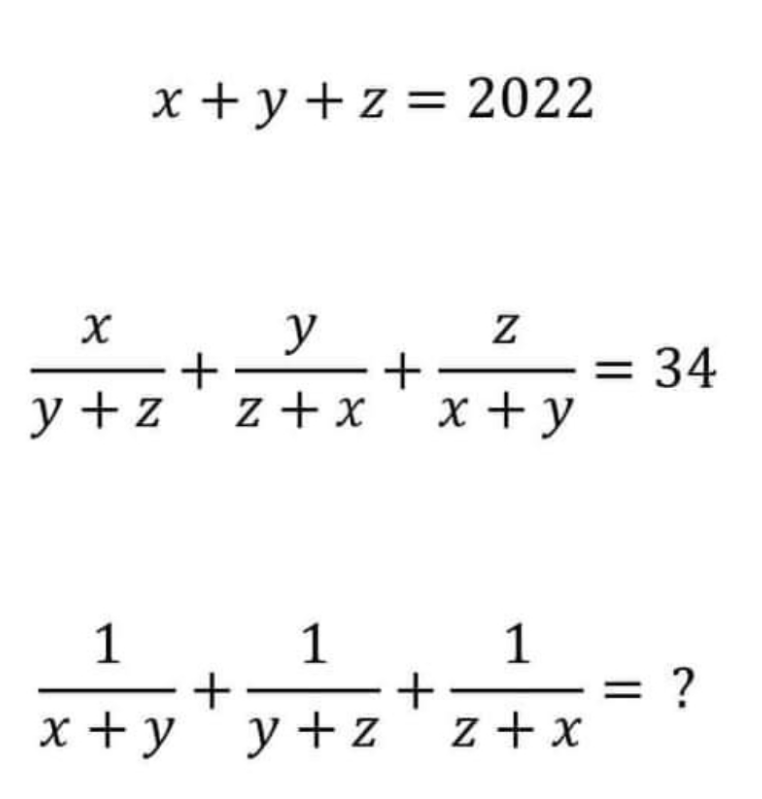


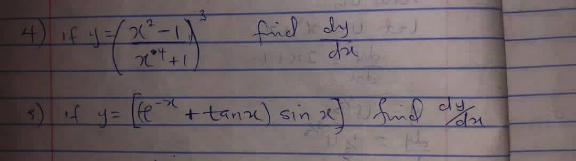

Pg 378 Pg 379 Pg 380 Pg 381 Pg 382 Pg 383 Pg 384 Pg 385 Pg 386 Pg 387
|
Question and Answers Forum |
AllQuestion and Answers: Page 383 |
| 23+2323+232323+....(n terms)=? |
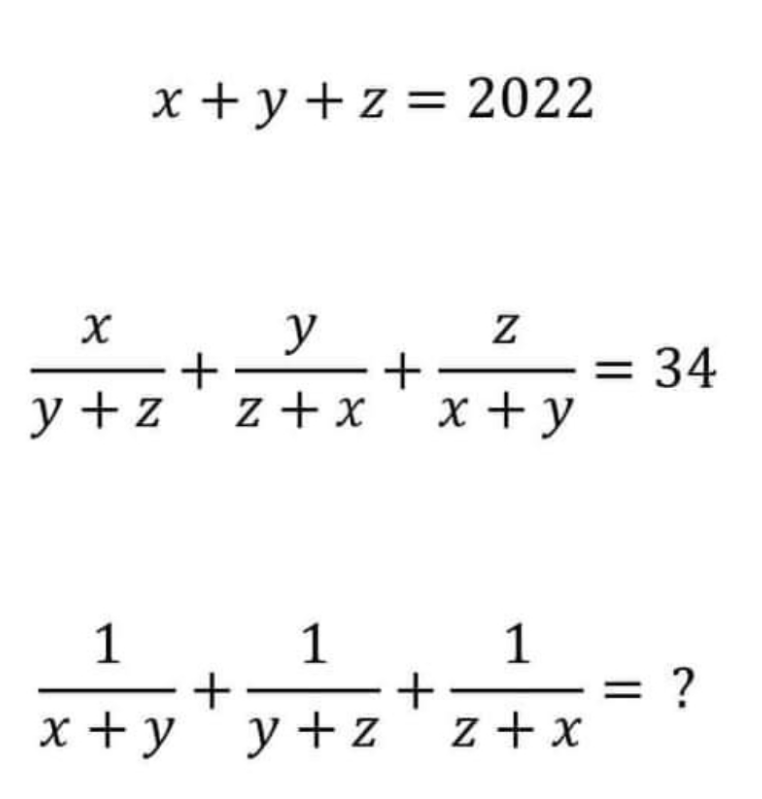
|

|

|

|
| 2^m −2^n = 2016 find m and n |
| solve for x 2^x .3^x^2 = 6 |
| 7+67+667+6667+.....(n terms)=? |
| A=∫9xcos 2x dx |
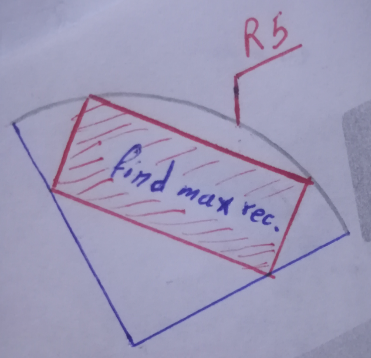
| exercise Consider a polygon with an odd number 𝗻 of vertices. We connect any 3 vertices of this polygon to form a triangle. What is the probability that this triangle contains the center of the circle circumscribing the polygon? |
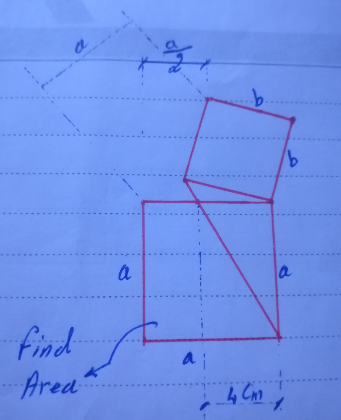
| Trouver la somme de S_n Si S_n =1+11+111+...+1111...111 |
| (d^2 y/dx^2 ) −tan (x)(dy/dx) = 0 |
| solve for x x_(n+1) =rx_n (1−x_n ) |
| if (x+1)^4 (√4)=x+1 find x |

|
| f(4) = (1/4) and f(8) = (1/2) ∫_4 ^8 (( [f′(x)]^2 )/([f(x)]^4 ))dx = 1 then f(6)= ? |
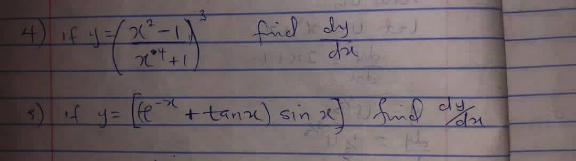
|
| if (x+1)_(√4) ^4 =x+1 find x |
| J=∫_0 ^(π/2) ((sin x)/(1+sin x+cos x)) dx |
| ∫_5 ^7 (([ (1/4)x+3 ])/( (√(9x^2 −12x+4)))) dx=? [ ..] =floor function |

|
| solve for x log_(∣sinx∣ ) (x^2 −8x+23) > (3/(log_2 ∣sinx∣ )) |
| lim_(x→(π/4)) ((sin x−cos x)/(tan ((π/8)−(x/2)))) =? |
| ax^2 +bx+c = 0 x = ((−b±(√(b^2 −4ac)))/(2a)) Example: Find the values of x in the equation x^2 +5x+4 = 0 In order to solve for that, let′s first take a look on what are the values of a, b and c 1x^2 + 5x + 4 = 0 a = 1 ; b = 5 ; c = 4 Now, using the quadratic formula x = ((−5±(√(5^2 −4(1)(4))))/(2(1))) = ((−5±(√(25−16)))/2) = ((−5±3)/2) |
| ∫_0 ^(π/2) ((sin^3 x)/(sin x+cos x)) dx =? |
| lim_(n→∞) (Σ_(j=1) ^n Σ_(i = 1) ^n ((i+j)/(i^2 +j^2 ))−((π/2)+ ln 2)n + ln n) = ? |
Pg 378 Pg 379 Pg 380 Pg 381 Pg 382 Pg 383 Pg 384 Pg 385 Pg 386 Pg 387 |