
Question and Answers Forum
AllQuestion and Answers: Page 388








Pg 383 Pg 384 Pg 385 Pg 386 Pg 387 Pg 388 Pg 389 Pg 390 Pg 391 Pg 392
|
Question and Answers Forum |
AllQuestion and Answers: Page 388 |
| hello, please, someone help me to correct the equation? It's typed wrong and I can't find where Solve: 5,76[((log_a ((√(log _b ((√a))))))/(log((√(log(a)))))) + log_(log (a)) (2)]((log _2 (x)))^(1/5) + ((log_2 (x))/(25)) = [log _2 (x)]^(3/5) Answers x_1 =1 , x_2 =2^(243) , x_3 =2^(−243) , x_4 =2^(1024) , x_5 =2^(−1024) |

|

|

|
| prove: Use the residus form ∫_0 ^(+∞) ((xsinx)/((x^2 +1)^2 ))dx=(π/(4e)) |
| A large lot of tires contain 5% defectives. 4 tires are to be chosen for a car. Find the probability that you find (a) 2 defective tires before 4 good ones (b) at most 2 defective tires before 4 good ones. |
| A ball of p of mass 0.25kg losses (1/3) of its velocity when it makes an head on collision with an identical ball q at rest. After collision, q moves off with a velocity of 2ms^(−1) in the original direction of p. Calculate the initial velocity of p. |
| Find The Centroid Coordinate If Trapezoid With B_1 =4, B_2 =3 And Height=5 With Positions Like This |
| 𝚺_(n=0) ^∞ (((−1)^n )/(7+6n))[𝛙^((0)) (((9+6n)/2))−𝛙^((0)) (((7+6n)/2))] |
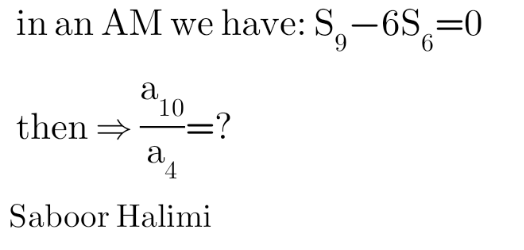
|

|

|

|

|

|

|
| ∫ ((x+sin x)/(1+cos x)) dx =? |

|
| solve for all x ⌊x^2 ⌋ − ⌊x⌋^2 = 100 |
| lim_(n→∞) (1/n)[1+(√2)+^3 (√3)+...^n (√n)] |
| Have you seen this method of solving quadratic problem? x^2 −x−12=0 y′=±(√(b^2 −4ac)) |
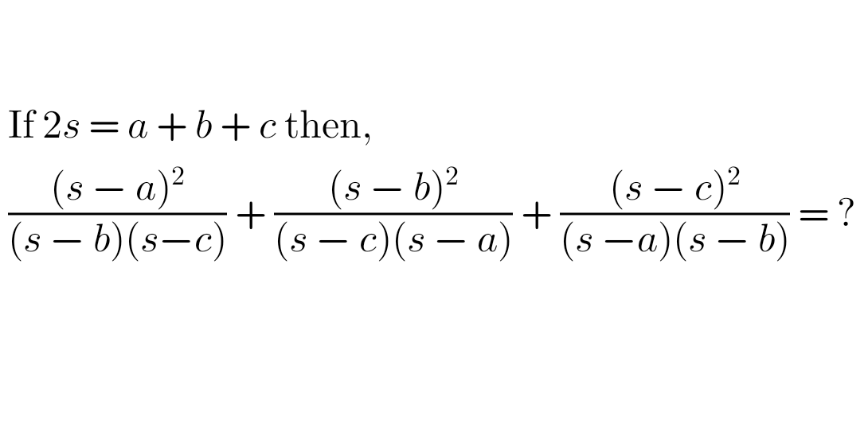
|
| How many digits does 1000^(1000) have? Mastermind |
| Find the value of a for which the limit lim_(x→0) ((sin (ax)−arctan x−x)/(x^3 +x^4 )) is finite and then evaluate the limit |
| sum of the all proper factors of 360 ? |
| let u_n = ∫_0 ^1 x^n artan(nx)dx 1)lim u_n ? 2)nature of Σ u_n 3) calculate u_n 4)equivalent of u_n ? |
Pg 383 Pg 384 Pg 385 Pg 386 Pg 387 Pg 388 Pg 389 Pg 390 Pg 391 Pg 392 |