
Question and Answers Forum
AllQuestion and Answers: Page 390








Pg 385 Pg 386 Pg 387 Pg 388 Pg 389 Pg 390 Pg 391 Pg 392 Pg 393 Pg 394
|
Question and Answers Forum |
AllQuestion and Answers: Page 390 |
|

|
| ≪_• ^• I THINK...^ ^(−) _•^• _(−) ≫ One question per post, IDEAL 👍👍👍 Two questions per post,OK (BEARABLE) (👎+👍)/2 Three or more questions:NO, NO, NO! 👎👎👎 |
| A graduating student keeps applying for a job until she gets an offer. The probability of getting an offer at any trial is 0.35. What is the expected number of applications? |

|
|

|
| ∫_0 ^∞ (e^(−x^2 ) /((x^2 +a^2 )^2 ))dx |

|
| lim_(x→∞) cos((π/4))cos((π/8)) ... cos((π/2^(n+1) )) |
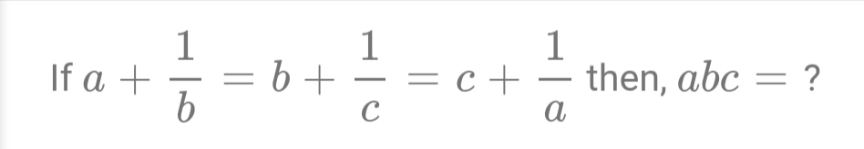
|

|
| prove that : 𝛀 = ∫_0 ^( ∞) ( (( x)/( sinh (x))) )^( 3) dx =(𝛑^( 2) /(16)) (12− 𝛑^( 2) ) written and prepared by : m.n |

|
| prove that : Ω = ∫_0 ^( ∞) (( x^( 2) )/(cosh(x ))) dx = (π^( 3) /( 8)) |
| lim_(x→0) [(x^2 /(sinx tanx ))] [∙] greatest integer function |
| Q: I , J are two ideals of commutative ring , ( R ,⊕, ) .prove that : (√( I ∩ J )) =^? (√( I )) ∩ (√( J )) m.n note : (√(I )) = { x ∈ R ∣ ∃ n∈ N , x^( n) ∈ I } |
| In a game of lotto, balls are numbered 1 through to 90. They are placed in a barrel and five balls are drawn out without replacement. The balls are of the same size and are equally like to be drawn. The first five balls drawn out are 28 - 06 - 27 - 54 - 86. What is the probability that the next ball drawn out will be number 18? |

|
| In △ABC the following relationship holds: a^4 < (b^2 + c^2 )^2 + 9R^4 |
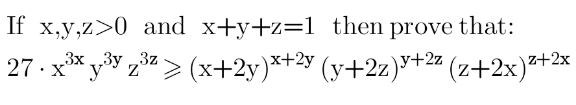
|
| for what values a and b is the function differentiable at x=2? 4x−2x^(2 ) x≤2 ax^(3 ) +bx,x>2 |

|
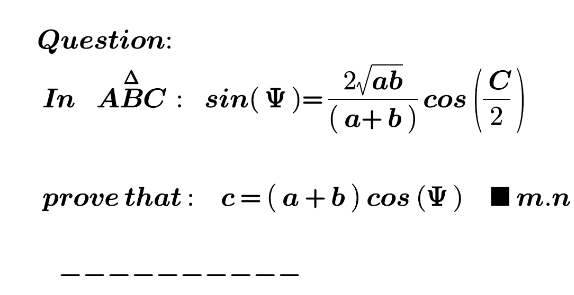
|
| find the domain and range of tbe following 1) y = cot(2x+1) 2) y = sin∣x∣ 3) ∣y∣ = cosx 4) y = cos(cosx) 5) y =∣cscx∣ −1 6) y = sin(sinx) |

|
Pg 385 Pg 386 Pg 387 Pg 388 Pg 389 Pg 390 Pg 391 Pg 392 Pg 393 Pg 394 |