
Question and Answers Forum
AllQuestion and Answers: Page 472



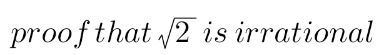
Pg 467 Pg 468 Pg 469 Pg 470 Pg 471 Pg 472 Pg 473 Pg 474 Pg 475 Pg 476
|
Question and Answers Forum |
AllQuestion and Answers: Page 472 |
| calculate :: lim_(t→∞) 8∫_0 ^(π/2) e^x ∙sin (tx)∙sin (2tx)∙cos (3tx)∙cos (4tx)dx=? |
| calculate :: lim_(x→(√π)) ∫_(√π) ^x (((x^2 +(√π)t)e^t^2 )/((x−(√π))(x^2 +π)t^2 lnt))dt=? |
| Σ_(n=2) ^(n=∞) ((3/4))^n cos (180°n)= ? |
| 1+1≠?? |
| hi! help me! lim_(x→∞) ∫_0 ^∞ e^2 c(√x)∫_0 ^∞ =? |
| find the maximum of f(x)=sin x+cos x+sin x cos x |
| hi ! help me ! lim_(x→−∞) (e^(1/(x+(√(x^2 +1)))) /x) = ??? |
| γ=∫ ((e^x (sin x+1))/(cos x+1)) dx =? |
| If polynomial x^3 −9x^2 +11x−1=0 have the roots are a,b an c . Given Δ = (√a) + (√b) + (√c) then Δ^4 −18Δ^2 −8Δ =? |
| calculate ∫_0 ^( ∞) (((√x) arctan(x))/(1+x^( 2) ))dx =? |
| Given x^3 −3x^2 (√2) +6x−2(√2)−8=0 then x^5 −41x^2 +2022 =? |

|

|

|
|
| A particle of mass 0.25kg vibrates with a period of 2secs. If its greatest displacement is 0.4m. What is its maximum kinetic energy. |

|
| lim_(n→∞) (5^n /(n!))=? |

|
| Ω= Σ_(n=1) ^∞ (( H_( n) )/(n(n+1))) = −−−−−− Ω = Σ_(n=1) ^∞ −(1/(n+1)) ∫_(0 ) ^( 1) x^( n−1) ln(1−x )dx = ∫_0 ^( 1) {−(1/x^2 )ln(1−x).Σ_(n=1) (x^( n+1) /(n+1))}dx = ∫_0 ^( 1) {((−ln(1−x))/x^( 2) )Σ_(n=2) ^∞ (x^( n) /n)}dx = ∫_0 ^( 1) ((−ln(1−x))/x^( 2) ) {−x +Σ_(n=1) ^∞ (x^( n) /n) }dx = −li_( 2) ( 1) +[ ∫_0 ^( 1) ((ln^( 2) ( 1−x ))/x^( 2) )dx=_(derived) ^(earlier) (π^( 2) /3) ] = −(π^( 2) /6) + (π^( 2) /3) = (( π^( 2) )/6) = ζ (2) ■ m.n |
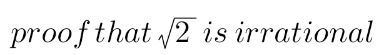
|
| given that is prime,proof that (√p) is irrational |
| A pendulum has a period 2sec. The bob pulled aside a distance 8cm as the amplitude and release. Find the displacement of the bob 0.7sec after it has been released. |
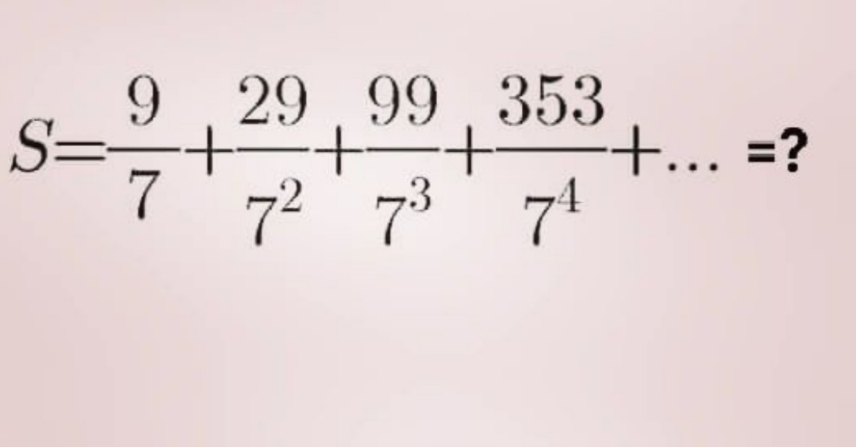
|
| calculate : lim_(x→1) (((1+x)^(1/x) (1+(1/x))^x −4)/((x−1)^2 ))=ln16−3 |
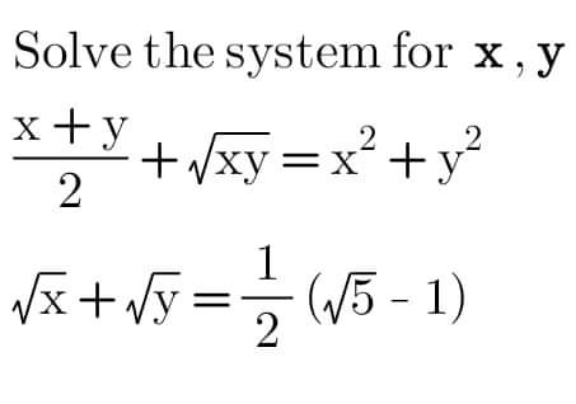
|
Pg 467 Pg 468 Pg 469 Pg 470 Pg 471 Pg 472 Pg 473 Pg 474 Pg 475 Pg 476 |