Question and Answers Forum

AllQuestion and Answers: Page 487

Question Number 165240 Answers: 1 Comments: 0

soit la serie de fonction Σ_(n=2 ) (x^n /(nx+ln(n))) etudie la convergence simple sur [0,1[

$s o i t l a s e r i e d e f o n c t i o n \sum_{n = 2} \frac{x^{n}}{n x + l n (n)}$ $e t u d i e l a c o n v e r g e n c e s i m p l e s u r [0, 1 [$

Question Number 165234 Answers: 0 Comments: 1

Question Number 165253 Answers: 3 Comments: 0

Question Number 165232 Answers: 1 Comments: 1

Question Number 165250 Answers: 0 Comments: 1

Question Number 165229 Answers: 0 Comments: 1

Σ_(i=1) ^(n=∞) lim_(x→0) ((xyn^x )/(nx_1 ))xz∫((xn^n x)/(nx^n y))dn (√(c^n +n_1 )) ∫((xn^(n!) )/(n^n xn^x ))dn

$\underset{i = 1}{\sum^{n = \infty}} \lim_{x \to 0} \frac{{x y n}^{x}}{{n x}_{1}} x z \int \frac{{x n}^{n} x}{{n x}^{n} y} d n$ $\sqrt{c^{n} + n_{1}}$ $\int \frac{{x n}^{n!}}{n^{n} {x n}^{x}} d n$

Question Number 165226 Answers: 1 Comments: 0

make x the subject of the formula; a^x +bx+c=0

$m a k e x t h e s u b j e c t o f t h e f o r m u l a;$ $a^{x} + b x + c = 0$

Question Number 165217 Answers: 2 Comments: 5

Question Number 165218 Answers: 1 Comments: 1

Question by M.N July Φ = ∫_0 ^( 1) ((ln(1 + x^4 + x^8 ))/x)dx Φ =^(x=x^(1/2) ) (1/2)∫_0 ^( 1) ((ln(1+x^2 +x^4 ))/x)dx Φ = (1/2)∫_0 ^( 1) ((ln(((1−x^2 )/(1−x^6 ))))/x)dx = (1/2)∫_0 ^( 1) ((ln(1−x^2 ))/x)dx − (1/2)∫_0 ^( 1) ((ln(1−x^6 ))/x)dx Φ = (1/2)(A − B) A =^(x=x^(1/2) ) (1/2)∫_0 ^( 1) ((ln(1−x))/x)dx = (1/2)Li_2 (1) B =^(x=x^(1/6) ) (1/6)∫_0 ^( 1) ((x^((1/6)−1) ln(1−x))/x^(1/6) )dx B = (1/6)∫_0 ^( 1) ((ln(1−x))/x)dx = (1/6)Li_2 (1) Φ = (1/2)((1/2)Li_2 (1)−(1/6)Li_2 (1)) = (1/6)Li_2 (1) 𝚽 = ((𝛇(2))/3) ▲▲▲

Question Number 165215 Answers: 1 Comments: 0

Find: Σ_(n=1) ^∞ tan^(−1) ((1/n^2 ))

$Find : \underset{n = 1}{\sum^{\infty}} \tan^{- 1} (\frac{1}{n^{2}})$

Question Number 165210 Answers: 0 Comments: 1

Σ_(n=4) ^∞ 2 × ((3/4))^(n+3)

$\underset{n = 4}{\sum^{\infty}} 2 \times {(\frac{3}{4})}^{n + 3}$

Question Number 165209 Answers: 0 Comments: 0

Find: Σ_(n=1) ^k ((n^2 sin^2 ((nπ)/2))/(n^4 π^4 + n^2 π^2 a + b)) = ?

$Find : \underset{n = 1}{\sum^{k}} \frac{n^{2} \sin^{2} \frac{n π}{2}}{n^{4} π^{4} + n^{2} π^{2} a + b} = ?$

Question Number 165208 Answers: 1 Comments: 0

lim_(x→1_(y→2) ) x^2 +y^2 =?

$\lim_{x \to \underset{y \to 2}{1}} x^{2} + y^{2} = ?$

Question Number 165194 Answers: 2 Comments: 0

∫_0 ^( 2π) ln ( 1+ cos (x)).cos (nx )dx=?

$\int_{0}^{2 π} l n (1 + c o s (x)) . c o s (n x) d x = ?$

Question Number 165183 Answers: 0 Comments: 1

Question Number 165178 Answers: 2 Comments: 0

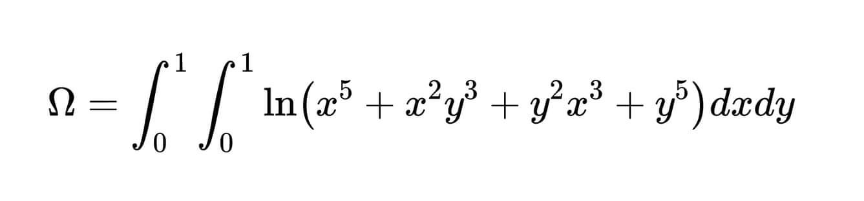
x∈R ⇒ ∣ log _2 ((x/2))∣^3 +∣log _2 (2x)∣^3 =28

$x \in R \Rightarrow ∣ \log_{2} (\frac{x}{2}) ∣^{3} + ∣ \log_{2} (2 x) ∣^{3} = 28$

Question Number 165170 Answers: 2 Comments: 3

Question Number 165168 Answers: 1 Comments: 0

Question Number 165164 Answers: 1 Comments: 0

Question Number 165162 Answers: 0 Comments: 0

f(x)=2x^2 +5x. Montrer que f est lipschitzienne sur R.

$f (x) = 2 x^{2} + 5 x .$ $M o n t r e r q u e f e s t l i p s c h i t z i e n n e$ $s u r R .$

Question Number 165161 Answers: 0 Comments: 0

f(x)=2x^2 +5x. Montrez que f est uniformement continue sur R.

$f (x) = 2 x^{2} + 5 x .$ $M o n t r e z q u e f e s t u n i f o r m e m e n t c o n t i n u e$ $s u r R .$

Question Number 165160 Answers: 1 Comments: 0

f(x+f(x))=3f(x) and f(−1)=7 faind f(27)=?

$f (x + f (x)) = 3 f (x) a n d f (- 1) = 7$ $f a i n d f (27) = ?$

Question Number 165150 Answers: 1 Comments: 1

Question Number 165142 Answers: 0 Comments: 2

Question Number 165136 Answers: 3 Comments: 0

Question Number 165271 Answers: 1 Comments: 0

Let , f : [ 0 , 1 ] → R is a continuous function , prove that : lim_( n→ ∞) ∫_0 ^( 1) (( n f(x))/(1+ n^2 x^( 2) )) dx = (π/2) f (0 ) −−− proof −−− S_( n) = [∫_(0 ) ^( (1/( (√n)))) (( n. f(x))/(1 + n^( 2) x^( 2) )) dx =Ω_( n) ]+[ ∫_(1/( (√n))) ^( 1) ((n.f (x))/(1 + n^( 2) x^( 2) )) dx = Φ_( n) ] Ω_( n) =_(∃ t_( n) ∈ ( 0 , (1/( (√n) )) )) ^(MeanValueTheorem( first)) f (t_( n) )∫_(0 ) ^( (1/( (√n)))) (( n)/(1 + n^( 2) x^( 2) ))dx = f ( t_( n) ) ( tan^( −1) ( (√n) )) lim_( n→∞) (Ω_( n) ) = (π/2) f (lim_( n→∞) ( t_( n) ) ) = (π/2) f (0 ) Φ_( n) = ∫_(1/( (√n))) ^( 1) (( n. f(x) )/(1 + n^( 2) x^( 2) )) dx ⇒_(∃ M >0) ^(f is bounded) ∣ Φ_( n) ∣ ≤ M.∫_(1/( (√n))) ^( 1) (n/(1+ n^( 2) x^( 2) )) dx ⇒ ∣ Φ_( n) ∣ ≤ M . ( tan^( −1) ( n )− tan^( −1) ( (√n) )) lim_( n→ ∞) ∣ Φ_( n) ∣ = 0 ⇒ lim_( n→∞) Φ_( n) =0 ∴ lim_( n→ ∞) ( S_( n) ) = (π/2) f (0 ) ■ m.n

$L e t, f : [0, 1] \to R i s a c o n t i n u o u s$ $f u n c t i o n, p r o v e t h a t :$ ${l i m}_{n \to \infty} \int_{0}^{1} \frac{n f (x)}{1 + n^{2} x^{2}} d x = \frac{π}{2} f (0)$ $- - - p r o o f - - -$ $S_{n} = [\int_{0}^{\frac{1}{\sqrt{n}}} \frac{n . f (x)}{1 + n^{2} x^{2}} d x = Ω_{n}] + [\int_{\frac{1}{\sqrt{n}}}^{1} \frac{n . f (x)}{1 + n^{2} x^{2}} d x = Φ_{n}]$ $Ω_{n} \underset{\exists t_{n} \in (0, \frac{1}{\sqrt{n}})}{\overset{M e a n V a l u e T h e o r e m (f i r s t)}{=}} f (t_{n}) \int_{0}^{\frac{1}{\sqrt{n}}} \frac{n}{1 + n^{2} x^{2}} d x$ $= f (t_{n}) ({t a n}^{- 1} (\sqrt{n}))$ ${l i m}_{n \to \infty} (Ω_{n}) = \frac{π}{2} f ({l i m}_{n \to \infty} (t_{n})) = \frac{π}{2} f (0)$ $Φ_{n} = \int_{\frac{1}{\sqrt{n}}}^{1} \frac{n . f (x)}{1 + n^{2} x^{2}} d x \underset{\exists M > 0}{\overset{f i s b o u n d e d}{\Rightarrow}} ∣ Φ_{n} ∣ ⩽ M . \int_{\frac{1}{\sqrt{n}}}^{1} \frac{n}{1 + n^{2} x^{2}} d x$ $\Rightarrow ∣ Φ_{n} ∣ ⩽ M . ({t a n}^{- 1} (n) - {t a n}^{- 1} (\sqrt{n}))$ ${l i m}_{n \to \infty} ∣ Φ_{n} ∣ = 0 \Rightarrow {l i m}_{n \to \infty} Φ_{n} = 0$ $∴ {l i m}_{n \to \infty} (S_{n}) = \frac{π}{2} f (0) ◼ m . n$

Pg 482 Pg 483 Pg 484 Pg 485 Pg 486 Pg 487 Pg 488 Pg 489 Pg 490 Pg 491

Contact: info@tinkutara.com