
Question and Answers Forum
AllQuestion and Answers: Page 532
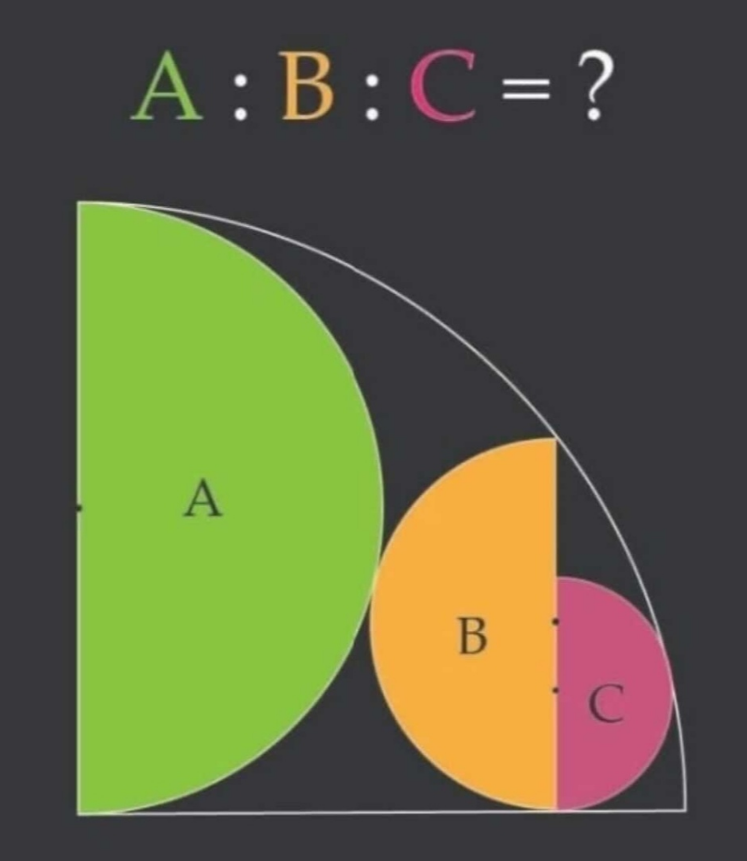
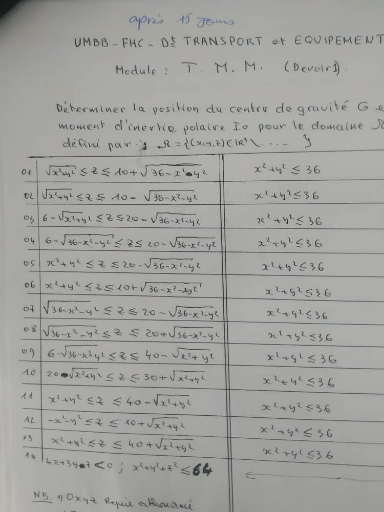




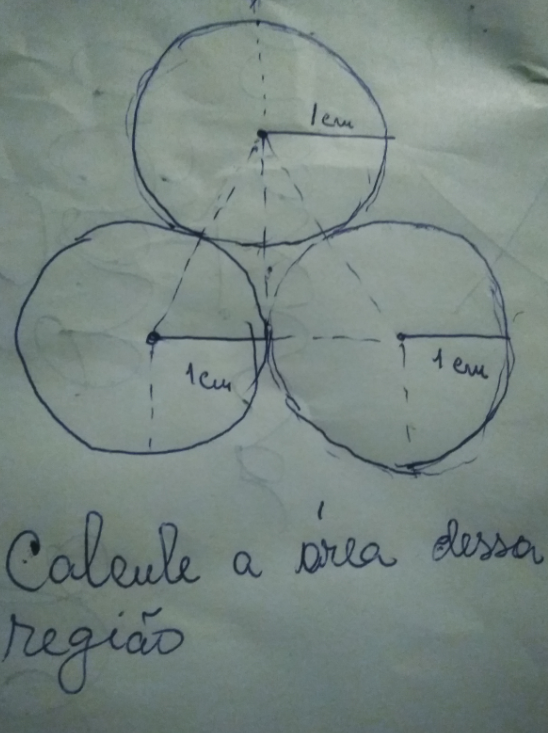

Pg 527 Pg 528 Pg 529 Pg 530 Pg 531 Pg 532 Pg 533 Pg 534 Pg 535 Pg 536
|
Question and Answers Forum |
AllQuestion and Answers: Page 532 |
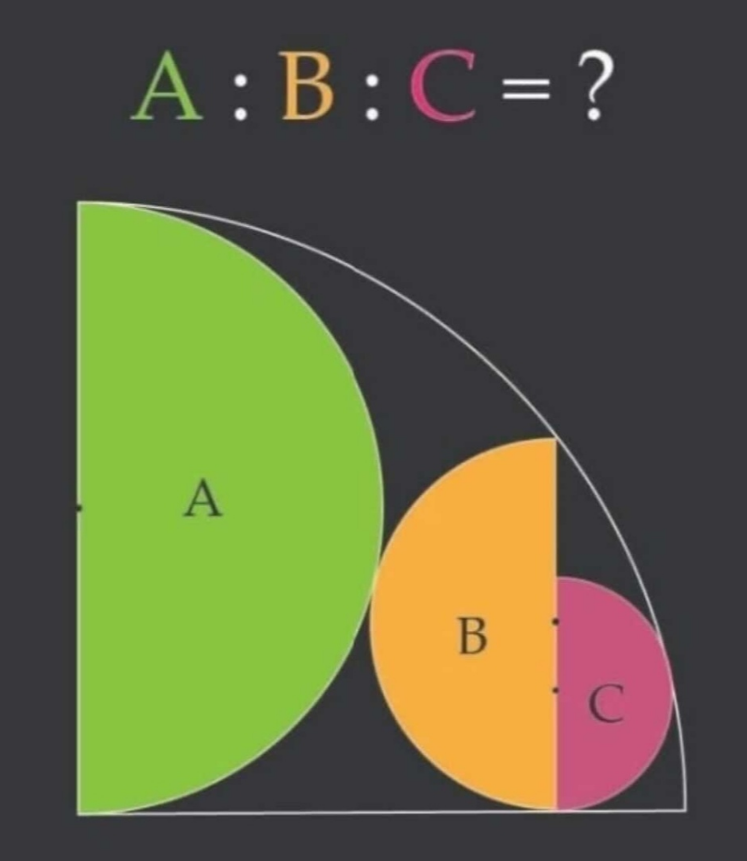
|

|
| lim_(x→π) (((tan x)/(1+cos x)))=? |
| Evaluate (d^2 y/dx^2 ) when x=ln 2 and y=2. |
| Given that y=(3 sin x−4 cos x+6)^2 , 0≤x≤2π. Find the smallest value of y. |
| S_n = ((12)/((4^2 −3^2 )(4^2 −3^2 )))+((12^2 )/((4^2 −3^2 )(4^3 −3^3 )))+((12^3 )/((4^2 −3^2 )(4^4 −3^4 )))+...+((12^n )/((4^2 −3^2 )(4^(n+1) −3^(n+1) ))) lim_(n→∞) S_n = ? |
| (x+(√(x^2 +1)))(y+(√(y^2 +1)))=2021 ∀x,y∈R^+ . min (x+y)=? |
| ∫_0 ^( (π/2)) (dx/(2−cos x)) =? |

|
| ∫ (dx/(1−tan^2 (x))) =? |
| ∫ ((lnz)/(z+lnz)) dz help me sir |

|
| Ω:=∫_0 ^( (1/2)) (( arcsinh(x))/x) dx =^? (π^2 /(20)) |

|
| Ω=∫_0 ^( 1) tan^( −1) (x).ln(x) = ? −−−−solution−−−− f(a)=∫_0 ^( 1) tan^( −1) ( x) .x^( a) dx = Σ_(n=1) ^∞ (( (−1)^( n−1) )/(2n−1)) ∫_0 ^( 1) x^( 2n+a−1) dx = Σ_(n=0) ^∞ (((−1)^( n−1) )/(2n−1)) ((1/(2n+ a)) ) Ω= f ′ (a )∣_(a=0) = Σ_(n=1) ^∞ (((−1)^n )/((2n−1)( 2n+ a)^( 2) )) Ω= f ′ (0 )=(1/4)Σ(( (−1 )^(n−1) (2n−1−2n ) )/(( 2n−1)n^( 2) )) =(1/4) Σ_(n=1) ^∞ (((−1)^(n−1) )/n^( 2) ) − Σ_(n=1) ^∞ (((−1)^( n−1) )/((2n−1)(2n))) = (π^( 2) /(48)) −{ Σ_(n=1) ^∞ {(((−1)^(n−1) )/(2n−1)) −(((−1)^( n−1) )/(2n))}} ∴ Ω = (π^( 2) /(48)) − (π/4) +(1/2) ln(2) |

|
| Calculate 1. lim_(x→+∞) ((x(√(ln (x^2 +1))))/(1+e^(x−3) )) 2. lim_(x→+∞) (((x^3 +5)/(x^2 +2)))^((x+1)/(x^2 +1)) |
| Calculate 1. lim_(x→0) [2e^(x/(x+1)) −1]^((x^2 +1)/x) 2. lim_(x→0) (((1+x×2^x )/(1+x×3^x )))^(1/x^2 ) |
| if the roots of the equation ax^2 +bx+c=0 are in the ratio 3:4,then show that 12b^2 =49ac. |
| ∫x{x}[x]dx=? |

|
| Find: 𝛀 =∫_( 1) ^( ∞) ((ln(x))/(x^4 + x^2 + 1)) dx = ? |
| solve ⌊ x− (√(1−x^( 2) )) ⌋+⌊ x+ (√(1−x^( 2) )) ⌋=0 |
| ∫_0 ^∞ ((arctg(x))/(1+x))∙(dx/( (x)^(1/4) ))=? |

|
Pg 527 Pg 528 Pg 529 Pg 530 Pg 531 Pg 532 Pg 533 Pg 534 Pg 535 Pg 536 |