
Question and Answers Forum
AllQuestion and Answers: Page 574
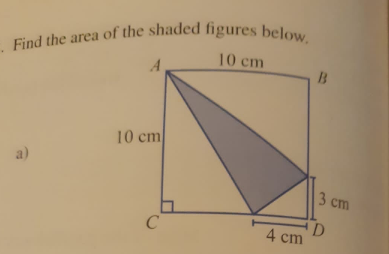





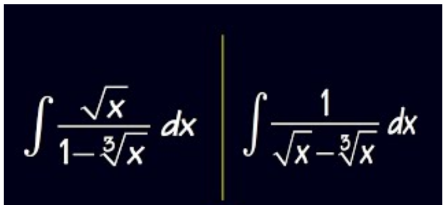




Pg 569 Pg 570 Pg 571 Pg 572 Pg 573 Pg 574 Pg 575 Pg 576 Pg 577 Pg 578
|
Question and Answers Forum |
AllQuestion and Answers: Page 574 |
| If abc^(−) = cba^(−) + def^(−) and a∈{c+2;...;9} Then find def^(−) + fed^(−) |
| Solve for real numbers: 3 + sin(2x) = 4sin(x + (π/4)) |
| if a;b;c;d>1 then: Σ_(cyc) log_a (((b^3 + c^3 + d^3 )/(b^2 + c^2 + d^2 ))) ≥ 4 |

|

|

|
| Solve for real numbers: 9 + log_2 (x/(x^4 + 48)) = 2 + (2/( (√(x - 1)))) |
| Find x;y;z≥0 such that: { ((x-y-z = sinx-siny-sinz)),((x^2 -y^2 -z^2 = sin^2 x-sin^2 y-sin^2 z)),((x^3 -y^3 -z^3 = sin^3 x-sin^3 y-sin^3 z)) :} |

|
| If 0<a≤b<π then prove that: ((sin(√(ab)))/(sin(((a+b)/2)))) ≥ ((32a^2 b^2 (√(ab)))/((a+b)^5 )) |
| Find all functions f : Z → R such that f(n+m)=nf(n)+mf(m)+nm-n-m ∀n;m∈Z |

|
| in a second order differenti equation, a 4 henry inductor,an 8 ohm resistor and o.2 farad capacito are connected in series with the temperature of the battery with ggl. E=80 sin 3t. solid=0 the charge in the capacitor and the current in the circuit is zero. a.charge b.current at t>0 |
| Given that tan α and tan β are the roots of the equation x^2 +3ax+4a+1=0, where a>1 and α,β∈(−(π/2),(π/2)). Evaluate tan(((α+β)/2)). |

|
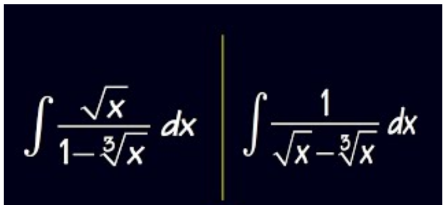
|

|
| x is positive integer number can you check if Q=((((x+2)^4 −x^4 ))^(1/3) is a natural number |

|
| x^4 +bx^2 +cx+d=0 let cx=m+px^2 +x^4 ⇒ 2x^4 +(b+p)x^2 +m+d=0 x^2 =−(((b+p)/4))±(√((((b+p)/4))^2 −(((m+d)/2)))) (p−b)x^2 −2cx+m−d=0 x=(c/(p−b))±(√(((c/(p−b)))^2 −(((m−d)/(p−b))))) ⇒ (((b^2 −p^2 ))/4)±(√(((b^2 −p^2 )^2 −8(p−b)^2 (m+d))/(16))) +((2c^2 )/(b−p))∓(√((4c^4 −4c^2 (p−b)(m−d))/((p−b)^2 ))) +m−d=0 Just if m=d ⇒ (((b^2 −p^2 ))/4)±(√(((b^2 −p^2 )^2 −16d(p−b))/(16))) +((4c^2 )/(b−p))=0 ⇒ (((b^2 −p^2 )/4)+((4c^2 )/(b−p)))^2 +d(p−b)=(((b^2 −p^2 )^2 )/(16)) ⇒ ((16c^4 )/((b−p)^2 ))+(d+2c^2 )(b+p)=0 let b−p=z z^2 (2b−z)=−((16c^4 )/(2c^2 +d)) z^3 −2bz^2 −k=0 (k>0) if b=−1 , d=0, c→−c z^3 +2z^2 −8c^2 =0 let z=((2c)/t) 8c^3 +8c^2 t−8c^2 t^3 =0 ⇒ t^3 −t=c back to square one. |
| find two numbers between (1/3)and (3/4) |

|

|
| ∫_0 ^( (𝛑/2)) (x^2 /(sin(x))) dx |
| φ (n )= Σ_(k=1) ^n (−1 )^( k−1) ((( n)),(( k)) ) H_( k) Find the value of : Σ_(n=1) ^∞ (−1)^( n−1) φ ( n^( 2) ) =? |
| A. lim_(x→+∞) Σ_(k=1) ^n [tan((kπ)/(2n))] |
Pg 569 Pg 570 Pg 571 Pg 572 Pg 573 Pg 574 Pg 575 Pg 576 Pg 577 Pg 578 |