
Question and Answers Forum
AllQuestion and Answers: Page 587









Pg 582 Pg 583 Pg 584 Pg 585 Pg 586 Pg 587 Pg 588 Pg 589 Pg 590 Pg 591
|
Question and Answers Forum |
AllQuestion and Answers: Page 587 |

|
| ∫((x+sinx)/(1+cosx))dx please,help me |
| Ω :=∫_0 ^( 1) (( x.sin(ln(x)))/(1−x))dx method 1 Ω= Im[∫_0 ^( 1) (( x^( i+1) )/(1−x)) dx=Φ] Φ = ∫_0 ^( 1) (( x^( i+1) +x^( i+2) )/(1−x^( 2) ))dx =^(x^( 2) =t) (1/2)∫_0 ^( 1) (( t^( (i/2)) −t^((i+1)/2) )/(1−t))dt = (1/2) { ψ (1 +((i+1)/2))−ψ (1+(i/2))} = (1/2) { (2/(1+i)) + ψ ( ((1+i)/2) )−(2/i)−ψ ((i/2))} = ((−i)/(−1+i)) + (1/2) {ψ (((1+i)/2))−ψ((i/2) )} = −(1/2) +(i/(2 )) +(1/2) {ψ(((1+i)/2))−ψ((i/2))} Ω = Im (Φ )= (1/2) +(1/2) Im(ψ((1/2) +(i/2)))−(1/2) Im((i/2)) = (1/(2 )) { 1 +(π/2) tanh((π/2)) −1−(π/2) coth((π/2))} =(π/4) {((−1)/(sinh((π/2)).cosh((π/2))))}=((−π)/(2 sinh (π ))) ✓ |
|

|
|

|
| z^4 - ((50)/(2z^4 - 7)) = 14 ⇒ z = ? |

|

|

|
| Given f(x)=((x+(√(1+x^2 ))))^(1/3) +((x−(√(1+x^2 ))))^(1/3) Find f^(−1) (x)=? |
| A long distance runner runs 14km 30°north of east, 7km 60° north of west, 6km 30° south of west, and finally 4km south. Find his final distance and direction relative to the starting point? |
| g(((x−1)/(x+1)))=((7x+3)/(x+1)) and f(x^2 −2x+3)=3x^2 −6x+7 find (f+g)(x)=? |

|
| ∫ e^(√x) dx =? |
| si w est une racine cubique de 1 different de 1,alors: (1+w−w^2 )^7 =? |

|
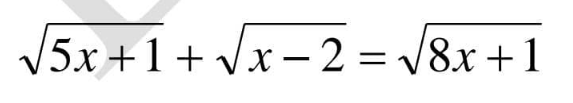
|
| { ((x^2 +y(√(xy)) = 72)),((y^2 +x(√(xy)) = 36)) :} |

|

|

|
| lim_(x→∞) ((32x^5 −14x^4 +3))^(1/5) −((128x^7 +6x^6 −1))^(1/7) =? |
| Ω =∫_0 ^( (π/2)) ln^2 (((1+sin t)/(1−sin t)))dt |

|
Pg 582 Pg 583 Pg 584 Pg 585 Pg 586 Pg 587 Pg 588 Pg 589 Pg 590 Pg 591 |