
Question and Answers Forum
AllQuestion and Answers: Page 606





Pg 601 Pg 602 Pg 603 Pg 604 Pg 605 Pg 606 Pg 607 Pg 608 Pg 609 Pg 610
|
Question and Answers Forum |
AllQuestion and Answers: Page 606 |
| An electric current passes through two voltmeters in series containing copper sulphate (CuSO₄) and silver nitrate (AgNO₃) respectively. What is the mass of silver deposited in a given time, if the mass of copper deposited in that time is 1g. (Cu = 63 , Ag = 108 valency of Cu is 2 and valency of Ag is 1). |
| Find the coefficient of 1.x^(20 ) in (1−x+x^2 )^(20) 2.x^4 in (1+x+x^2 +x^3 )_ ^(11) please,help me |

|
| resouds ∣1−x∣y′+xy=x |
| Given that f○g = (x^2 /(2x^2 − x + 4)) and g(x) = (x/(x − 2)), find f(x). |
| 𝛗=∫_0 ^( ∞) ((sin(x ))/x)(((a^( 2) +cos^( 2) (x))/(b^( 2) + cos^( 2) (x ))))dx=? |

|
| Solve .......... Ω := ∫_0 ^( 1) x. sin( ln (x ))dx =^? ((−1)/( 5)) solution.... Ω :=^(i.b.p) [ (x^( 2) /2) . sin(ln(x))]_0 ^( 1) −(1/2)∫_0 ^( 1) x.cos( ln (x ))dx := ((−1)/2) ∫_0 ^( 1) x. cos (ln (x ))dx :=^(i.b.p) ((−1)/2) {[ (x^( 2) /2) cos (ln(x ))]_0 ^1 +(1/2) ∫x. sin(ln(x ))dx} := ((−1)/4) −(1/4) Ω (5/4) Ω = ((−1)/4) ⇒ Ω := ((−1)/( 5)) .........■ m.n ................................. |
| if ((((x−2))^(1/3) +2))^(1/3) + ((2−((x−2))^(1/3) ))^(1/3) =2 then find the value of (√(198x^4 −868x^3 −229x^2 +200x)) |
| If f(z) = z sin(z) + ∣z∣^2 , verify if f(z) satisfy cauchy rieman condition |
| How to prove that a<b<c ⇒ a+b > c which a,b,c are sides of a triangle ? |
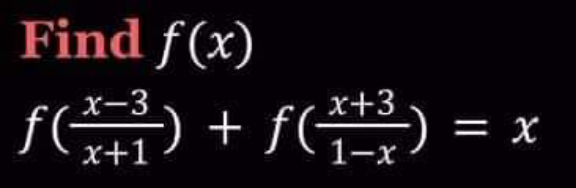
|
| lim_(x→∞) (((5x+1)/(−5x+2)))^(1+2x) =? |
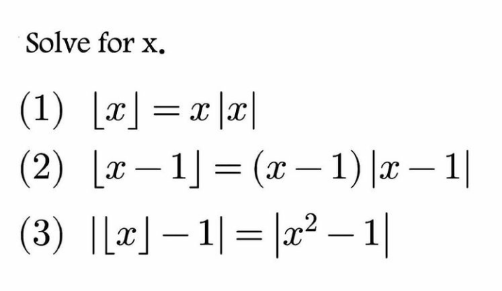
|
| prove that: S := Σ_(n=1) ^∞ ((( 1)/(sinh (2^( n) .x)))) =^? (( 2)/(e^( 2x) −1)) m.n... |
| Find all complex number z such that (3z+1)(4z+1)(6z+1)(12z+1)=2 |
| ∫!dx i found this question somewhere and i dont know even know how to approach it. |
| Determine all triplets (a;b;c) of positive integers which satisfy: (1/a) + (1/b) + (1/c) = (1/2) |
| Given ((((x−2))^(1/3) +2))^(1/3) +((2−((x+2))^(1/3) ))^(1/3) =2 then (√(198x^4 −868x^3 −229x^2 +200x)) =? |
| ∫_0 ^( ∞) (((cos(2cos(x)+1)+1)^(3/2) )/((ln((√(x^4 +1))−x))^4 )) dx |

|
| ∫_0 ^(π/3) ((tanx)/( (√(2cosx−1))))dx |

|

|

|
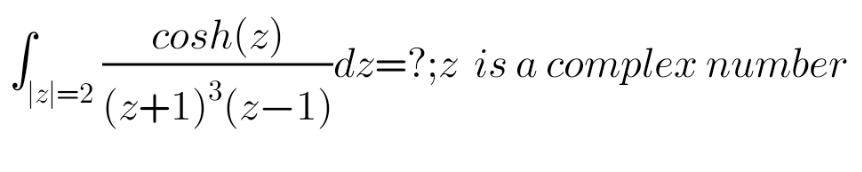
|
Pg 601 Pg 602 Pg 603 Pg 604 Pg 605 Pg 606 Pg 607 Pg 608 Pg 609 Pg 610 |