Question and Answers Forum

AllQuestion and Answers: Page 708

Question Number 142341 Answers: 1 Comments: 1

Question Number 142338 Answers: 2 Comments: 0

∫_0 ^∞ ((sinx)/x^μ )dx =?

$\int_{0}^{\infty} \frac{s i n x}{x^{μ}} d x = ?$

Question Number 142325 Answers: 0 Comments: 0

Given that a ≥ 1 ≥ b > 0. Prove the followings: (1) (1/2)(a−b)^2 ≤ (a−1)^2 +(1−b)^2 ≤ (a−b)^2 (2) (1/4)(a−b)^3 ≤ (a−1)^3 +(1−b)^3 ≤ (a−b)^3

$Given that a ⩾ 1 ⩾ b > 0 . Prove the followings :$ $(1) \frac{1}{2} {(a - b)}^{2} ⩽ {(a - 1)}^{2} + {(1 - b)}^{2} ⩽ {(a - b)}^{2}$ $(2) \frac{1}{4} {(a - b)}^{3} ⩽ {(a - 1)}^{3} + {(1 - b)}^{3} ⩽ {(a - b)}^{3}$

Question Number 142349 Answers: 2 Comments: 0

Σ_(n=0) ^∞ ((ζ(2n+2)(−1)^n )/4^n )=?

$\underset{n = 0}{\sum^{\infty}} \frac{ζ (2 n + 2) {(- 1)}^{n}}{4^{n}} = ?$

Question Number 142348 Answers: 1 Comments: 0

(((4−(√(15))))^(1/6) /( (√(4−(√(15)))) ∙ ((4+(√(15))))^(1/3) )) = ?

$\frac{\sqrt[6]{4 - \sqrt{15}}}{\sqrt{4 - \sqrt{15}} \cdot \sqrt[3]{4 + \sqrt{15}}} = ?$

Question Number 142318 Answers: 1 Comments: 0

Nice...≽≽≽∗∗∗≼≼≼...Calculus Ω:=∫_0 ^( 1) (((1−(x)^(1/3) )(1−((x ))^(1/5) )(1−(x)^(1/7) ))/(ln( ((x ))^(1/3) ))) dx=? ....m.n

$N i c e . . . ≽≽≽ * * * ≼≼≼ . . . C a l c u l u s$ $Ω := \int_{0}^{1} \frac{(1 - \sqrt[3]{x}) (1 - \sqrt[5]{x}) (1 - \sqrt[7]{x})}{l n (\sqrt[3]{x})} d x = ?$ $. . . . m . n$

Question Number 142333 Answers: 2 Comments: 1

Question Number 142329 Answers: 2 Comments: 0

lim_(x→∞) (((x!)/x^x ))^(1/x)

${l i m}_{x \to \infty} {(\frac{x!}{x^{x}})}^{\frac{1}{x}}$

Question Number 142326 Answers: 2 Comments: 0

Question Number 142310 Answers: 2 Comments: 0

Show that for n∈ N, A_n =n^2 (n^2 −1) is divisible by 12

$Show that for n \in N, A_{n} = n^{2} (n^{2} - 1)$ $is divisible by 12$

Question Number 142309 Answers: 1 Comments: 0

lim_(x→0^+ ) ((x^((sin x)^x ) −(sin x)^x^(sin x) )/x^3 )=?

$\lim_{x \to 0^{+}} \frac{x^{{(\sin x)}^{x}} - {(\sin x)}^{x^{\sin x}}}{x^{3}} = ?$

Question Number 142308 Answers: 0 Comments: 0

lim_(x→0) ((lnlnln[x+(1+x)^(((1+x)^(1/x) )/x) ]+x(1−(1/e^(e+1) )))/x^2 )=?

$\lim_{x \to 0} \frac{lnlnln [x + {(1 + x)}^{\frac{{(1 + x)}^{\frac{1}{x}}}{x}}] + x (1 - \frac{1}{e^{e + 1}})}{x^{2}} = ?$

Question Number 142304 Answers: 0 Comments: 0

Question Number 142305 Answers: 1 Comments: 0

Question Number 142301 Answers: 1 Comments: 0

Question Number 142300 Answers: 0 Comments: 0

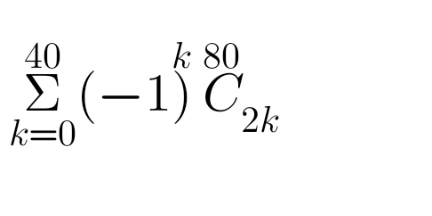
lim_(x→0) (((e^(sin x) +sin x)^(1/(sin x)) −(e^(tan x) +tan x)^(1/(tan x)) )/x^3 )=?

$\lim_{x \to 0} \frac{{(e^{\sin x} + \sin x)}^{\frac{1}{\sin x}} - {(e^{\tan x} + \tan x)}^{\frac{1}{\tan x}}}{x^{3}} = ?$

Question Number 142299 Answers: 0 Comments: 0

Question Number 142290 Answers: 1 Comments: 0

evaluate: Θ:=Σ_(n=1) ^∞ ((ζ(n+1)−1)/(n+1)) =?

$e v a l u a t e :$ $Θ := \underset{n = 1}{\sum^{\infty}} \frac{ζ (n + 1) - 1}{n + 1} = ?$

Question Number 142285 Answers: 2 Comments: 0

L(((1+2bt)/( (√t)))e^(bt) )(s)=?

$L (\frac{1 + 2 bt}{\sqrt{t}} e^{bt}) (s) = ?$

Question Number 142282 Answers: 1 Comments: 0

Three interior angles of a polygon are 160° each. If the other interior angles are 120° each, find the number of sides of the polygon.

$Three interior angles of a polygon are 160 °$ $each . If the other interior angles are 120 ° each,$ $find the number of sides of the polygon .$

Question Number 142307 Answers: 1 Comments: 0

lim_(x→0) ((tan (tan x)−tan (tan (tan x)))/(tan x∙tan (tan x)∙tan (tan (tan x))))=?

$\lim_{x \to 0} \frac{\tan (\tan x) - \tan (\tan (\tan x))}{\tan x \cdot \tan (\tan x) \cdot \tan (\tan (\tan x))} = ?$

Question Number 142306 Answers: 0 Comments: 0

Question Number 142277 Answers: 2 Comments: 0

lim_(x→0) ((1−e^(sin x ln (cos x)) )/x^3 ) =?

$\lim_{x \to 0} \frac{1 - e^{\sin x \ln (\cos x)}}{x^{3}} = ?$

Question Number 142276 Answers: 1 Comments: 0

∫_0 ^( 2) (√(1+e^(2x) ))dx

$\int_{0}^{2} \sqrt{1 + e^{2 x}} d x$

Question Number 142275 Answers: 0 Comments: 0

.......Advanced ...∗∗∗∗∗ ...Integral...... Prove that :: Φ :=∫_0 ^( 1) ((1−x)/((1−x+x^2 )log(x)))dx= proof:: Φ:=∫_0 ^( 1) ((1−x^2 )/((1−x^3 )log(x)))dx f (a):= ∫_0 ^( 1) ((1−x^a )/((1−x^3 )log(x))) Φ := f (2) ........✓ f ′(a):=∫_0 ^( 1) ((−x^a log(x))/((1−x^3 )log(x)))=∫_0 ^( 1) ((−x^a )/(1−x^3 ))dx (★) (★):: x^3 =y ⇒ f ′(a):=(1/3)∫_0 ^( 1) ((y^((−2)/3) −y^((a/3)−(2/3)) )/(1−y))dy :=(1/3)∫_0 ^( 1) ((y^((−2)/3) −1+1−y^((a/3)−(1/3)) )/(1−y))dy :=(1/3)(ψ((a/3)+(2/3))−ψ((2/3))) f (a):=log(Γ((a/3)+(2/3)))−(a/3)ψ((2/3))+C f (0):=0=log(Γ((2/3)))+C C :=−log(Γ((2/3))) Φ:= f (2)=log(Γ((4/3)))−(2/3) ψ((2/3))−log(Γ((2/3))) :=log(((Γ((4/3)))/(Γ((2/3)))))−(2/3)ψ((2/3)) ....✓

$. . . . . . . A d v a n c e d . . . * * * * * . . . I n t e g r a l . . . . . .$ $P r o v e t h a t :: Φ := \int_{0}^{1} \frac{1 - x}{(1 - x + x^{2}) l o g (x)} d x =$ $p r o o f ::$ $Φ := \int_{0}^{1} \frac{1 - x^{2}}{(1 - x^{3}) l o g (x)} d x$ $f (a) := \int_{0}^{1} \frac{1 - x^{a}}{(1 - x^{3}) l o g (x)}$ $Φ := f (2) . . . . . . . . ✓$ $f^{'} (a) := \int_{0}^{1} \frac{- x^{a} l o g (x)}{(1 - x^{3}) l o g (x)} = \int_{0}^{1} \frac{- x^{a}}{1 - x^{3}} d x (★)$ $(★) :: x^{3} = y \Rightarrow f^{'} (a) := \frac{1}{3} \int_{0}^{1} \frac{y^{\frac{- 2}{3}} - y^{\frac{a}{3} - \frac{2}{3}}}{1 - y} d y$ $:= \frac{1}{3} \int_{0}^{1} \frac{y^{\frac{- 2}{3}} - 1 + 1 - y^{\frac{a}{3} - \frac{1}{3}}}{1 - y} d y$ $:= \frac{1}{3} (ψ (\frac{a}{3} + \frac{2}{3}) - ψ (\frac{2}{3}))$ $f (a) := l o g (Γ (\frac{a}{3} + \frac{2}{3})) - \frac{a}{3} ψ (\frac{2}{3}) + C$ $f (0) := 0 = l o g (Γ (\frac{2}{3})) + C$ $C := - l o g (Γ (\frac{2}{3}))$ $Φ := f (2) = l o g (Γ (\frac{4}{3})) - \frac{2}{3} ψ (\frac{2}{3}) - l o g (Γ (\frac{2}{3}))$ $:= l o g (\frac{Γ (\frac{4}{3})}{Γ (\frac{2}{3})}) - \frac{2}{3} ψ (\frac{2}{3}) . . . . ✓$

Question Number 142273 Answers: 0 Comments: 1

∫_(1/3) ^3 ((x+sin (x^2 −(1/x^2 )))/(x(2+cos (x+(1/x))))) dx ?

$\underset{\frac{1}{3}}{\int^{3}} \frac{x + \sin (x^{2} - \frac{1}{x^{2}})}{x (2 + \cos (x + \frac{1}{x}))} d x ?$

Pg 703 Pg 704 Pg 705 Pg 706 Pg 707 Pg 708 Pg 709 Pg 710 Pg 711 Pg 712

Contact: info@tinkutara.com