
Question and Answers Forum
AllQuestion and Answers: Page 736




Pg 731 Pg 732 Pg 733 Pg 734 Pg 735 Pg 736 Pg 737 Pg 738 Pg 739 Pg 740
|
Question and Answers Forum |
AllQuestion and Answers: Page 736 |
| (√(x^2 +8x)) ≤ 24−(x+2)(x+6) |

|
| ^• I am uncomcofortable and so is my writer. ^• My writer sometimes regrets after writing me and wants to delete me. WHO AM I ? ^■ I am an answer of some question of this forum WITHOUT ANY FEEDBACK OF THE QUESTIONER ! |
| If α,β and γ are the interior angles of a triangle, find the value of determinant (((tan α),1,1),(1,(tan β),1),(1,1,(tan γ))) |
| Γ(z^− )=Γ(z)^(−) why? |
| 1+(π^6 /(27.6!))+(π^(12) /(27^2 .12!))+(π^(18) /(27^3 .18!))+...=((e^(π/( (√a))) +e^(−(π/( (√a)))) )/(2a)) Find a |
| ....advanced ....★★★.....calculus..... :=Σ_(n=1) ^∞ (((sin(n))/n))^3 =? |
| ......... nice ... ... ... calculus........ Φ:=∫_0 ^( 1) ((ln(((1+x)/2)))/(x^2 −1))dx=(π^2 /(24)) NOTE :: li_2 (z)+li_2 (1−z)=(π^2 /6)−ln(z)ln(1−z) Hence :: li_2 ((1/2))=(π^2 /(12))−(1/2)ln^2 (2) Φ:=^(⟨ ((1+x)/2)=y ⟩) 2∫_(1/2) ^( 1) ((ln(y))/(4y^2 −4y))dy :=(1/2)∫_(1/2) ^( 1) ((ln(y))/(y(y−1)))dy=(1/2) ∫_(1/2) ^( 1) {((ln(y))/(y−1))−((ln(y))/y)}dy :=−(1/2) [(1/2) ln^2 (y)]_((1 )/2) ^1 +(1/2){li_2 (1)−li_2 ((1/2))} :=(1/4)ln^2 (2)+(π^2 /(12))+(1/2)(−(π^2 /(12))−(1/2)ln^2 (2)) Φ:=(π^2 /(24)) |

|
| let: Ω_n =∫_( 0) ^( 2π) cos(x)∙cos(2x)∙...∙cos(nx) dx for which integers n, 1≤n≤10, is Ω_n ≠0? |
| prove: (√(3+(√2)−(√(9+4(√2))))) = (√(2−(√2))) |
| _ prove that :: Σ_(n=0) ^∞ (1/((3n)!)) =(e/3)+(2/(3(√e))) cos(((√3)/2)) |
| E=log_a (√(c/b^(log_(ab) c) ))+log_b (√(c/a^(log_(ab) c) )) |
| # calculus# evaluate: 𝛗:=Σ_(k=1) ^∞ (((−1)^(k−1) Γ ((k/2)))/(k Γ(((k+1)/2)))) =? |
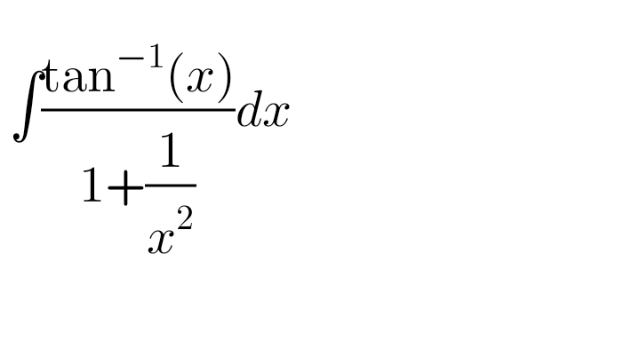
|
| If w≠1 is a cube root of unity, x=a+b, y=aw+bw^2 and z=aw^2 +bw, then x^3 +y^3 +z^3 =? |
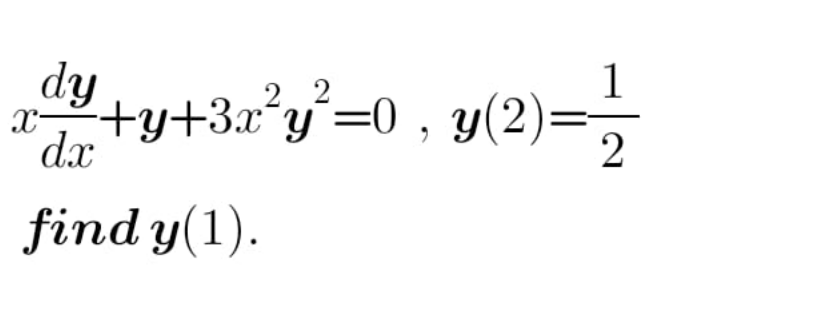
|

|
| ∫ sin^4 (12x) ((cos 6x))^(1/(5 )) dx =? |
| ∫_( (√2)) ^(3(√2)) (√(x^2 −2))dx+∫_0 ^4 (√(x^2 +2))dx =? |

|

|
| C_n ^( 2) =(1/8)P_(n ) ^( 8) find n |
| ABCD is a rectangle such that AD=2AB and its center is O. H is the top of a pyramid which has ABCD as base. All lateral faces are isosceles triangles. planes (HAB) and (HCD) are ⊥. i have joined a graphic. 1. show that (OH)⊥(ABC). 2. show that OH=((√3)/2)AB |
| ∫_( 0) ^( π/2) ((cos^2 x)/(cos(x−π/4))) dx |
| hi ! prove this : cos (π/(10)) + cos ((4π)/(10)) + cos ((6π)/(10)) + cos ((9π)/(10)) = 0. (by the easiest possible way...!) |
Pg 731 Pg 732 Pg 733 Pg 734 Pg 735 Pg 736 Pg 737 Pg 738 Pg 739 Pg 740 |