
Question and Answers Forum
AllQuestion and Answers: Page 759







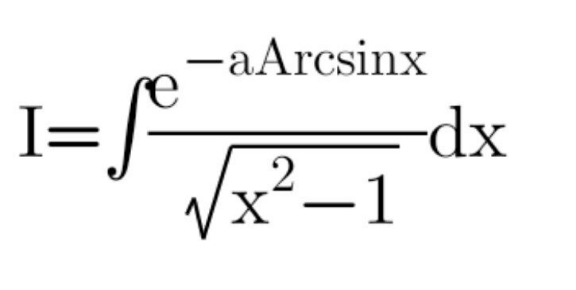
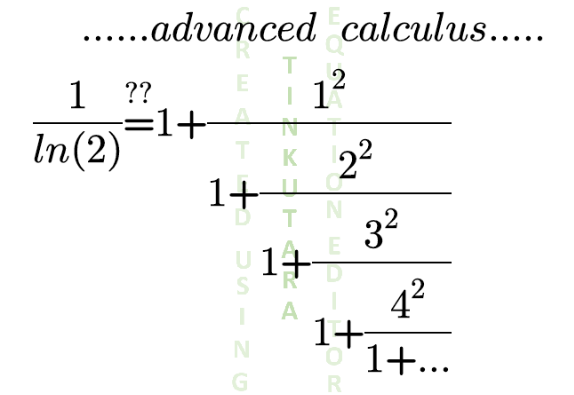

Pg 754 Pg 755 Pg 756 Pg 757 Pg 758 Pg 759 Pg 760 Pg 761 Pg 762 Pg 763
|
Question and Answers Forum |
AllQuestion and Answers: Page 759 |

|

|
| Decompose the function P(x) = ((x^4 +2x^3 +6x^2 +20x+6)/(x^3 +x^2 +x)) in partial fractions. |
| ......Advanced ... calculus...... 𝛗=∫_0 ^( 1) x^2 ln(x)ln(1−x)dx=??? |
| ∫ (((3sin x+2))/((2sin x+3)^2 )) dx =? |

|
| Q137026 |
| Q137014 |

|

|
| If x,y > 0 then prove that 3(√((x^2 +y^2 )/2)) + (√(xy)) ≥ 2(x+y) |

|
| ∫ ((ln(1+x))/x)=? |
| α , β ε (0 , (π/2)) tan^2 α = 1+2tan^2 β ⇒(√2)cosα−cosβ=? |
| ∫ ((cos x)/(1+cos x+sin x)) dx =? |
| Some useful approximations of sine function sin((π/7))=((96)/(221)) sin((π/9))=((128)/(373)) sin((π/(11)))=((32)/(113)) ... I am counting more ..thanking you! |
| ....advanced ....... calculus..... prove that::: 𝛗=∫_0 ^( 1) ln(ln((1/x))).(dx/( (√(ln((1/x)))))) =−(√π) (γ+ln(4)) |

|
| I_n =∫_0 ^(π/2) sin^n x dx Write a relation between I_(n+2) and I_n . |
| ∫_1 ^x e^((1−ln^2 t)^(1/n) ) dt=...? n an integer |
| ......advanced .... calculus.... Φ=Σ_(k=1) ^∞ ((ψ′(k))/k) =Σ_(n=1) ^∞ (a/n^b ) a , b =?? (adapted from brilliant) ................ ψ(k)=^(??) −γ+∫_0 ^( 1) (((1−t^(k−1) )/(1−t)))dt ∴ ψ′(k)=∫_0 ^( 1) ((−t^(k−1) ln(t))/(1−t))dt Σ_(k=1) ^∞ ((ψ′(k))/k)=∫_0 ^( 1) ((−t^(k−1) ln(t))/((1−t)k))dt =∫_0 ^( 1) ((ln(t))/(t(1−t)))(−(t^k /k))dt=∫_0 ^( 1) ((ln(t)ln(1−t))/(t(1−t)))dt=𝛗 𝛗=∫_0 ^( 1) ((ln(t)ln(1−t))/(t(1−t)))dt=𝛗_1 +𝛗_2 where ... ={∫_0 ^( 1) ((ln(t).ln(1−t))/(1−t))dt=𝛗_1 }+{∫_0 ^( 1) ((ln(1−t).ln(t))/t)dt=𝛗_2 } 𝛗_1 =[−(1/2)ln(t)ln^2 (1−t)]_0 ^1 +(1/2)∫_0 ^( 1) ((ln^2 (1−t))/t)dt ∴ 𝛗_1 = (1/2)(2ζ(3))=ζ(3)=^(easy) 𝛗_2 note : ∫_0 ^( 1) ((ln^2 (1−t))/t)dt=2ζ(3) (derived earlier) 𝛗=𝛗_1 +𝛗_2 =2ζ(3)=Σ_(n=1) ^∞ (2/n^3 ) .... 𝚽= Σ_(n=1) ^∞ (a/n^b ) ......⇒a=2 , b=3 |
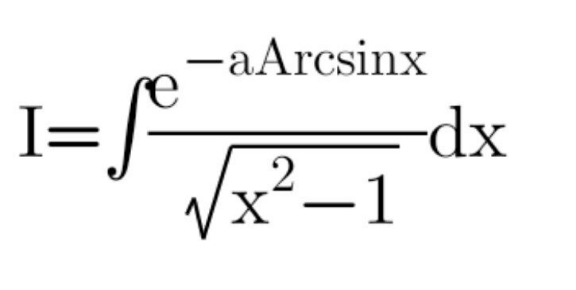
|

|

|
| ......nice calculus ...... evaluate :: 𝛗=∫_0 ^( 2π) (1/(1+cos^4 (x)))dx=??? |
| ∫_1 ^∞ ((√(3x^4 +5x^3 +1))/(4x^3 +x^2 +2)) dx = ... |
Pg 754 Pg 755 Pg 756 Pg 757 Pg 758 Pg 759 Pg 760 Pg 761 Pg 762 Pg 763 |