
Question and Answers Forum
AllQuestion and Answers: Page 760








Pg 755 Pg 756 Pg 757 Pg 758 Pg 759 Pg 760 Pg 761 Pg 762 Pg 763 Pg 764
|
Question and Answers Forum |
AllQuestion and Answers: Page 760 |

|

|

|
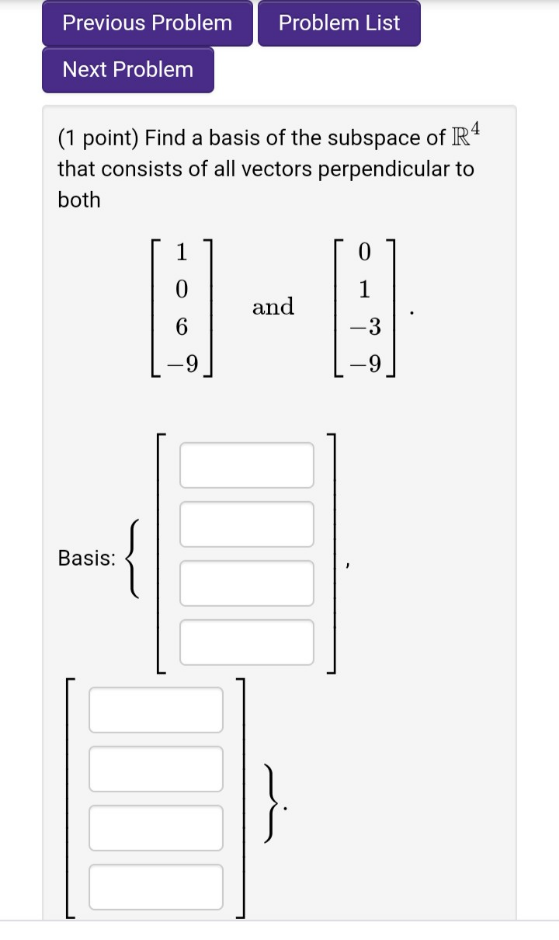
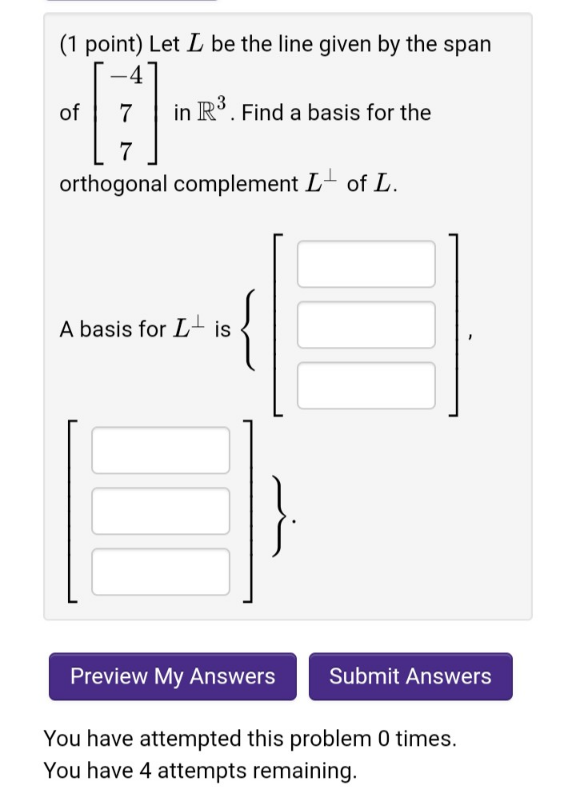
| Proof by mathematical induction that f(n) = n^3 + 5n is a multiple of 6. |
| .......nice ... .... calculus.... evaluate :: Ω=∫_0 ^( 1) (((ln(1−x))/x))^3 =?.... |
| Find the area bounded by the standard normal distribution p(−1.96≤Z≤2.5) |
| prove that Arg(z) is harmonic function and find the conjecate this ? |
| if , acosα+bcosβ=Acos∅ proof that asinα+bsinβ=Asin∅ |

|

|

|

|
| If 2^(2sin^2 θ−3sin θ+1) + 2^(2−2sin^2 θ+3sin θ) = 9 find the value of sin θ+cos θ . |
| Solve the equation (x^2 −x+1)^2 +(y^2 +y+1)^2 =2021 x,y∈Z |
| .......nice ............calculus....... 𝛗=Σ_(n=1) ^∞ ((sin(nx))/n) =(π/2)−(x/2) 𝛗=−Im(1−e^(ix) )=−Imln{(1−cos(x)−isin(x))} =−Im{ln((√((1−cos(x))^2 +sin^2 (x))) +itan^(−1) (((−sin(x))/(1−cos(x))))} =−Im{(√(2−2cos(x)_ )) −itan^(−1) (tan((π/2)−(x/2)))} =tan^(−1) (tan((π/2)−(x/2))) ........ 𝛗=(π/2)−(x/2) ...✓.....⟨∗⟩ ^(′′) ∫ ^(′′) both sides of ⟨∗⟩ −Σ_(n=1) ^∞ ((cos(nx))/n^2 )dx+C=((πx)/2)−(x^2 /4) x=0 ⇒ C=(π^2 /6) ...✓ Σ_(n=1) ^∞ ((cos(nx))/n^2 )=−((πx)/2)+(x^2 /4)+(π^2 /6) ....✓ x=π(1+(√(1/3)) ) .... Σ((cos(nπ(1+(√(1/3)) )))/n^2 )=(1/2)(−π^2 (1+(√(1/3)) )+((π^2 (1+(√(1/3)) )^2 )/2)+(π^2 /3)) =(π^2 /2)(−1−((√3)/3) +((1+2.((√3)/3) +(1/3))/2)+(1/3)) =(π^2 /2)(−1−(((√3) )/3)+(4/6)+(((√3) )/3)+(1/3))=0..✓✓ ......prepared by mr (Dwaipayan)..... m.n# |

|
| ∫log_x edx=?? |
| ∫_0 ^∞ ((sin x−sin x^2 )/x)=(π/4) ∫_0 ^∞ ((cos x−cos x^2 )/x)dx=−(γ/2) |

|
| .......nice ... ... .... calculus..... prove that :::: 𝛗=∫_0 ^( 1) (((log(1−x))/x))^2 dx=2ζ(2).... |
| lim_(x→π/2) (((1−tan (x/2))(1−sin x))/((1+tan (x/2))(π−2x)^3 )) ? |

|
| Let f(x)=x^2 −2x−3; x≥1 & g(x)=1+(√(x+4)) ; x≥−4 then the number of real solutions of equation f(x)=g(x) is ... |
| cos (arctan (((21)/(60))))=? |
| Given { ((cos (x−y)=−1+(1/2)cos x)),((cos (x+y)= 1+(1/3)cos x)) :} where 270° < y< 360° . find sin 2y . |
| ..... mathematical .. ... ... analysis.... evaluate :: 𝛗=∫_0 ^( 1) (((ln^2 (1−x^2 ))/x))=? |
Pg 755 Pg 756 Pg 757 Pg 758 Pg 759 Pg 760 Pg 761 Pg 762 Pg 763 Pg 764 |