
Question and Answers Forum
AllQuestion and Answers: Page 762





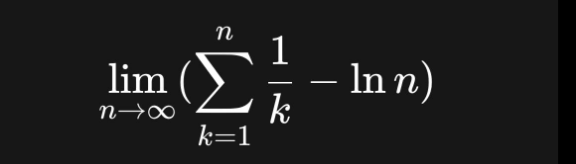



Pg 757 Pg 758 Pg 759 Pg 760 Pg 761 Pg 762 Pg 763 Pg 764 Pg 765 Pg 766
|
Question and Answers Forum |
AllQuestion and Answers: Page 762 |

|
| x=2^(p ) and y=2^(q ) . Evaluate in terms of x and/ or y (i)2^(p+q) (ii) 2^(2q ) (iii) 2^(p−1) |

|

|

|
| (dy/dx) = ((6xy+y^2 )/(x+y)) |

|
| ∫_0 ^( (π/2)) ln(((1+tanx)/(1−tanx)))^(xcos(8x)) dx |
| Two particles of mass m and 4m are connected by a light inelastic string of length 3a, which passes through a small smooth fixed ring. The heavier particle hangs at rest at a distance 2a beneath the ring, while the lighter particle describes a horizontal circle with constant speed. Find (a) The distance of the plane of the circle below the ring. (b) The angular speed of the lighter particle. |
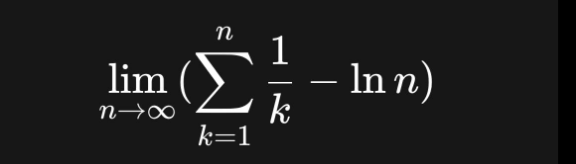
|
| ........ mathematical analysis (II).... prove that :: 𝛀=∫_0 ^( 1) (1/(1+x))ln(((x^2 +2x+1)/(1+x+x^2 )))=Σ_(n=1) ^∞ (1/(n^2 (((2n)),(( n)) )))=(π^2 /(18)).. |
| .....nice calculus... prove that:: Σ_(n=0) ^∞ tan^(−1) ((1/F_n )).tan^(−1) ((1/F_(n+1) ))=(π^2 /4) F_n is fibonacci sequence.... |

|
| Find the minimum value of x^(2) +y^(2) +z^(2) , subject to the condition 2x+3y+5z=30? |
| (x+(√(x^2 +1)))(y+(√(y^4 +4)))=9 x(√(y^4 +4))+y(√(x^2 +1))=? |
| ......advanced.....calculus.... 𝛀=Σ_(n=1) ^∞ ((ψ′′(n))/n)=??? I havefound :: Ω=−(π^4 /(36)) ... ! |
| a=(4)^(1/3) +(2)^(1/3) +(1)^(1/3) (3/a)+(3/a^2 )+(1/a^3 )=? |
| x=1+((π+(π+1)^2 +(π+2)^3 +(π+3)^4 )/(4+5^2 +6^3 +7^4 )) (√(x+2(√(x−1))))+(√(x−2(√(x−1))))=? |
| For a positive number n , let f(n) be the value of f(n)=((4n+(√(4n^2 −1)))/( (√(2n+1)) +(√(2n−1)))) calculate f(1)+f(2)+f(3)+...+f(40). |
| Find the cube of the number N= (√(7(√(3(√(7(√(3(√(7(√(3(√(7(√(3...)))))))))))))))) |
| Given { ((a_(2n) = a_n .a_2 +1)),((a_(2n+1) = a_n .a_2 −2 )) :} and { ((a_7 = 2)),((0<a_1 <1)) :}. Find a_(25) =? |
| A=(((1/3)((2)^(1/3) −1)((2)^(1/3) +1)^3 ))^(1/3) |
| (−1)×(1/(π.i)) =? |

|

|

|
Pg 757 Pg 758 Pg 759 Pg 760 Pg 761 Pg 762 Pg 763 Pg 764 Pg 765 Pg 766 |