
Question and Answers Forum
AllQuestion and Answers: Page 991



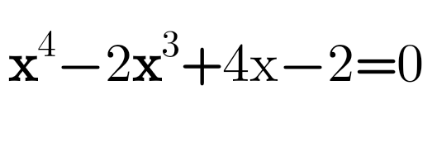




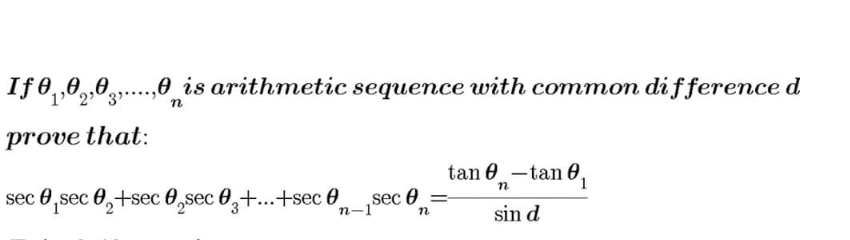
Pg 986 Pg 987 Pg 988 Pg 989 Pg 990 Pg 991 Pg 992 Pg 993 Pg 994 Pg 995
|
Question and Answers Forum |
AllQuestion and Answers: Page 991 |

|
| 0^0 =? |

|

|
| Evaluate: ∫ (1/(sin^5 x + cos^5 x)) dx |
| Solve for ∣x−2∣+∣1−x∣=4 |
| Find the maximum. and minimum value of ⌊1+sinx⌋+⌊1+sin3x⌋+⌊1+sin2x⌋ |
| Find period of f(x)=e^(cos^4 (πx)+x−⌊x⌋+cos^2 (πx)) |
| Π_(n=1) ^∞ ((4n^2 (10n−6)(10n−4))/((2n−1)^2 (10n−1)(10n+1))) =? |
| Without L′Hopital lim_(x→(π/4)) ((((1/(cos^2 x)) −2tan x ))/(cos 2x)) ? |
| find minimum value of function f(x) = (((x+17)^3 )/x) , x>0 |
| question proposed by m.n july 1970 show that ∫_0 ^∞ ((ln(1+x))/(x(1+x^2 )))dx=((5π^2 )/(48)) |
| find the largest and smallest coefficient in (4+3x)^(−5) . |
| .... nice mathematics... prove that:: Σ_(n=1) ^∞ (([ (((2n)),(n) )]^2 )/((2n−1)2^(4n) )) =1−(2/π) ... m.n.july. 1970# |
| (3+4x)^(−5) =3^(−5) ×(1+(4/3)x)^(−5) ... pls what is the explanation behind the fact that i always have to factorize to get the form (1+b)^(−s) any time i am solving for binomial with negatuve power? can it be solved without first factorizing? |
| a_n (d^n Ψ/dt^n )+a_(n−1) (d^(n−1) Ψ/dt^(n−1) )+.....+a_1 (dΨ/dt)+a_0 Ψ=0 Is it solvable??? |
| ....nice calculus.... prove that:: Φ=∫_(0 ) ^( 1) xln[ln(x).ln(1−x)]dx=−γ γ ::= euler mascheroni constant. ...m.n.july.1970... |
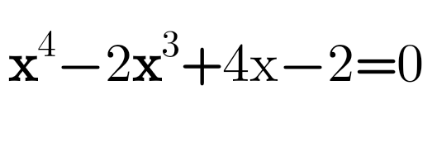
|

|

|

|

|
| ∫_0 ^(π/2) (dx/( (√(1+tan^4 x)))) ? |
| lim_(x→0) ((sinh (2x)−sin 2x)/x^5 ) =? |
| ∫xsin^n xdx |
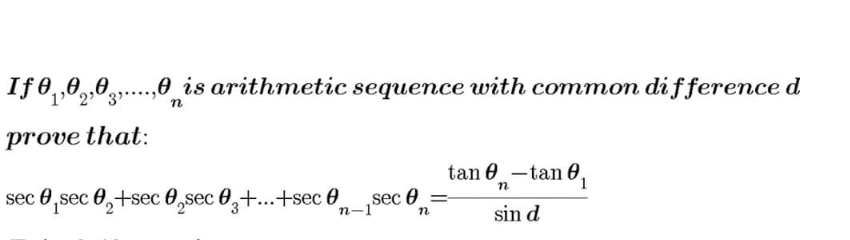
|
Pg 986 Pg 987 Pg 988 Pg 989 Pg 990 Pg 991 Pg 992 Pg 993 Pg 994 Pg 995 |