
Question and Answers Forum
AllQuestion and Answers: Page 998




Pg 993 Pg 994 Pg 995 Pg 996 Pg 997 Pg 998 Pg 999 Pg 1000 Pg 1001 Pg 1002
|
Question and Answers Forum |
AllQuestion and Answers: Page 998 |
| Montrer que pour 0<z<1 on a Γ(z)Γ(1−z)=(π/(sin(πz))) |
| Bonjour besoin d′aide Calculer ∫ln(cosx)dx |
| calculate U_n =∫_(1/n) ^n (1+(1/t^2 ))arctan(1−(1/t))dt find lim_(n→+∞) U_n |
| explicit g(a) =∫_0 ^(π/4) ln(1+acos^2 θ)dθ |
| find f(a) =∫_0 ^(π/8) ln(1+a sinθ)dθ with o<a<1 |
| lim_(x→0) (((1+x)^(1/x) −e−((ex)/2))/x^2 ) =? |

|
| A bag contains 6 red, 5 white and 4 black balls If twl balls are drawn , what is the probability that none of them are red ? |
| if A={1,2} and let R={(a,b)∈A×A:a=3b} then R= ? help me sir |

|
| Prouver que β(a,b)=((Γ(a)Γ(b))/(Γ(a+b)))=∫_0 ^1 x^(a−1) (1−x)^(b−1) dx |
| (6/(5×14))+(6/(14×23))+(6/(23×32))+...+(6/((9n−4)(9n+5)))=? |
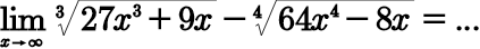
|
| prove the following integral ∫_0 ^(π/2) (x^2 /(sinx))dx=2πG−(7/2)ζ(3) ∫_0 ^1 ln[((x+(√(1−x^2 )))/(x−(√(1−x^2 ))))]^2 (x/(1−x^2 ))dx=((.π^2 )/2) ∫_0 ^∞ ((e^x lnx)/(1+e^(2x) ))dx=(π/2)ln[((Γ((3/4)))/(Γ((1/4))))(√(2π))] |

|
| find ∫ (dx/((x+1)(√(x^2 −1))+(x−1)(√(x^2 +1)))) |
| calculate ∫_0 ^∞ (dx/(x^4 +ix^2 +2)) |

|
| lim_(r→∞) (√(4r^2 +2r)) −((8r^3 +4r^2 ))^(1/(3 )) =? |
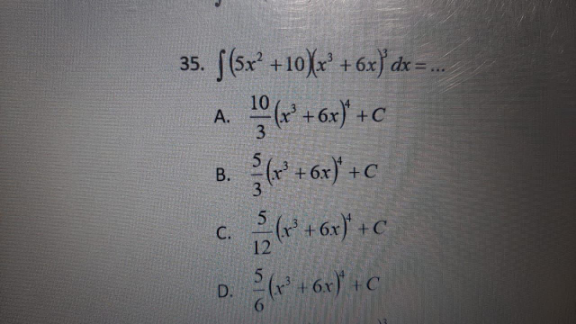
|
| ∫_1 ^2 f(2x+1) dx = 3 and ∫_1 ^2 (x^2 f(x^3 +2))dx = 5 then ∫_5 ^(10) f(x) dx = ? |
| calculate ∫_0 ^∞ (dx/(x^4 +ix^2 +2)) |
| Using displacement vector (((−4)),((−2)) ), what is the image of ((6),(3) ) when translated? |

|
| let A = (((1 −(1/n))),(((1/n) 1)) ) 1) calculate A^2 2) calculate A^m (m integr natural) 3) coclude A^n and lim_(n→+∞) A^n 4) calculate e^A and e^(−A) |
| App Update v2.203 A function plotter for one variable is available. Steps to use Insert Drawing Insert Equation select equation from the list of shape tap on BUILD and select plot A sample plot is show as image below. Line color for plot can be changed using edit shape menu to change range of x values select scale from options menu. to zoomin/out use option menu to scroll with zoom: clear selection and place touch in move mode. You can add multiple plots on one drawing and also add another drawing on the canvas. |
Pg 993 Pg 994 Pg 995 Pg 996 Pg 997 Pg 998 Pg 999 Pg 1000 Pg 1001 Pg 1002 |