Determine x^4 − (1/x^4 ) , if x^2 + (1/x^2 )=34 .


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 8148 by Rasheed Soomro last updated on 02/Oct/16

$Determine x^{4} - \frac{1}{x^{4}}, if x^{2} + \frac{1}{x^{2}} = 34 .$
Commented by sou1618 last updated on 02/Oct/16

$s e t X = x^{2} ⩾ 0$ $X + \frac{1}{X} = 34$ ${(X - \frac{1}{X})}^{2} = {(X + \frac{1}{X})}^{2} - 4 = 34^{2} - 2^{2} = 36 \times 32$ $\Rightarrow$ $X - \frac{1}{X} = \pm 24 \sqrt{2}$ $X^{2} - \frac{1}{X^{2}} = (X + \frac{1}{X}) (X - \frac{1}{X}) = 34 \times (\pm 24 \sqrt{2})$ $= \pm 816 \sqrt{2}$
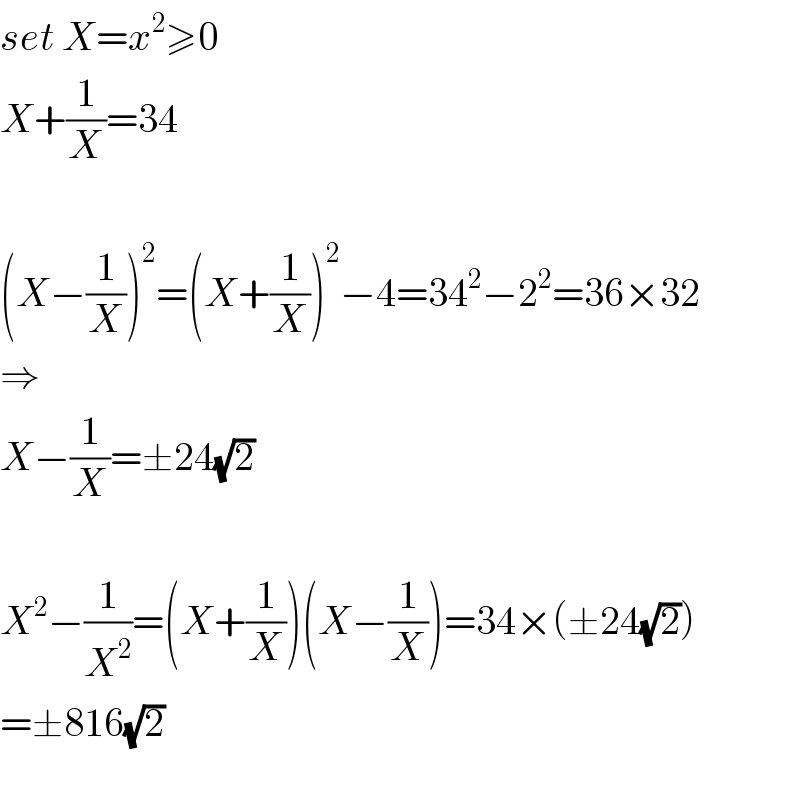
Commented by Rasheed Soomro last updated on 02/Oct/16

$Thank S! G \underset{\overset{}{⌣}}{\overset{⌢}{∙}} \overset{⌢}{∙} D approach!$
	Answered by trapti rathaur@ gmail.com last updated on 02/Oct/16

	$Missing \left or extra \right$ $34 \times 34 = x^{4} + \frac{1}{x^{4}} + 2$ $x^{4} + \frac{1}{x^{4}} = 1154$ $Missing \left or extra \right$ $Missing \left or extra \right$ ${(1154)}^{2} = {(x^{4} - \frac{1}{x^{4}})}^{2} + 4$ ${(x^{4} - \frac{1}{x^{4}})}^{2} = {(1154)}^{2} - {(2)}^{2}$ $(x^{4} - \frac{1}{x^{4}}) = \sqrt{1331712}$ $= 816 \sqrt{2}$ $A N S W E R -$ $(x^{4} - \frac{1}{x^{4}}) = 816 \sqrt{2}$
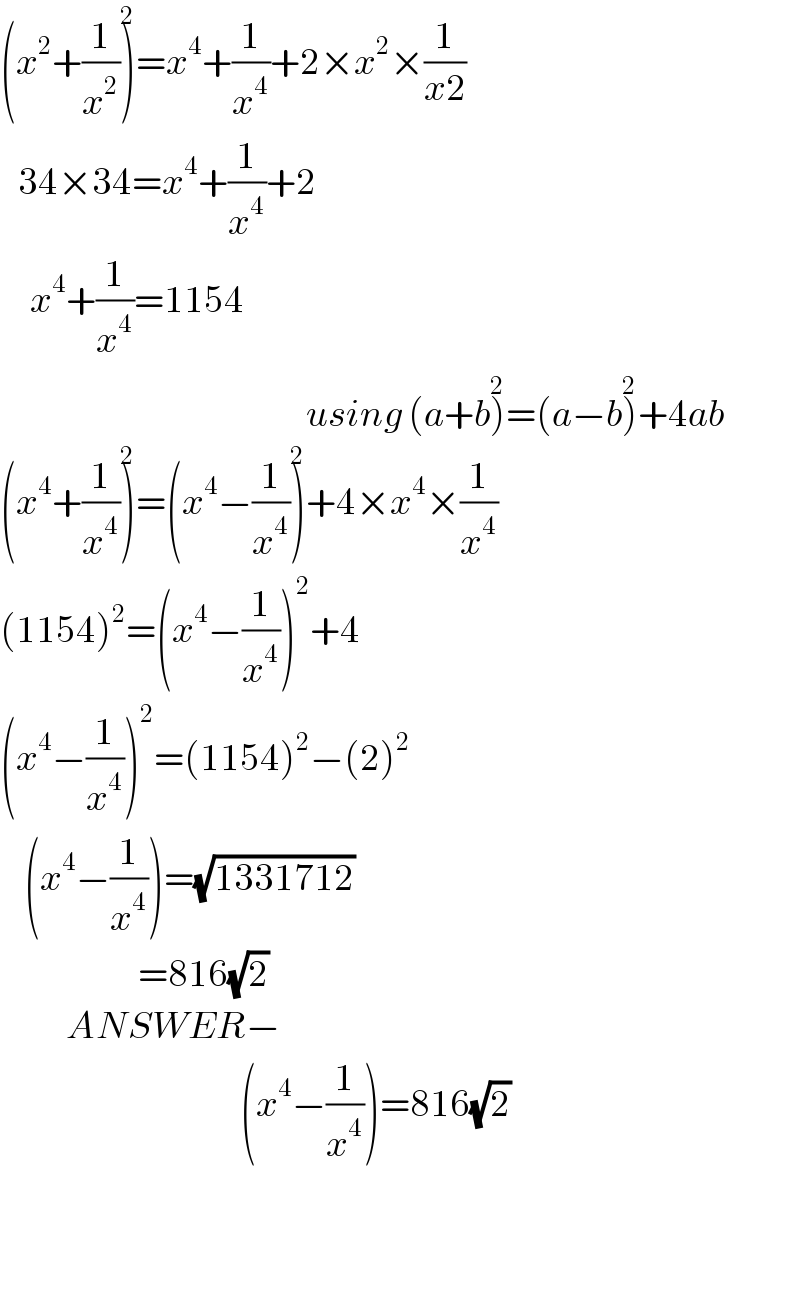
	Commented by Rasheed Soomro last updated on 02/Oct/16

	$Thank S!$ $You lost one answer by taking only + ve$ $squareroot of 1331712 : (x^{4} - \frac{1}{x^{4}}) = \sqrt{1331712}$ $You should have written$ $(x^{4} - \frac{1}{x^{4}}) = \pm \sqrt{1331712} = \pm 816 \sqrt{2}$
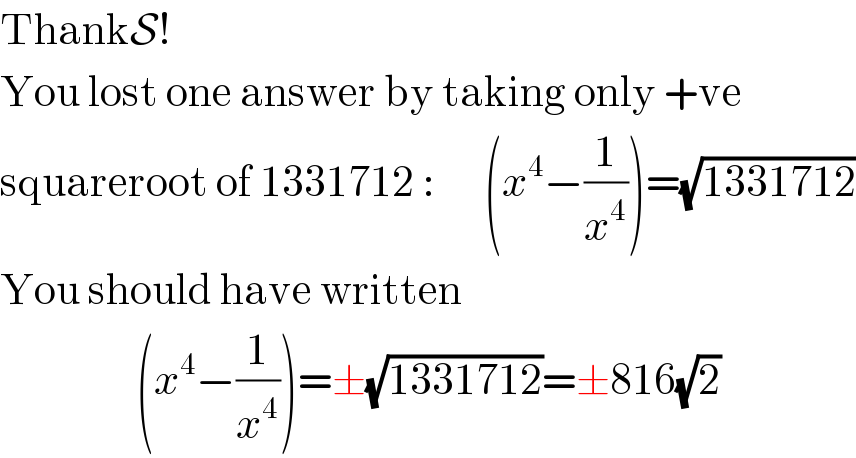
	Commented by trapti rathaur@ gmail.com last updated on 02/Oct/16

	$t h a n k s . i f o r g e t i t$
	Answered by Rasheed Soomro last updated on 02/Oct/16
	$x^2 + (1/x^2 )=34 , x^4 − (1/x^4 )=? Approach: x^2 + (1/x^2 )→ { ((x+ (1/x))),((x− (1/x))) :} →x^2 − (1/x^2 )∧x^2 + (1/x^2 )→x^4 − (1/x^4 ) x^2 + (1/x^2 )=34 x^2 +2+ (1/x^2 )=34+2 ∧ x^2 −2+ (1/x^2 )=34−2 (x+(1/x))^2 =(±6)^2 ∧ (x−(1/x))^2 =(±4(√2) )^2 x+(1/x)=±6 ∧ x−(1/x)=±4(√2) ⇒x^2 − (1/x^2 )=±24(√2) {: ((x^2 − (1/x^2 )=±24(√2))),(( × )),((x^2 + (1/x^2 )=34 (given))) }⇒x^4 −(1/x^4 )=±816(√2)$
	$x^{2} + \frac{1}{x^{2}} = 34, x^{4} - \frac{1}{x^{4}} = ?$ $Approach :$ $x^{2} + \frac{1}{x^{2}} \to {\begin{cases} x + \frac{1}{x} \\ x - \frac{1}{x} \end{cases} \to x^{2} - \frac{1}{x^{2}} \land x^{2} + \frac{1}{x^{2}} \to x^{4} - \frac{1}{x^{4}}$ $x^{2} + \frac{1}{x^{2}} = 34$ $x^{2} + 2 + \frac{1}{x^{2}} = 34 + 2 \land x^{2} - 2 + \frac{1}{x^{2}} = 34 - 2$ ${(x + \frac{1}{x})}^{2} = {(\pm 6)}^{2} \land {(x - \frac{1}{x})}^{2} = {(\pm 4 \sqrt{2})}^{2}$ $x + \frac{1}{x} = \pm 6 \land x - \frac{1}{x} = \pm 4 \sqrt{2}$ $\Rightarrow x^{2} - \frac{1}{x^{2}} = \pm 24 \sqrt{2}$ $\begin{matrix} x^{2} - \frac{1}{x^{2}} = \pm 24 \sqrt{2} \\ \times \\ x^{2} + \frac{1}{x^{2}} = 34 (given) \end{matrix}} \Rightarrow x^{4} - \frac{1}{x^{4}} = \pm 816 \sqrt{2}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum