
Question and Answers Forum
DifferentiationQuestion and Answers: Page 11



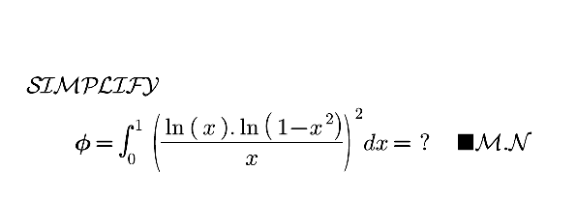
|
Question and Answers Forum |
DifferentiationQuestion and Answers: Page 11 |
| In AB^Δ C : cos^( 2) (A )+ cos^( 2) (B )+ cos^( 2) ( C )=1 . Prove that AB^Δ C is right angled. −−−−−−−− |
| prove that Ω=∫_0 ^( 1) ln(((1+x)/(1−x)) ).(dx/(x (√( 1−x^( 2) )))) = (π^( 2) /2) −− m.n−− |
| Air leaks from a spherical ballon so that it maintains its shape at a rate of 25 cc/m .What is the rate of change in the length of the radius of the balloon when the radius is 5 cm |
| f(x)=((2x^(100!) )/(100!))+x^(100) +1 find ((d^(100!) f(x))/dx^(100!) )=? |
| If , 𝛗 = ∫_(−∞) ^( +∞) (( sin(x).ln^( 2) (x ))/x) then find : Im (𝛗 ) = ? ■ m.n |
| Ω= Σ_(n=1) ^∞ n(ζ (1+n) −1) =? |
| K(x) = ((3 cos x)/(5+4sin x)) {: ((max K(x))),((min K(x))) } =? |
| Ω= ∫_0 ^( 1) (Li_( 2) ^ (x ))^( 2) dx = ? ■ m.n −−− −−− |

|
| Re^ soudre (∂^2 u/∂x^2 )+(∂^2 u/∂y^2 )=e^(2x+y) |
| If 𝛗=∫_0 ^( (π/2)) (( 1)/( (√(sin^( 5) (x).cos(x))) +(√(cos^( 5) (x).sin(x)))))dx = find the value of : Γ^( 2) ((3/4) ). 𝛗 |
| Ω= ∫_0 ^( 1) (( sin^( 2) ( ln(x )). ln (x))/( (√x))) dx=? −−−−− |
| prove Ω= ∫_0 ^( ∞) cot^( −1) (1+x^( 2) )=((√((1/( (√2)))−(1/2))) ) π |

|
| put : gcd( a , b )= (a, b ) if ( a ,b )= (a ,c )= (b ,c )=1 prove that : (abc , ab +ac +bc )=1 |
| Re ( ∫_0 ^( 1) sin^( −1) ((( 1)/(1− x^( 2) )) )dx )=? |

|
| prove or disprove ∫_(2π) ^( 4π) (( sin(x))/x) dx >0 because ∫_(2π) ^( 3π) (( sin(x ))/x) dx > ∫_(3π) ^( 4π) ((∣sin(x)∣)/x) dx |
| F(x)= (((x^2 −4x)^2 ))^(1/3) {: ((local maximum)),((absolut maximum)) } =? |
| prove that Σ_(n=1) ^∞ (( ( 2n+1 )!!)/((2n )!!)) (1/(2^( n) (2n +1)^( 2) )) =((π(√2))/4)−1 |
| Ω= ∫_0 ^( ∞) (( x − sin (x ))/x^( 3) )dx −−− solution−−− Ω=^(I.B.P) [ ((−1)/(2 x^( 2) )) (x−sin(x))]_0 ^∞ +(1/2) ∫_0 ^( ∞) ((1−cos (x))/x^( 2) )dx = (1/2) ∫_0 ^( ∞) (( 2sin^( 2) ((x/2)))/x^( 2) )dx=∫_0 ^( ∞) ((sin^( 2) ((x/2)))/x^( 2) )dx =^((x/2) = α) (1/2)∫_0 ^( ∞) ((sin^( 2) ( α))/α^( 2) ) dα = (1/2) [((−1)/α) sin^( 2) (α)]_0 ^∞ +(1/2)∫_0 ^( ∞) ((sin(2α))/α)dα =^(2α=ϕ) (1/2) ∫_0 ^( ∞) (( sin(ϕ ))/ϕ) dϕ =(π/4) −− Ω= (π/4) −−− |
| prove that i:Σ_(n=0) ^∞ (((−1 )^( n) )/((n +(1/2))cosh(n+(1/2))π)) =(π/4) ii: ∫_0 ^( 1) (( sin( π x ))/(x^( x) ( 1−x )^( 1−x) )) (dx/(1+x)) =(π/4) −−− |
| lim_( x→ 3) ( a ⌊x ⌋ + ⌊ −x⌋).tan(((πx)/2) )=−∞ a ∈ ? |
| 𝛗 =∫_0 ^( ∞) (( e^( −x^( 2) ) .ln( x ))/( (√x))) dx=λ Γ((1/4)) λ=? ■ |
| Ω = ∫ sin^( 2) (x).cos^( 4) (x ) dx |
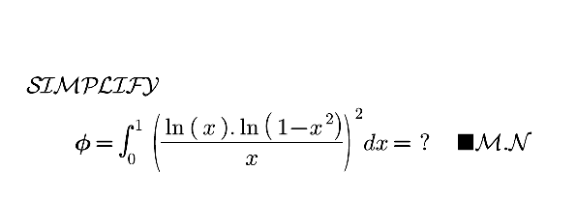
|