
Question and Answers Forum
DifferentiationQuestion and Answers: Page 13

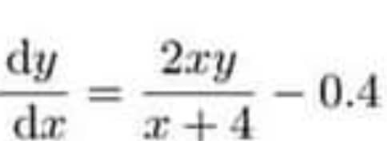
|
Question and Answers Forum |
DifferentiationQuestion and Answers: Page 13 |
| solve (d^2 y/dx^2 )−y=x^2 sin3x |
| (x+(√(x^2 +1)))(y+(√(y^2 +1)))=2021 ∀x,y∈R^+ . min (x+y)=? |
| Ω=∫_0 ^( 1) tan^( −1) (x).ln(x) = ? −−−−solution−−−− f(a)=∫_0 ^( 1) tan^( −1) ( x) .x^( a) dx = Σ_(n=1) ^∞ (( (−1)^( n−1) )/(2n−1)) ∫_0 ^( 1) x^( 2n+a−1) dx = Σ_(n=0) ^∞ (((−1)^( n−1) )/(2n−1)) ((1/(2n+ a)) ) Ω= f ′ (a )∣_(a=0) = Σ_(n=1) ^∞ (((−1)^n )/((2n−1)( 2n+ a)^( 2) )) Ω= f ′ (0 )=(1/4)Σ(( (−1 )^(n−1) (2n−1−2n ) )/(( 2n−1)n^( 2) )) =(1/4) Σ_(n=1) ^∞ (((−1)^(n−1) )/n^( 2) ) − Σ_(n=1) ^∞ (((−1)^( n−1) )/((2n−1)(2n))) = (π^( 2) /(48)) −{ Σ_(n=1) ^∞ {(((−1)^(n−1) )/(2n−1)) −(((−1)^( n−1) )/(2n))}} ∴ Ω = (π^( 2) /(48)) − (π/4) +(1/2) ln(2) |
| solve ⌊ x− (√(1−x^( 2) )) ⌋+⌊ x+ (√(1−x^( 2) )) ⌋=0 |
| Σ_(k=1) ^n (n/(n^2 +k)) (1/n)Σ_(k=1 ) ^n cos((1/( (√(n+k))))) convergente? |

|
| (2cosh(x)cos(y))dx+(sinh(x)sin(y))dy=0 |
| s>0 limΣ_(k=1) ^(2n) ( −1)^( k) .((( k)/(2n)) )^( s) = ? |
| ∫_0 ^( 1) (( ln^( 2) (1−x )ln(x))/x)dx=? |
| Ω:=∫_0 ^( ∞) (( x)/(( e^( x) +e^( −x) )^( 3) )) dx =? |
| prove that .. ∫_0 ^( ∞) (( x)/(cosh^( 3) (x ))) dx = G − (1/2) ■ G: catalan constant |
| a y=(√(x+(√(x+(√(x+.....)))))) b y=(√(x(√(x(√(x(√(x.....)))))))) find (dy/dx) |
| y = sin 8x cos 4x y^((n)) =? |
| Given the curve y=x^4 +3x^3 −6x^2 −3x determine for which value of α the tangent to the curve from point P(α,0) is maximum. |
| (1+bf(x))f′′(x)=(p/(λa)) solve this equation: find f(x) |
| Ω := ∫_0 ^( (π/4)) x.ln(sin(x))dx= ? |
| prove that : Φ = ∫_0 ^( ∞) (( sin^( 4) (x))/x^( 3) )dx= ln(2) −−−−−−−−− |
| y = sin^2 (2x) y^((n)) =? |
| calculate: I :=∫_0 ^( ∞) (((arctan(x))/x))^3 dx=? |
| prove that : 𝛗 := ∫_0 ^( ∞) (( sin((√x) ).sin((π/3) +(√x) ).sin(((2π)/3)+(√x) ).ln((1/x^( 2) ) ))/x)dx=^? π.(γ + ln(3) ) −−−−−−−−−− m.n |
| Ω= ∫_0 ^( ∞) (( sin^( 3) (x)ln(x))/x) dx=^(??) (π/8) (ln(3)−2γ) −−−−− solution.. Ω=∫_0^ ^( ∞) {(((3/4) sin(x)−(1/4) sin(3x))/x)} ln(x)dx = (3/4) (((−πγ)/2))− (1/4){ ∫_0 ^( ∞) ((sin(3x)ln(x))/x)dx=Ψ} ∴ Ψ := ∫_0 ^( ∞) (( sin(x).[ln(x)−ln(3)])/x)dx := −((πγ)/2) − ((ln(3).π)/2) ∴ Ω := ((−3πγ)/8) +((πγ)/8) +((π.ln(3))/8) := ((−2πγ)/8) + ((π.ln(3))/8) = (π/8) ( ln(3)−2γ ) |
| nice integral. prove that ∫^( ∞) _0 (( tan^( −1) (2x)+ tan^( −1) ((x/2) ))/(1+x^( 2) ))dx=(π^( 2) /4) |
| simplify ξ := Σ_(n=1) ^∞ ( (( 1)/(Σ_(k=1) ^n k^3 )) )=? |
| #calculate# Ω := ∫_0 ^( 1) ∫_0 ^( 1) (( x^( (t/2)) −x^( t) )/(1 − x)) dx dt = ? −−−m.n−−− |
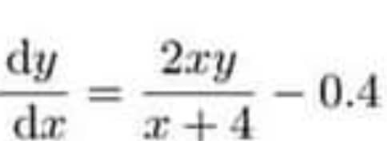
|

|