Question and Answers Forum

All Questions Topic List

DifferentiationQuestion and Answers: Page 18

Question Number 147411 Answers: 2 Comments: 0

Question Number 147355 Answers: 1 Comments: 0

Question Number 147346 Answers: 1 Comments: 0

Question Number 147262 Answers: 2 Comments: 0

...# Calculus #... I := ∫_0 ^( 1) Li_( 2) (x^( 2) ) dx = ?

$You can't use 'macro parameter character #' in math mode$ $I := \int_{0}^{1} {Li}_{2} (x^{2}) d x = ?$

Question Number 147209 Answers: 2 Comments: 1

Question Number 147135 Answers: 3 Comments: 0

Question Number 146924 Answers: 1 Comments: 0

Montrer que Σ_(k=0) ^(2n−1) cos^(2n) (𝛉+((k𝛑)/(2n)))= ((nC_(2n) ^n )/2^(2n−1) )

$Montrer que$ $\underset{k = 0}{\sum^{2 n - 1}} {c o s}^{2 n} (θ + \frac{k π}{2 n}) = \frac{{n C}_{2 n}^{n}}{2^{2 n - 1}}$

Question Number 146632 Answers: 0 Comments: 0

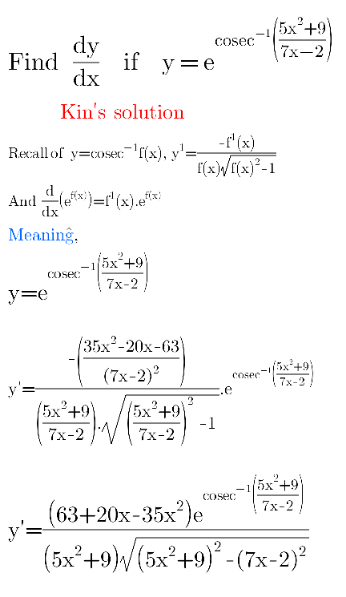
Question Number 146602 Answers: 1 Comments: 0

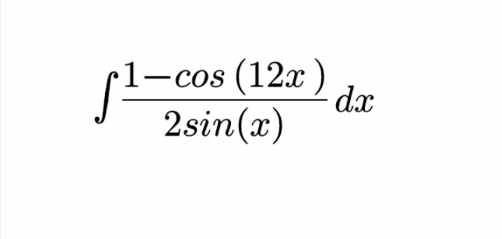
Question Number 146523 Answers: 2 Comments: 0

Question Number 146442 Answers: 2 Comments: 0

(d/dn)∣_(n=1) H_n =?

$\frac{d}{dn} ∣_{n = 1} H_{n} = ?$

Question Number 146300 Answers: 1 Comments: 0

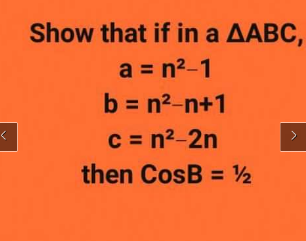
Question Number 146366 Answers: 1 Comments: 0

Question Number 146164 Answers: 1 Comments: 0

calulate :: S : = Σ_(n=1) ^∞ (( H_((n/2) ) )/( 2^( n) )) =? .......m.n.

$c a l u l a t e ::$ $S : = \underset{n = 1}{\sum^{\infty}} \frac{H_{\frac{n}{2}}}{2^{n}} = ?$ $. . . . . . . m . n .$

Question Number 146063 Answers: 1 Comments: 0

if g(x)=((x^( 2) −x)/(2x−1)) , D_g = [1 , ∞) , lim_(x→∞) ((g^( −1) (x))/(ax + b)) = b−a (a <0 ) then find the value of Max (b ) D_( g) = Domain

$i f g (x) = \frac{x^{2} - x}{2 x - 1}, D_{g} = [1, \infty)$ $, {l i m}_{x \to \infty} \frac{g^{- 1} (x)}{a x + b} = b - a (a < 0)$ $t h e n f i n d t h e v a l u e o f M a x (b)$ $D_{g} = D o m a i n$

Question Number 146054 Answers: 0 Comments: 0

I := ∫_0 ^( ∞) e^( −x) . J_(1/2) (x ) dx J_(v ) (x ) = x^( v) Σ_(n=0) ^( ∞) (((− 1 )^( n) x^( 2n) )/(2^( n + v) n ! Γ ( n + v +1 ))) ....

$I := \int_{0}^{\infty} e^{- x} . J_{\frac{1}{2}} (x) d x$ $J_{v} (x) = x^{v} \underset{n = 0}{\sum^{\infty}} \frac{{(- 1)}^{n} x^{2 n}}{2^{n + v} n! Γ (n + v + 1)}$ $. . . .$

Question Number 145701 Answers: 2 Comments: 1

Question Number 145620 Answers: 1 Comments: 0

(d/dx)(((x+((x+((x+...))^(1/3) ))^(1/3) ))^(1/3) )=?

$\frac{d}{d x} (\sqrt[3]{x + \sqrt[3]{x + \sqrt[3]{x + . . .}}}) = ?$

Question Number 145602 Answers: 5 Comments: 0

.....Advanced .........Calculus..... Q:: Find the value of :: determinant ((( i :: 𝛗 := ∫_0 ^( 1) Ln ( Γ ( 2 + x ) )dx = ? )),(( ii :: Ω := Σ_(n=1) ^∞ (( 1)/( n ( 2n + 3 ))) = ?))) .....m.n.july.1970..... ■

$. . . . . Advanced . . . . . . . . . Calculus . . . . .$ $Q :: Find the value of ::$ $\begin{array}{cc} i :: ϕ := \int_{0}^{1} Ln (Γ (2 + x)) d x = ? \\ i i :: Ω := \underset{n = 1}{\sum^{\infty}} \frac{1}{n (2 n + 3)} = ? \end{array}$ $. . . . . m . n . j u l y . 1970 . . . . . ◼$

Question Number 145450 Answers: 2 Comments: 0

Question Number 145449 Answers: 1 Comments: 0

Question Number 145399 Answers: 1 Comments: 0

Prove that lim_(n→+∞) ∫^( n) _( 0) (t^n /(n!)) e^(−t) dt = (1/2)

$Prove that$ $\lim_{n \to + \infty} {\int_{0}}^{n} \frac{t^{n}}{n!} e^{- t} dt = \frac{1}{2}$

Question Number 145164 Answers: 3 Comments: 0

Question Number 145163 Answers: 2 Comments: 0

Question Number 145021 Answers: 2 Comments: 0

Question Number 144995 Answers: 1 Comments: 0

Find the maximum distance between two points on the curve (x^4 /a^4 ) + (y^4 /b^4 ) = 1 .

$Find the maximum distance$ $between two points on the$ $curve \frac{x^{4}}{a^{4}} + \frac{y^{4}}{b^{4}} = 1 .$

Pg 13 Pg 14 Pg 15 Pg 16 Pg 17 Pg 18 Pg 19 Pg 20 Pg 21 Pg 22

Contact: info@tinkutara.com