
Question and Answers Forum
DifferentiationQuestion and Answers: Page 8





|
Question and Answers Forum |
DifferentiationQuestion and Answers: Page 8 |
|
| Max and min P=(√2) x+ (√3) y subject to constraint (x^2 /9) +(y^2 /(25)) ≤ 1 ≤ x^2 +y^2 |
| if y=bcoslog((x/n))^n , then (dy/dx)=? |
| solve: ∫(((√(x^2 +1)) −(√(x^2 −1)))/( (√(x^4 −1))))dx |
| ∫ (((x^(−6) −64)/(4+2x^(−1) +x^(−2) )).(x^2 /(4−4x^(−1) +x^(−2) )) − ((4x^2 (2x+1))/(1−2x)))dx |
| The function f(x)=ax^2 +bx+c has gradient function 4x+2 and stationary value 1. Find the values of a,b and c. |
| The curve for which (dy/dx)=a(x−p)(x−q), where a, p and q are constants, has turning points at (2,0) and (1,1). i) state the value of p and q. ii) using these values, determine the value of a |
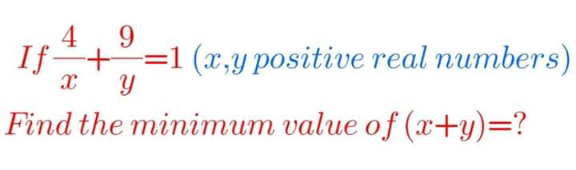
|
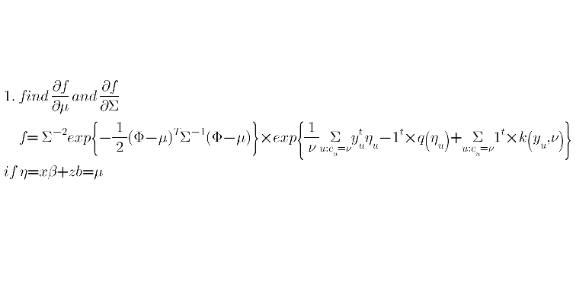
|
| f(x)= { ((2x+3 ;x>0)),((3x−5 ;x≤0)) :} ((df(x))/dx)=? |
| The tangent to the curve y=ax^2 +bx+2 at (1,(1/2)) is parallel to the normal to the curve y=x^2 +6x+10 at (−2,2). Find the values of a and b. |
| Find the coordinates of the point on the curve y=(x/(1+x)) at which the tangents to the curve are parallel to the line x−y+8=0. Find the equations of the tangents at these points. |
| find the drivative of f(x,y,z)=cos(xy)+e^(zy) +ln(zy) at point (1,0,(1/2)) in the direction v=i+2j+2k |

|

|
| show that the padel equation of the curve x = acos𝛉 −acos 2𝛉 , y = 2asin 𝛉 −asin 2𝛉 is 9(r^2 −a^2 ) = 8p^2 |

|
| prove Σ_(n=1) ^∞ (( 1)/( F_n )) < 4 F_n : fibonacci sequence |
| Find min value f(x)= (x+4)(x+5)(x+6)(x+7) |
| Given f(x)=x(√(1−x+(√(1−x)))) where 0≤x≤1 find max f(x) |
| Given f(x)=(√(sin x)) +(√(3 cos x)) x∈ (0, (π/2)) Find max f(x). |
| solve the D.E 2dx−e^(y−x) dy=0 |
| convert this D.E to exact D.E and solve it ydx+x(1+y)dy=0 |
| solve the D.E. y^′ =tan(x+y)−1 |

|

|