
Question and Answers Forum
Question Number 38284 by NECx last updated on 23/Jun/18

Commented by MrW3 last updated on 23/Jun/18
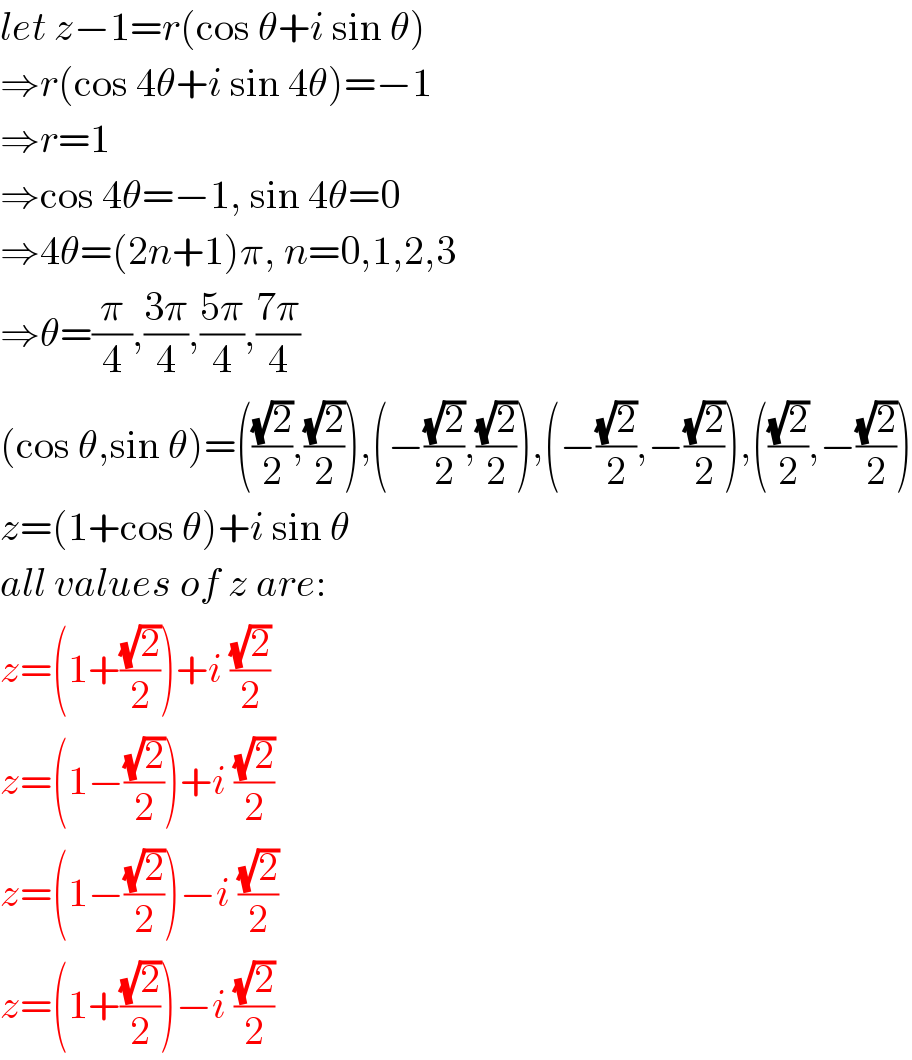
Commented by abdo.msup.com last updated on 23/Jun/18
![let put z−1=x (e)⇔x^4 =e^(iπ) if x=r e^(iθ) we get r^4 e^(i4θ) =e^(i(2k+1)π) ⇒r=1 and 4θ=(2k+1)π ⇒θ_k =(((2k+1)π)/4) with k∈[[0,3]] and x_k =e^(i(((2k+1)π)/4)) ⇒z_k =x_k +1= 1+e^(i(((2k+1)π)/4)) ⇒ z_0 =1+e^(i(π/4)) z_1 =1+e^(i((3π)/4)) z_2 =1+e^(i((5π)/4)) z_3 =1+e^(i((7π)/4))](Q38313.png)
Answered by tanmay.chaudhury50@gmail.com last updated on 23/Jun/18

| ||
Question and Answers Forum | ||
Question Number 38284 by NECx last updated on 23/Jun/18 | ||
 | ||
Commented by MrW3 last updated on 23/Jun/18 | ||
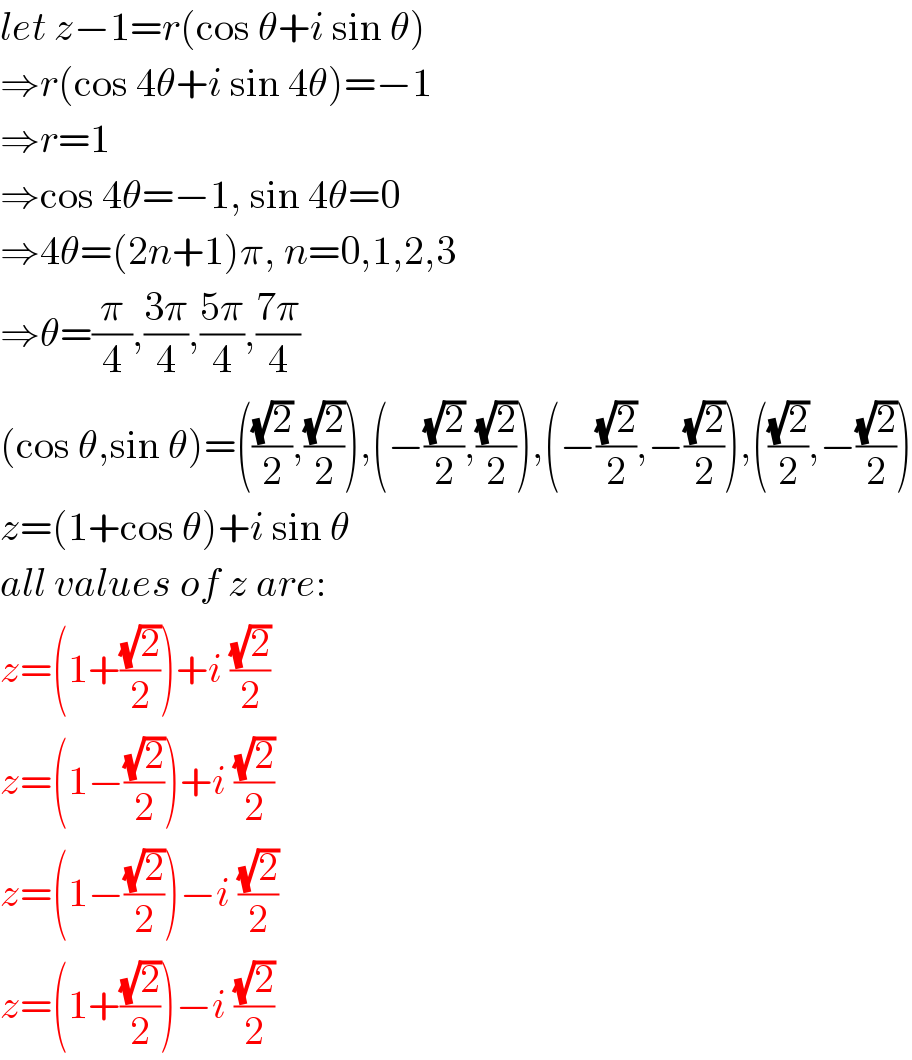 | ||
Commented by abdo.msup.com last updated on 23/Jun/18 | ||
![let put z−1=x (e)⇔x^4 =e^(iπ) if x=r e^(iθ) we get r^4 e^(i4θ) =e^(i(2k+1)π) ⇒r=1 and 4θ=(2k+1)π ⇒θ_k =(((2k+1)π)/4) with k∈[[0,3]] and x_k =e^(i(((2k+1)π)/4)) ⇒z_k =x_k +1= 1+e^(i(((2k+1)π)/4)) ⇒ z_0 =1+e^(i(π/4)) z_1 =1+e^(i((3π)/4)) z_2 =1+e^(i((5π)/4)) z_3 =1+e^(i((7π)/4))](Q38313.png) | ||
Answered by tanmay.chaudhury50@gmail.com last updated on 23/Jun/18 | ||
 | ||
| ||