Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 7887 by tawakalitu last updated on 23/Sep/16

$$Findthefirstfourtermsofthepowerseries$$$$expansionof\frac{sinx}{1-x}$$

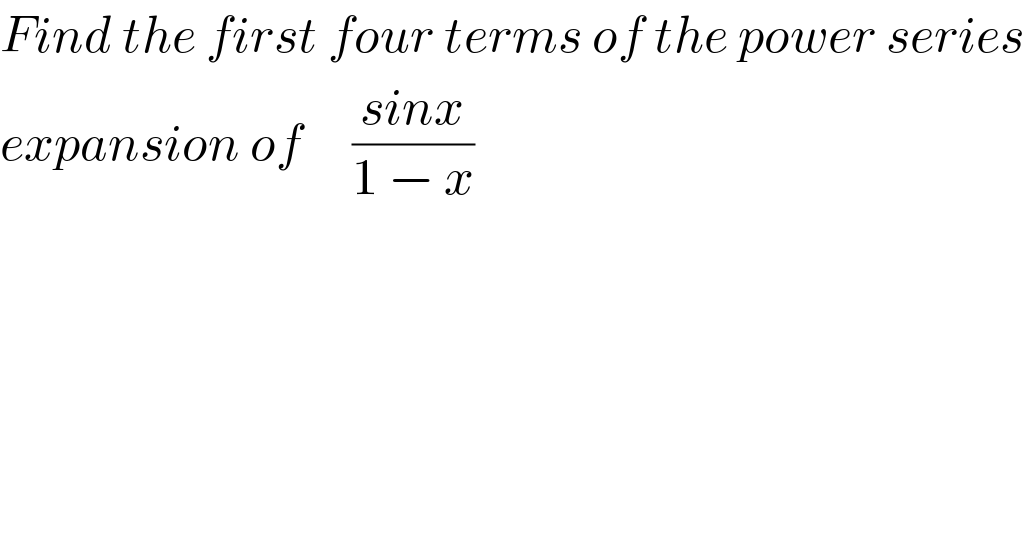
Answered by sandy_suhendra last updated on 23/Sep/16

$$fromthepowerseries:$$$$sinx=\underset{n=0}{\sum ^{\mathrm{\infty }}}{(-1)}^n\frac{x^{2n+1}}{(2n+1)!}$$$$=x-\frac{x^3}{3!}+\frac{x^5}{5!}-\frac{x^7}{7!}\left(thefirstfourterm\right)$$$$\frac{1}{1-x}=\underset{n=0}{\sum ^{\mathrm{\infty }}}{x}^n$$$$=1+x+x^2+x^3\left(thefirstfourterm\right)$$$$so\frac{sinx}{1-x}=1\left(x\right)+x(-\frac{x^3}{3!})+x^2\left(\frac{x^5}{5!}\right)+x^3(-\frac{x^7}{7!})$$$$=x-\frac{x^4}{3!}+\frac{x^7}{5!}-\frac{x^{10}}{7!}$$

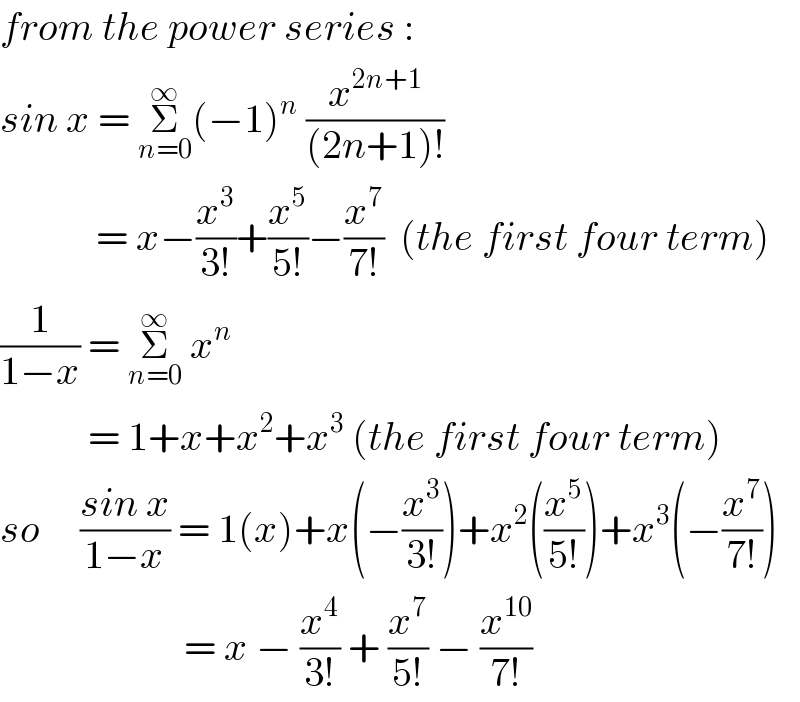
Commented by tawakalitu last updated on 23/Sep/16

$$Thankyousomuch.$$

Commented by Rasheed Soomro last updated on 24/Sep/16

$$(x-\frac{x^3}{3!}+\frac{x^5}{5!}-\frac{x^7}{7!})(1+x+x^2+x^3)=x-\frac{x^4}{3!}+\frac{x^7}{5!}-\frac{x^{10}}{7!}???$$

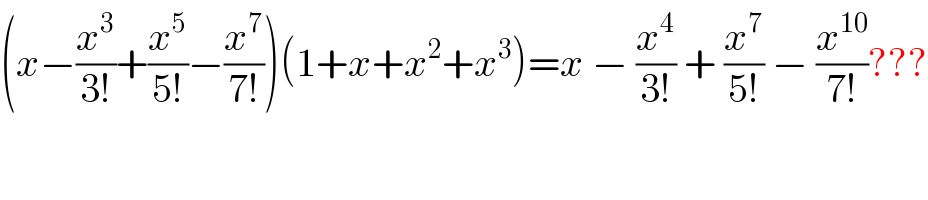
Commented by prakash jain last updated on 24/Sep/16

$$(x-\frac{x^3}{6}+...)(1+x+x^2+x^3+..)$$$$=x+x^2+x^3+x^4-\frac{x^3}{6}-\frac{x^4}{6}+\left(\mathrm{higher}\mathrm{order}\right)$$$$=x+x^2-\frac{5x^3}{6}-\frac{5x^4}{6}$$

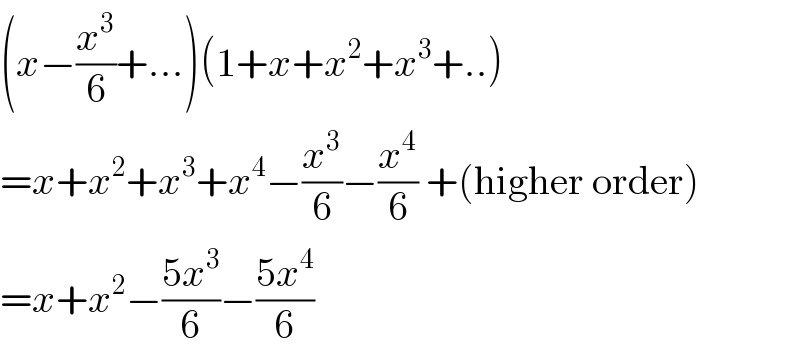
Commented by sandy_suhendra last updated on 28/Sep/16

$$Ithought$$$$\frac{sinx}{1-x}=sinx\left(\frac{1}{1-x}\right)$$$$=\underset{n=0}{\sum ^{\mathrm{\infty }}}{(-1)}^n\frac{x^{2n+1}}{(2n+1)!}\left[\underset{n=0}{\sum ^{\mathrm{\infty }}}{x}^n\right]$$$$=\underset{n=0}{\sum ^{\mathrm{\infty }}}{(-1)}^n\frac{x^{2n+1}}{(2n+1)!}{x}^n$$$$but{I}^{\prime }mwrong,{thank}^{\prime }sforyourcorrection$$

Commented by sandy_suhendra last updated on 28/Sep/16

$${thank}^{\prime }sforyourcorrection$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com