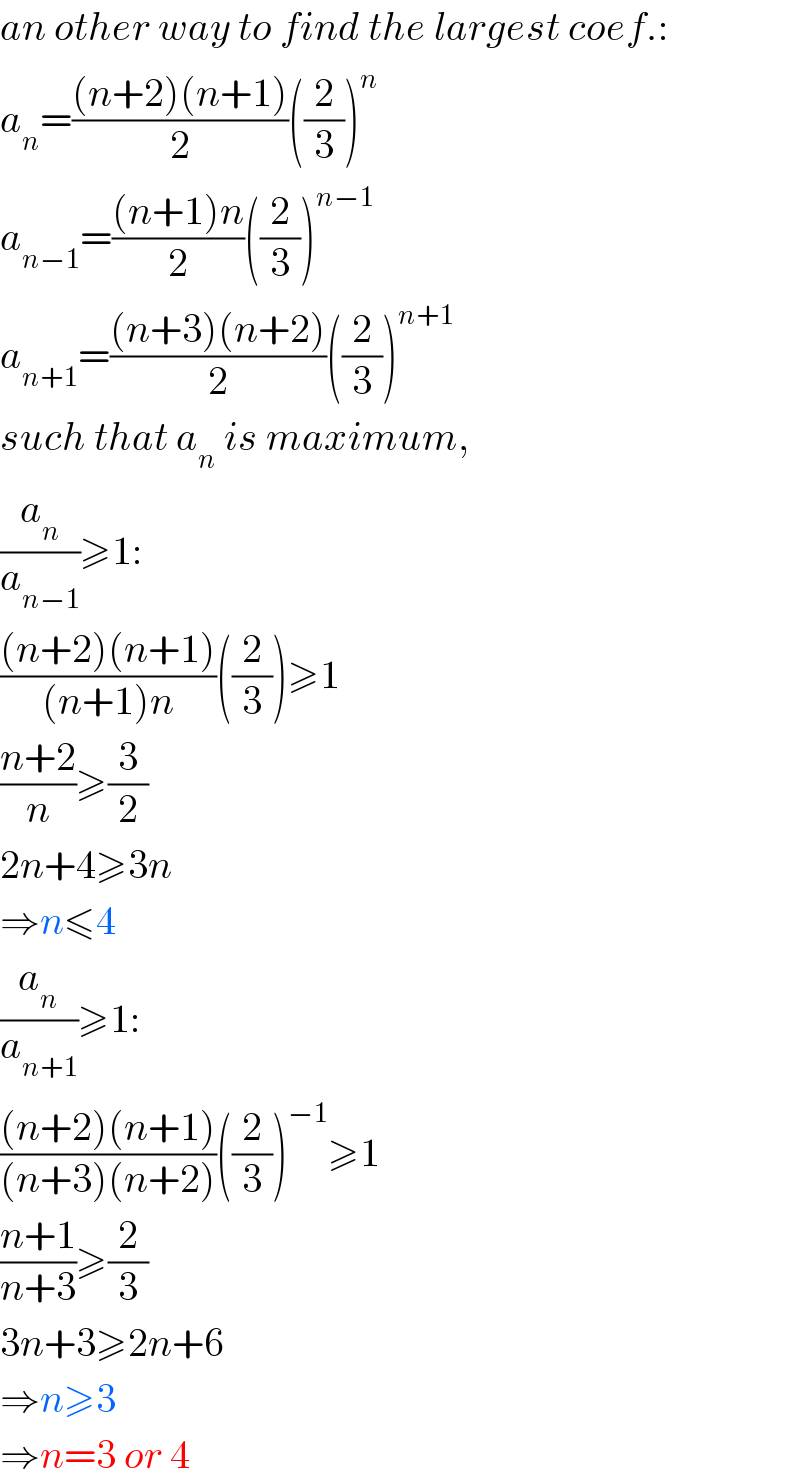Find the greatest coefficient in the expansion of (6 − 4x)^(−3)


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 62945 by Tawa1 last updated on 27/Jun/19

$Find the greatest coefficient in the expansion of {(6 - 4 x)}^{- 3}$
	Answered by mr W last updated on 27/Jun/19

	${(6 - 4 x)}^{- 3}$ $= 6^{- 3} {(1 - \frac{2 x}{3})}^{- 3}$ $= 6^{- 3} \underset{n = 0}{\sum^{\infty}} C_{2}^{n + 2} {(\frac{2}{3})}^{n} x^{n}$ $= 6^{- 3} \underset{n = 0}{\sum^{\infty}} a_{n} x^{n}$ $a_{n} = C_{2}^{n + 2} {(\frac{2}{3})}^{n} = \frac{(n + 2) (n + 1)}{2} {(\frac{2}{3})}^{n}$ $a (x) = \frac{(x + 2) (x + 1)}{2} {(\frac{2}{3})}^{x}$ $a (x) h a s m a x i m u m a t x = 3.48$ $\Rightarrow a_{n} h a s m a x i m u m a t n = 3 o r 4$ $a_{3} = \frac{5 \times 4}{2} \times {(\frac{2}{3})}^{3} = \frac{80}{27}$ $a_{4} = \frac{6 \times 5}{2} \times {(\frac{2}{3})}^{4} = \frac{80}{27}$ $\Rightarrow x^{3} a n d x^{4} t e r m h a s t h e l a r g e s t c o e f .$ $w h i c h i s 6^{- 3} \times \frac{80}{27} = \frac{10}{729} .$
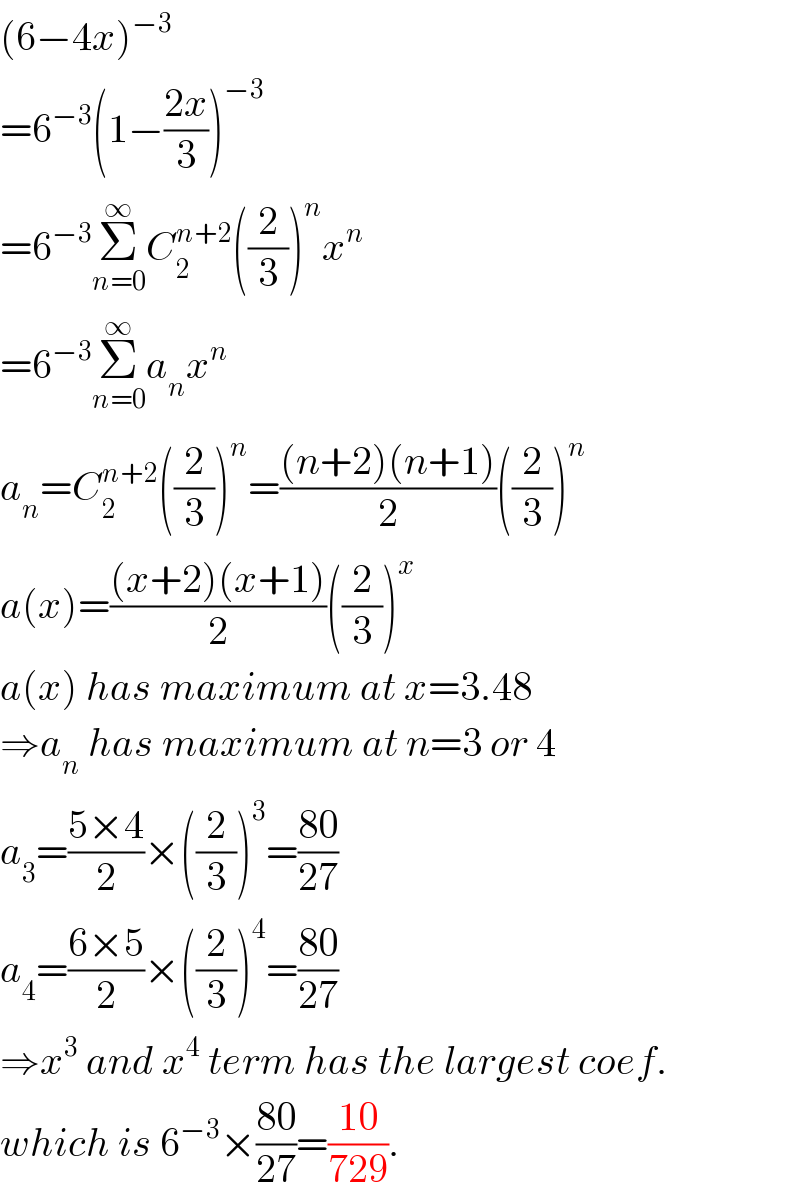
	Commented by Tawa1 last updated on 27/Jun/19

	$God bless you sir$
	Commented by Tawa1 last updated on 29/Jun/19

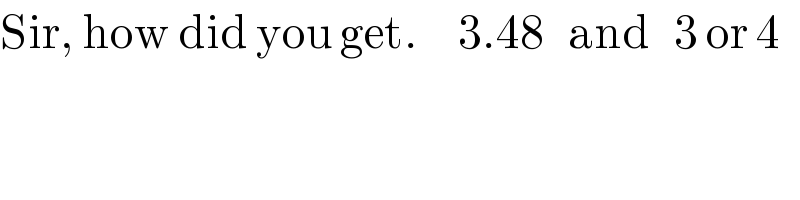
	$Sir, how did you get . 3.48 and 3 or 4$
	Commented by mr W last updated on 29/Jun/19

	$l o o k a t a (x) = \frac{(x + 2) (x + 1)}{2} {(\frac{2}{3})}^{x}$ $(x + 2) (x + 1) i s i n c r e a s i n g w i t h x,$ ${(\frac{2}{3})}^{x} i s d e c r e a s i n g w i t h x,$ $s o a n (x) h a s a m a x i m u m a t s o m e$ $p o i n t, a t t h i s p o i n t \frac{d a (x)}{d x} = 0 .$ $\frac{d a (x)}{d x} = \frac{(2 x + 3)}{2} {(\frac{2}{3})}^{x} + \frac{(x^{2} + 3 x + 1)}{2} {(\frac{2}{3})}^{x} \ln (\frac{2}{3}) = 0$ $(2 x + 3) + (x^{2} + 3 x + 1) \ln (\frac{2}{3}) = 0$ $\ln (\frac{2}{3}) x^{2} + [3 \ln (\frac{2}{3}) - 2] x + [\ln (\frac{2}{3}) - 3] = 0$ $\Rightarrow x = \frac{- 3 \ln (\frac{2}{3}) + 2 + \sqrt{{[3 \ln (\frac{2}{3}) - 2]}^{2} - 4 \ln (\frac{2}{3}) [\ln (\frac{2}{3}) - 3]}}{2 \ln (\frac{2}{3})} = 3.48$ $i . e . a (x) h a s m a x i m u m a t x = 3.48$ $a_{n} w i l l h a v e m a x i m u m w h e n n i s$ $s o c l o s e t o 3.48 a s p o s s i b l e, t h a t i s$ $n = 3 o r n = 4 .$
	Commented by Tawa1 last updated on 29/Jun/19

	$God bless you sir$
	Commented by mr W last updated on 13/Jul/19

	$a n o t h e r w a y t o f i n d t h e l a r g e s t c o e f . :$ $a_{n} = \frac{(n + 2) (n + 1)}{2} {(\frac{2}{3})}^{n}$ $a_{n - 1} = \frac{(n + 1) n}{2} {(\frac{2}{3})}^{n - 1}$ $a_{n + 1} = \frac{(n + 3) (n + 2)}{2} {(\frac{2}{3})}^{n + 1}$ $s u c h t h a t a_{n} i s m a x i m u m,$ $\frac{a_{n}}{a_{n - 1}} ⩾ 1 :$ $\frac{(n + 2) (n + 1)}{(n + 1) n} (\frac{2}{3}) ⩾ 1$ $\frac{n + 2}{n} ⩾ \frac{3}{2}$ $2 n + 4 ⩾ 3 n$ $\Rightarrow n ⩽ 4$ $\frac{a_{n}}{a_{n + 1}} ⩾ 1 :$ $\frac{(n + 2) (n + 1)}{(n + 3) (n + 2)} {(\frac{2}{3})}^{- 1} ⩾ 1$ $\frac{n + 1}{n + 3} ⩾ \frac{2}{3}$ $3 n + 3 ⩾ 2 n + 6$ $\Rightarrow n ⩾ 3$ $\Rightarrow n = 3 o r 4$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum