Find the shortest distance between the lines L = (1, 4, 2) + N(1, 3, 2) and r = (−1, 1, −1) + λ(1, 2, −1)


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 56697 by Tawa1 last updated on 21/Mar/19

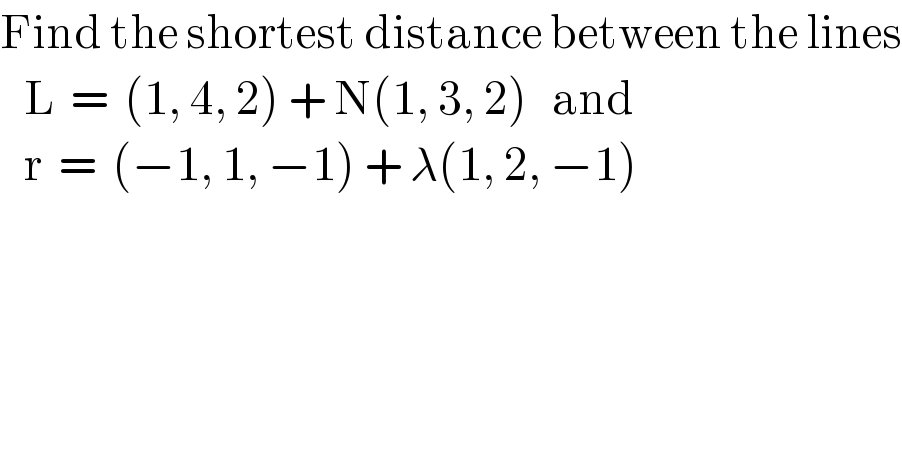
$Find the shortest distance between the lines$ $L = (1, 4, 2) + N (1, 3, 2) and$ $r = (- 1, 1, - 1) + λ (1, 2, - 1)$
	Answered by mr W last updated on 21/Mar/19

	$m a n y m e t h o d s t o s o l v e .$ $d^{2} = {[1 + N - (- 1 + λ)]}^{2} + {[4 + 3 N - (1 + 2 λ)]}^{2} + {[2 + 2 N - (- 1 - λ)]}^{2}$ $d^{2} = {(2 + N - λ)}^{2} + {(3 + 3 N - 2 λ)}^{2} + {(3 + 2 N + λ)}^{2} . . . (i i i)$ $\frac{\partial (d^{2})}{\partial N} = 2 (2 + N - λ) + 2 \times 3 (3 + 3 N - 2 λ) + 2 \times 2 (3 + 2 N + λ) = 0$ $\Rightarrow 14 N - 5 λ + 17 = 0 . . . (i)$ $\frac{\partial (d^{2})}{\partial λ} = - 2 (2 + N - λ) - 2 \times 2 (3 + 3 N - 2 λ) + 2 (3 + 2 N + λ) = 0$ $\Rightarrow 5 N - 6 λ + 5 = 0 . . . (i i)$ $s o l v e (i) a n d (i i) :$ $\Rightarrow N = - \frac{77}{59}$ $\Rightarrow λ = - \frac{15}{59}$ $p u t t h i s i n t o (i i i) :$ $d_{m i n}^{2} = {(\frac{56}{59})}^{2} + {(\frac{- 24}{59})}^{2} + {(\frac{8}{59})}^{2}$ $\Rightarrow d_{m i n} = \frac{\sqrt{56^{2} + 24^{2} + 8^{2}}}{59} = \frac{8}{\sqrt{59}} = 1.0415$
	Commented by Tawa1 last updated on 22/Mar/19

	$Wow, God bless you sir .$
	Answered by mr W last updated on 23/Mar/19

	$v e c t o r m e t h o d$ $n o r m a l t o L a n d r :$ $(1, 3, 2) \times (1, 2, - 1) = (- 7, 3, - 1)$ $(1 + 1, 4 - 1, 2 + 1) = (2, 3, 3)$ $(2, 3, 3) ∙ (- 7, 3, - 1) = - 14 + 9 - 3 = - 8$ $d = \frac{∣ - 8 ∣}{\sqrt{{(- 7)}^{2} + 3^{2} + {(- 1)}^{2}}} = \frac{8}{\sqrt{59}} \approx 1.0415$
	Commented by Tawa1 last updated on 24/Mar/19

	$God bless you sir . I appreciate .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum