
Question and Answers Forum
GeometryQuestion and Answers: Page 51
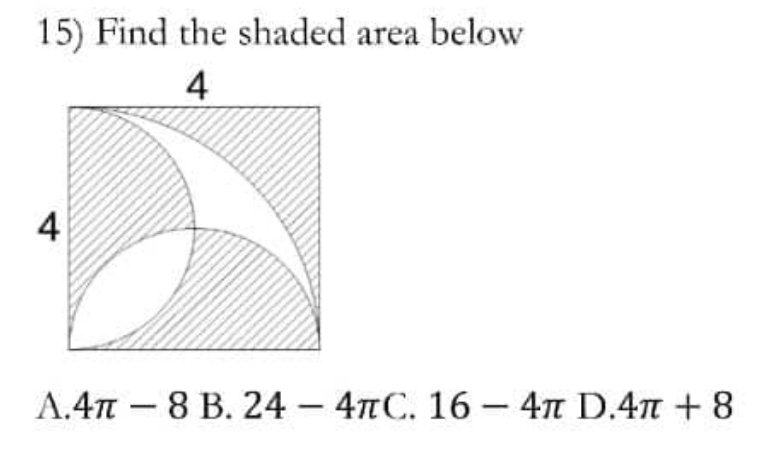
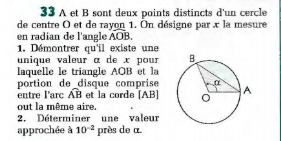



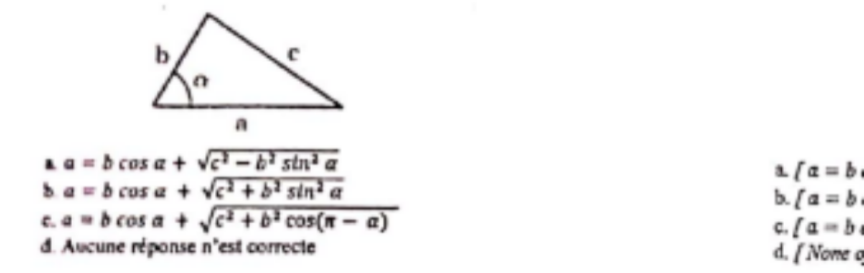







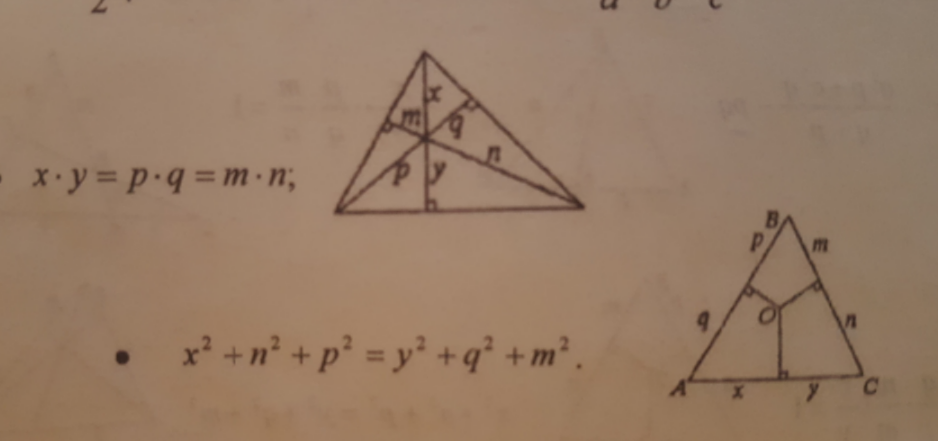



|
Question and Answers Forum |
GeometryQuestion and Answers: Page 51 |

|
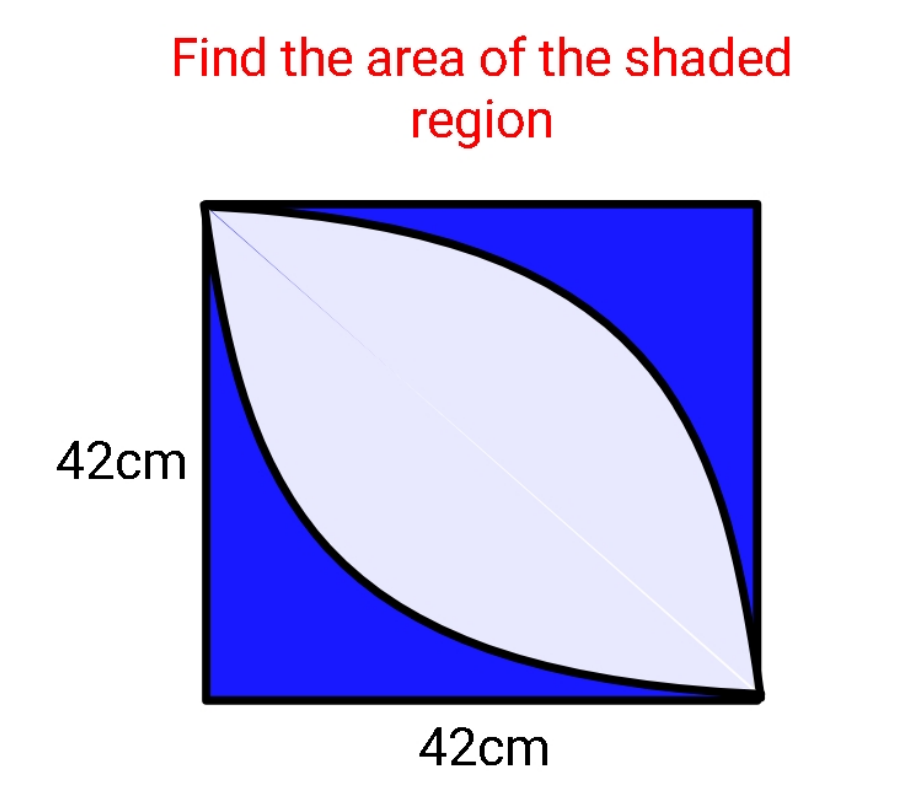
| S=(4/3)(√(m(m−m_a )(m−m_b )(m−m_c ))) m=((m_a +m_b +m_c )/2) m_a ;m_b ;m_c −mediani prove |

|

|

|

|

|

|

|

|

|

|

|

|
| (R/r)=(5/2) ⇒ a:b:c=3:4:5 prove R,r− radius h_a :h_b :h_c =(1/a):(1/b):(1/c)=bc:ac:ab prove |
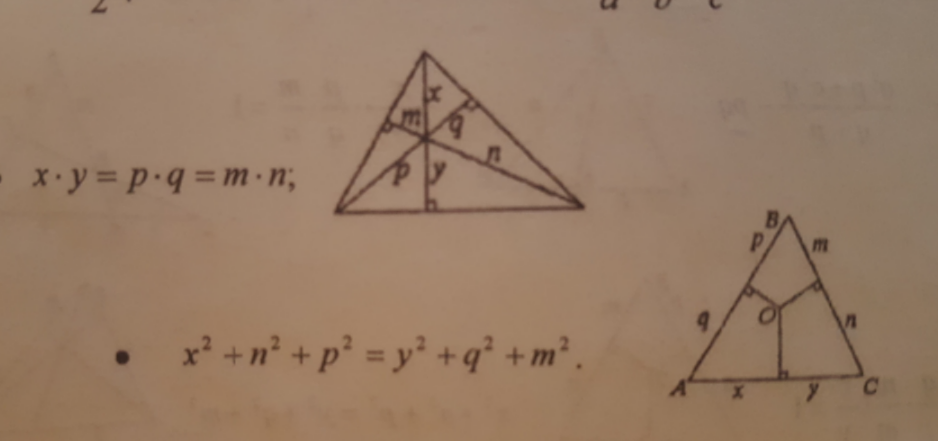
|

|

|
| prove that equation of a circle passing through the points of intersection of a circle S=0 and a line L=0 can be taken as S+λL=0 where λ is a parameter |
| if the radius of a circle touching parabola y^2 =4x at (4,4)and having directrix of y^2 =4x as its normal is r, then find [r]? (where [x] denote greatest integer lessthan or equal to x) |
| a parabola y=x^2 −15x+36 cuts the x axis at P and Q. a circle is drawn through P and Q so that the origin is outside it. then find the length of tangent to the circle from (0,0)? |

|
| Solid cube ABCD.EFGH is cut by a plane X so that it forms a plane of slices IJKLMN where I is mid AB, J is mid BF K is mid FG, L is mid HG, M mid DH and N mid AD. If the edge of the cube is X, find the area of the IJKLMN field |
| Cube ABCD.EFGH has side length a. Point P lies on AC such that AP : PC = 3 : 1 Through P a line l parallel to BD is drawn such that l each intersect BC at X and CD at Y. If AC and BD intersect at O find the distance between XY with OG! |
| in a triangle ABC we have { ((3sinA^ +4cosB^ =6)),((4sinB^ +3cosA^ =1)) :} find C^ |

|