Given { ((((4x^2 )/(4x^2 +1)) = y)),((((4y^2 )/(4y^2 +1)) = z)),((((4z^2 )/(4z^2 +1)) = x)) :} . find x+y+z ?


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 105448 by bemath last updated on 29/Jul/20
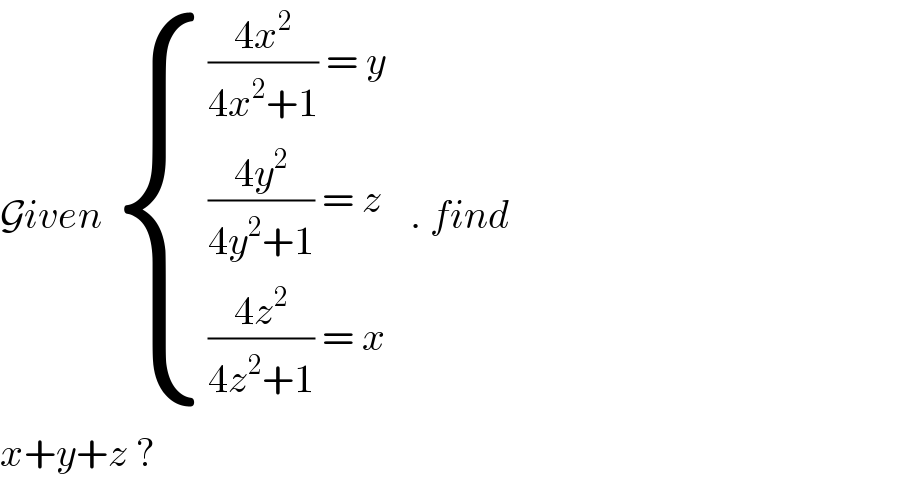
$Given { ((((4x^2 )/(4x^2 +1)) = y)),((((4y^2 )/(4y^2 +1)) = z)),((((4z^2 )/(4z^2 +1)) = x)) :} . find x+y+z ?$
$G i v e n {\begin{cases} \frac{4 x^{2}}{4 x^{2} + 1} = y \\ \frac{4 y^{2}}{4 y^{2} + 1} = z \\ \frac{4 z^{2}}{4 z^{2} + 1} = x \end{cases} . f i n d$ $x + y + z ?$
Commented by john santu last updated on 29/Jul/20
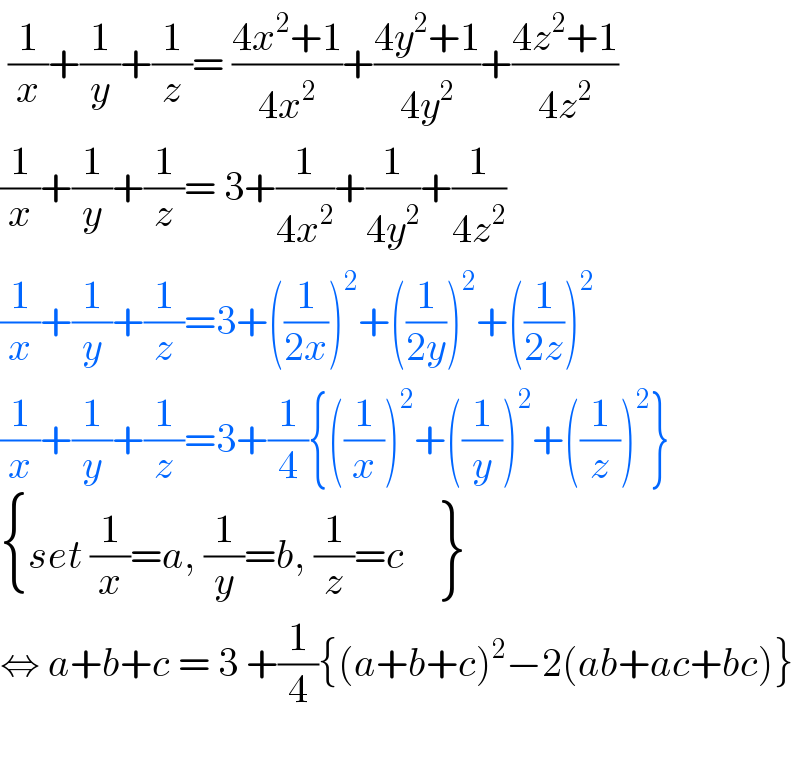
$(1/x)+(1/y)+(1/z)= ((4x^2 +1)/(4x^2 ))+((4y^2 +1)/(4y^2 ))+((4z^2 +1)/(4z^2 )) (1/x)+(1/y)+(1/z)= 3+(1/(4x^2 ))+(1/(4y^2 ))+(1/(4z^2 )) (1/x)+(1/y)+(1/z)=3+((1/(2x)))^2 +((1/(2y)))^2 +((1/(2z)))^2 (1/x)+(1/y)+(1/z)=3+(1/4){((1/x))^2 +((1/y))^2 +((1/z))^2 } { ((set (1/x)=a, (1/y)=b, (1/z)=c {: (),() })) :} ⇔ a+b+c = 3 +(1/4){(a+b+c)^2 −2(ab+ac+bc)}$
$\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = \frac{4 x^{2} + 1}{4 x^{2}} + \frac{4 y^{2} + 1}{4 y^{2}} + \frac{4 z^{2} + 1}{4 z^{2}}$ $\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = 3 + \frac{1}{4 x^{2}} + \frac{1}{4 y^{2}} + \frac{1}{4 z^{2}}$ $\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = 3 + {(\frac{1}{2 x})}^{2} + {(\frac{1}{2 y})}^{2} + {(\frac{1}{2 z})}^{2}$ $\frac{1}{x} + \frac{1}{y} + \frac{1}{z} = 3 + \frac{1}{4} {{(\frac{1}{x})}^{2} + {(\frac{1}{y})}^{2} + {(\frac{1}{z})}^{2}}$ ${\begin{cases} s e t \frac{1}{x} = a, \frac{1}{y} = b, \frac{1}{z} = c \begin{array}{c} \end{array}} \end{cases}$ $\Leftrightarrow a + b + c = 3 + \frac{1}{4} {{(a + b + c)}^{2} - 2 (a b + a c + b c)}$
Commented by behi83417@gmail.com last updated on 29/Jul/20
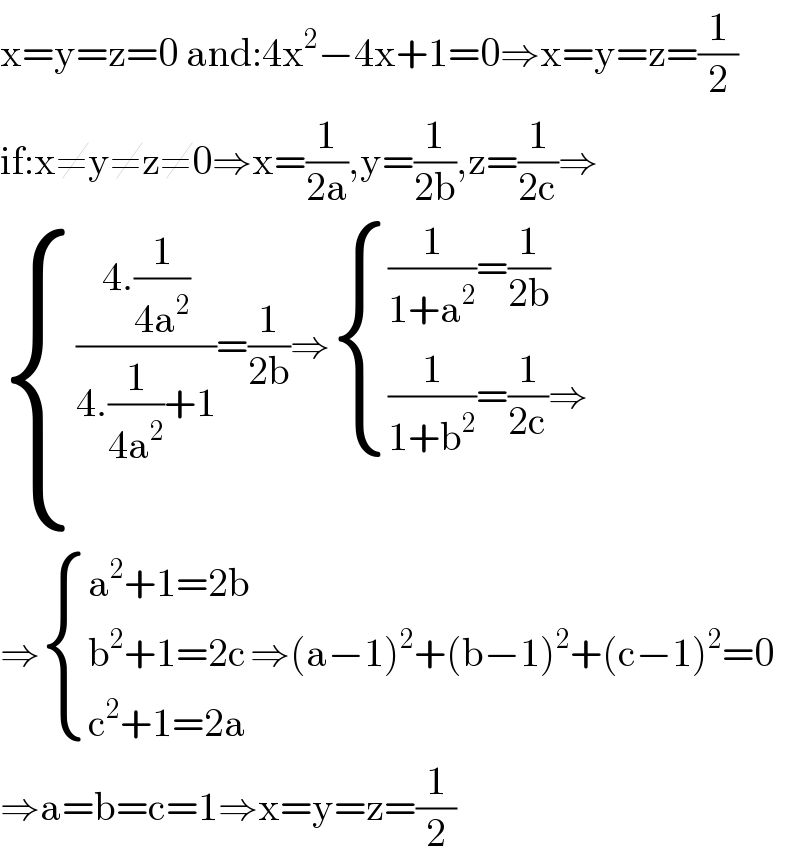
$x=y=z=0 and:4x^2 −4x+1=0⇒x=y=z=(1/2) if:x≠y≠z≠0⇒x=(1/(2a)),y=(1/(2b)),z=(1/(2c))⇒ { ((((4.(1/(4a^2 )))/(4.(1/(4a^2 ))+1))=(1/(2b))⇒ { (((1/(1+a^2 ))=(1/(2b)))),(((1/(1+b^2 ))=(1/(2c))⇒)) :})),() :} ⇒ { ((a^2 +1=2b)),((b^2 +1=2c)),((c^2 +1=2a)) :}⇒(a−1)^2 +(b−1)^2 +(c−1)^2 =0 ⇒a=b=c=1⇒x=y=z=(1/2)$
$x = y = z = 0 and : {4 x}^{2} - 4 x + 1 = 0 \Rightarrow x = y = z = \frac{1}{2}$ $if : x \neq y \neq z \neq 0 \Rightarrow x = \frac{1}{2 a}, y = \frac{1}{2 b}, z = \frac{1}{2 c} \Rightarrow$ ${\begin{cases} \frac{4 . \frac{1}{{4 a}^{2}}}{4 . \frac{1}{{4 a}^{2}} + 1} = \frac{1}{2 b} \Rightarrow {\begin{cases} \frac{1}{1 + a^{2}} = \frac{1}{2 b} \\ \frac{1}{1 + b^{2}} = \frac{1}{2 c} \Rightarrow \end{cases} \end{cases}$ $\Rightarrow {\begin{cases} a^{2} + 1 = 2 b \\ b^{2} + 1 = 2 c \\ c^{2} + 1 = 2 a \end{cases} \Rightarrow {(a - 1)}^{2} + {(b - 1)}^{2} + {(c - 1)}^{2} = 0$ $\Rightarrow a = b = c = 1 \Rightarrow x = y = z = \frac{1}{2}$
	Answered by ajfour last updated on 29/Jul/20

	$l e t \frac{1}{x} = p, \frac{1}{y} = q, \frac{1}{z} = r$ $\Rightarrow \frac{4}{4 - p^{2}} = \frac{1}{q}, \frac{4}{4 {(\frac{q}{p})}^{2} + q^{2}} = \frac{1}{r},$ $\frac{4}{4 + r^{2}} = \frac{1}{p}$ $\Rightarrow 4 - p^{2} = 4 q . . . (i)$ $4 {(\frac{q}{p})}^{2} + q^{2} = 4 r \Rightarrow q^{2} (\frac{1}{p^{2}} + \frac{1}{4}) = r . . (i i)$ $4 + r^{2} = 4 p . . . (i i i)$ $\Rightarrow {(1 - \frac{p^{2}}{4})}^{2} {(\frac{1}{p^{2}} + \frac{1}{4})}^{2} = 4 (p - 1)$ $\Rightarrow {(16 - p^{4})}^{2} = 64 \times 16 p^{4} (p - 1)$ $. . . . . . d e g r e e 8$
	Answered by bramlex last updated on 29/Jul/20
	$(1/y) = ((4x^2 +1)/(4x^2 )) →(1/y) = 1+(1/(4x^2 )) →(1/z) = 1+(1/4)(1+(1/(4x^2 )))^2 →1+(1/(4z^2 )) = (1/x) →1+(1/4){1+(1/4)(1+(1/(4x^2 )))^2 }= (1/x) set (1/x) = m 1+(1/4){1+(1/4)(1+(1/4)m^2 )^2 } = m (5/4)+(1/4)(1+(1/2)m^2 +(1/(16))m^4 )=m 20+4+2m^2 +(1/4)m^4 =16m m^4 +32m^2 −256m+16×24=0$
	$\frac{1}{y} = \frac{4 x^{2} + 1}{4 x^{2}} \to \frac{1}{y} = 1 + \frac{1}{4 x^{2}}$ $\to \frac{1}{z} = 1 + \frac{1}{4} {(1 + \frac{1}{4 x^{2}})}^{2}$ $\to 1 + \frac{1}{4 z^{2}} = \frac{1}{x}$ $\to 1 + \frac{1}{4} {1 + \frac{1}{4} {(1 + \frac{1}{4 x^{2}})}^{2}} = \frac{1}{x}$ $s e t \frac{1}{x} = m$ $1 + \frac{1}{4} {1 + \frac{1}{4} {(1 + \frac{1}{4} m^{2})}^{2}} = m$ $\frac{5}{4} + \frac{1}{4} (1 + \frac{1}{2} m^{2} + \frac{1}{16} m^{4}) = m$ $20 + 4 + 2 m^{2} + \frac{1}{4} m^{4} = 16 m$ $m^{4} + 32 m^{2} - 256 m + 16 \times 24 = 0$
	Answered by 1549442205PVT last updated on 07/Aug/20
	$WLOG suppose that x≥y≥z≥0 (1).Then ((4z^2 )/(4z^2 +1))≥((4x^2 )/(4x^2 +1))≥((4y^2 )/(4y^2 +1))≥0 ⇒(z^2 /(4z^2 +1))≥(x^2 /(4x^2 +1))⇒z^2 (4x^2 +1)≥x^2 (4z^2 +1) ⇒z^2 ≥x^2 ⇒z≥x≥0(2).From (1) and(2) we get x=y=z.Hence ((4x^2 )/(4x^2 +1))=x⇔4x^3 −4x^2 +x=0 ⇔x(4x^2 −4x+1)=0.⇔x(2x−1)^2 =0 ⇔x∈{0;(1/2)} Thus,the roots of the given system are: {(0;0);((1/2);(1/2))}$
	$WLOG suppose that x ⩾ y ⩾ z ⩾ 0 (1) . Then$ $\frac{{4 z}^{2}}{{4 z}^{2} + 1} ⩾ \frac{{4 x}^{2}}{{4 x}^{2} + 1} ⩾ \frac{{4 y}^{2}}{{4 y}^{2} + 1} ⩾ 0$ $\Rightarrow \frac{z^{2}}{{4 z}^{2} + 1} ⩾ \frac{x^{2}}{{4 x}^{2} + 1} \Rightarrow z^{2} ({4 x}^{2} + 1) ⩾ x^{2} ({4 z}^{2} + 1)$ $\Rightarrow z^{2} ⩾ x^{2} \Rightarrow z ⩾ x ⩾ 0 (2) . From (1) and (2)$ $we get x = y = z . Hence$ $\frac{{4 x}^{2}}{{4 x}^{2} + 1} = x \Leftrightarrow {4 x}^{3} - {4 x}^{2} + x = 0$ $\Leftrightarrow x ({4 x}^{2} - 4 x + 1) = 0 . \Leftrightarrow x {(2 x - 1)}^{2} = 0$ $\Leftrightarrow x \in {0; \frac{1}{2}}$ $Thus, the roots of the given system are :$ ${(0; 0); (\frac{1}{2}; \frac{1}{2})}$
	Commented by behi83417@gmail.com last updated on 31/Jul/20

	$You can't use 'macro parameter character #' in math mode$
	Commented by 1549442205PVT last updated on 01/Aug/20

	$Thank Sir . I understanded and shall$ $correct it$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum