
Question and Answers Forum
Question Number 156426 by ZiYangLee last updated on 11/Oct/21
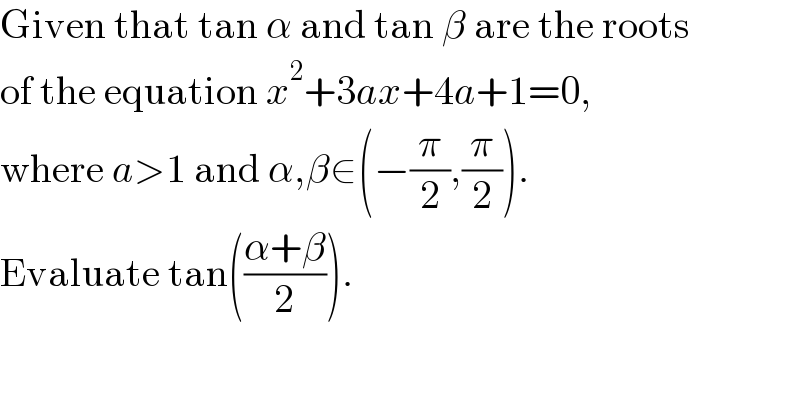
Answered by mr W last updated on 11/Oct/21

| ||
Question and Answers Forum | ||
Question Number 156426 by ZiYangLee last updated on 11/Oct/21 | ||
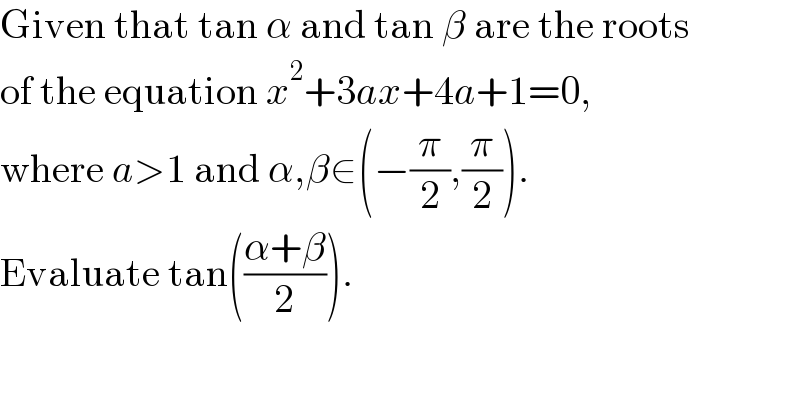 | ||
Answered by mr W last updated on 11/Oct/21 | ||
 | ||
| ||