Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

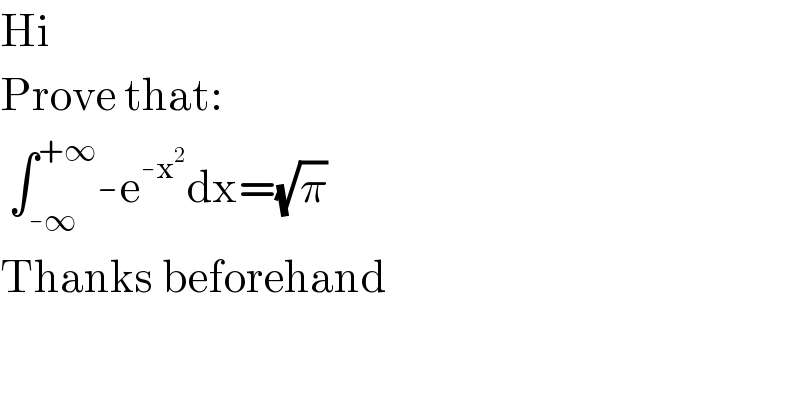
Question Number 116806 by Backer last updated on 07/Oct/20

$$\mathrm{Hi}$$$$\mathrm{Prove}\mathrm{that}:$$$${\int }_{-\mathrm{\infty }}^{+\mathrm{\infty }}-{\mathrm{e}}^{-{\mathrm{x}}^2}\mathrm{dx}=\sqrt{\pi }$$$$\mathrm{Thanks}\mathrm{beforehand}$$$$$$

Answered by Bird last updated on 07/Oct/20

$$let{A}_{\xi }=\int {\int }_{]-\xi ,\xi [{}^2}{e}^{-x^2-y^2}dxdy$$$$wehsve{lim}_{\xi \to +\mathrm{\infty }}{A}_{\xi }={\left({\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx\right)}^2$$$$letusethediffeomorphism$$$$\{\begin{array}{l}x=rcos\theta \\ y=rsin\theta wehave-\xi ⩽x⩽\xi \end{array}$$$$and-\xi ⩽y⩽\xi \Rightarrow 0⩽x^2+y^2⩽2{\xi }^2$$$$\Rightarrow 0⩽r⩽\xi \sqrt{2}\Rightarrow$$$$A_{\xi }={\int }_0^{\xi \sqrt{2}}r{e}^{-r^2}dr{\int }_{-\pi }^{\pi }d\theta$$$$=2\pi {[-\frac{1}{2}{e}^{-r^2}]}_0^{\xi \sqrt{2}}$$$$=\pi (1-e^{-2{\xi }^2})\Rightarrow {lim}_{\xi \to +\mathrm{\infty }}{A}_{\xi }=\pi$$$$={\left({\int }_{-\mathrm{\infty }}^{+\mathrm{\infty }}{e}^{-x^2}dx\right)}^{2}but{\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}{e}^{-x^2}dx>0$$$$\Rightarrow {\int }_{-\mathrm{\infty }}^{+\mathrm{\infty }}{e}^{-x^2}dx=\sqrt{\pi }$$

Answered by bobhans last updated on 07/Oct/20

$$\mathrm{I}=\underset{-\mathrm{\infty }}{\stackrel{\mathrm{\infty }}{\int }}{\mathrm{e}}^{-{\mathrm{x}}^2}\mathrm{dx}\Rightarrow {\mathrm{I}}^2=\underset{-\mathrm{\infty }}{\stackrel{\mathrm{\infty }}{\int }}\underset{-\mathrm{\infty }}{\stackrel{\mathrm{\infty }}{\int }}{\mathrm{e}}^{-{\mathrm{x}}^2-{\mathrm{y}}^2}\mathrm{dx}\mathrm{dy}$$$${\mathrm{I}}^2=\underset{0}{\stackrel{\mathrm{\infty }}{\int }}\underset{0}{\stackrel{2\pi }{\int }}\mathrm{r}{\mathrm{e}}^{-{\mathrm{r}}^2}\mathrm{d}\theta \mathrm{dr}=\underset{0}{\stackrel{\mathrm{\infty }}{\int }}\mid \left({\mathrm{re}}^{-{\mathrm{r}}^2}\left(\theta \right)\right){\mid }_0^{2\pi })\mathrm{dr}$$$${\mathrm{I}}^2=2\pi \underset{0}{\stackrel{\mathrm{\infty }}{\int }}{\mathrm{re}}^{-{\mathrm{r}}^2}\mathrm{dr}=\pi \underset{0}{\stackrel{\mathrm{\infty }}{\int }}{\mathrm{e}}^{-{\mathrm{r}}^2}\mathrm{d}\left({\mathrm{r}}^2\right)$$$${\mathrm{I}}^2=-\pi \mid \left({\mathrm{e}}^{-{\mathrm{r}}^2}\right){\mid }_0^{\mathrm{\infty }}=-\pi (0-1)=\pi$$$$\mathrm{Hence}\mathrm{I}=\sqrt{\pi }$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com