How many ordered pairs (m,n) are there for 1≤m,n≤100 such that 7^m +7^n is divisble by 5?


Question and Answers Forum

All Questions Topic List
Number Theory Questions
Previous in All Question Next in All Question
Previous in Number Theory Next in Number Theory
Question Number 2878 by Yozzi last updated on 29/Nov/15

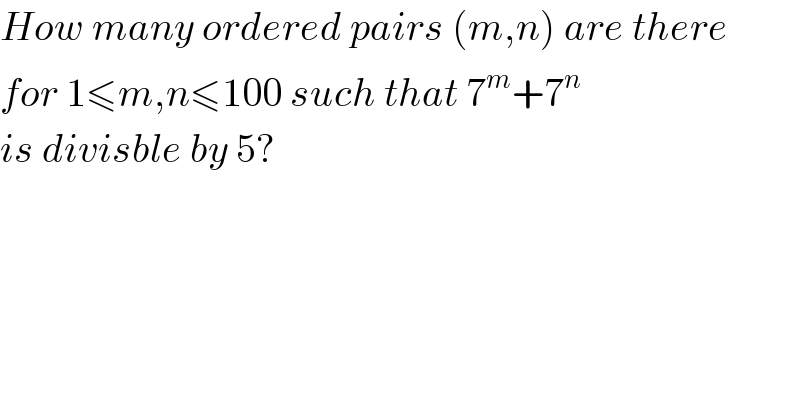
$H o w m a n y o r d e r e d p a i r s (m, n) a r e t h e r e$ $f o r 1 ⩽ m, n ⩽ 100 s u c h t h a t 7^{m} + 7^{n}$ $i s d i v i s b l e b y 5 ?$
Commented by prakash jain last updated on 29/Nov/15

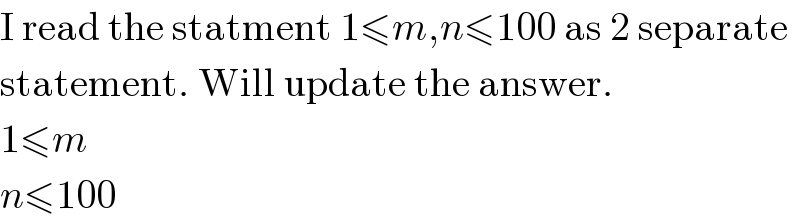
$I read the statment 1 ⩽ m, n ⩽ 100 as 2 separate$ $statement . Will update the answer .$ $1 ⩽ m$ $n ⩽ 100$
	Answered by prakash jain last updated on 29/Nov/15
	$7^m +7^n ≡0 (mod 5) 2^m +2^n ≡0 (mod 5) k∈{0,1,2,3} k=4j⇒2^k ≡1 (mod 5) k=4j+1⇒2^k ≡2 (mod 5) k=4j+2⇒2^k ≡4 (mod 5) k=4j+3⇒2^k ≡3 (mod 5) Solution for m and n j,l∈{0,1,2,3} m=4j, n=4l+2 m=4j+1, n=4l+3 m=4j+2, n=4l m=4j+3, n=4l+1 1≤n≤100, 1≤m≤100 n=4l 1≤l≤25 m=4j+2 0≤j≤24 n=4l+1 0≤l≤24 m=4j+3, 0≤j≤24 n=4l+2 0≤l≤24 m=4j 1≤j≤25 n=4l+3 0≤l≤24 m=4j+1 0≤j≤24 Total number of pairs=4×25^2 =625×4=2500$
	$7^{m} + 7^{n} \equiv 0 (\mod 5)$ $2^{m} + 2^{n} \equiv 0 (\mod 5)$ $k \in {0, 1, 2, 3}$ $k = 4 j \Rightarrow 2^{k} \equiv 1 (\mod 5)$ $k = 4 j + 1 \Rightarrow 2^{k} \equiv 2 (\mod 5)$ $k = 4 j + 2 \Rightarrow 2^{k} \equiv 4 (\mod 5)$ $k = 4 j + 3 \Rightarrow 2^{k} \equiv 3 (\mod 5)$ $Solution for m and n$ $j, l \in {0, 1, 2, 3}$ $m = 4 j, n = 4 l + 2$ $m = 4 j + 1, n = 4 l + 3$ $m = 4 j + 2, n = 4 l$ $m = 4 j + 3, n = 4 l + 1$ $1 ⩽ n ⩽ 100, 1 ⩽ m ⩽ 100$ $n = 4 l 1 ⩽ l ⩽ 25$ $m = 4 j + 2 0 ⩽ j ⩽ 24$ $n = 4 l + 1 0 ⩽ l ⩽ 24$ $m = 4 j + 3, 0 ⩽ j ⩽ 24$ $n = 4 l + 2 0 ⩽ l ⩽ 24$ $m = 4 j 1 ⩽ j ⩽ 25$ $n = 4 l + 3 0 ⩽ l ⩽ 24$ $m = 4 j + 1 0 ⩽ j ⩽ 24$ $Total number of pairs = 4 \times 25^{2} = 625 \times 4 = 2500$
	Answered by Rasheed Soomro last updated on 30/Nov/15

	$L e t 7^{m} \equiv r (m o d 5)$ $E x p e r i m e n t i n g a n d O b s e r v i n g f o r v a l u e s o f m a n d r :$ $7^{(0, 1, 2, 3, 4, 5, 6, . . .)} \equiv (1, 2, 4, 3, 1, 2, 4, . . .) (m o d 5)$ $G e n e r a l i z i n g :$ $7^{4 k} \equiv 1 (m o d 5) ∥ 7^{4 h + 2} \equiv 4 (m o d 5)$ $7^{4 k + 1} \equiv 2 (m o d 5) ∥ 7^{4 h + 3} \equiv 3 (m o d 5)$ $7^{4 k + 2} \equiv 4 (m o d 5) ∥ 7^{4 h} \equiv 1 (m o d 5)$ $7^{4 k + 3} \equiv 3 (m o d 5) ∥ 7^{4 h + 1} \equiv 2 (m o d 5)$ $A d d i n g c o r r e s p o n d i n g$ $7^{4 k} + 7^{4 h + 2} \equiv 0 (m o d 5)$ $7^{4 k + 1} + 7^{4 h + 3} \equiv 0 (m o d 5)$ $7^{4 k + 2} + 7^{4 h} \equiv 0 (m o d 5)$ $7^{4 k + 3} + 7^{4 h + 1} \equiv 0 (m o d 5)$ $F o u r t y p e s o f o r d e r e d p a i r s [(m, n)] s a t i s f y i n g t h e$ $g i v e n s t a t e m e n t .$ $1 ⩽ (4 k, 4 h + 2) ⩽ 100$ $1 ⩽ (4 k + 1, 4 h + 3) ⩽ 100$ $1 ⩽ (4 k + 2, 4 h) ⩽ 100$ $1 ⩽ (4 k + 3, 4 h + 1) ⩽ 100$ $N o w {i t}^{'} s e a s y t o c o u n t$ $S u p p o s e t h e r e a r e x 4 k + 1 t y p e n u m b e r s b e t w e e n 1 a n d$ $100 i n c l u s i v e a n d y 4 h + 3 t y p e n u m b e r s b e t w e e n 1 a n d$ $100 i n c l u s i v e THEN$ $T h e r e a r e x y o r d e r e d p a i r s o f s u c h n u m b e r s .$ $A m u l t i p l i c a t i o n a l t a b l e i s e a s y w a y o f r e c o r d i n g a n d$ $c o u n t i n g a l l r e q u i r e d {(m, n)}^{'} s$
	Commented by prakash jain last updated on 29/Nov/15

	$Thanks .$ $I thought$ $1 ⩽ m$ $n ⩽ 100$
	Commented by Rasheed Soomro last updated on 29/Nov/15

	$I t^{'} s n o t m i s r e a d i n g . A c t u a l l y t h e s t a t e m e n t c a n a l s o b e$ $r e a d i n t h a t w a y .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum