
Question and Answers Forum
Question Number 90135 by Ar Brandon last updated on 21/Apr/20
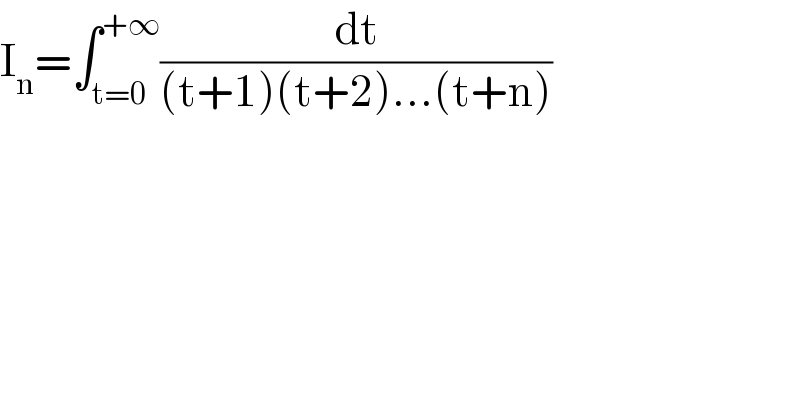
Answered by TANMAY PANACEA. last updated on 21/Apr/20
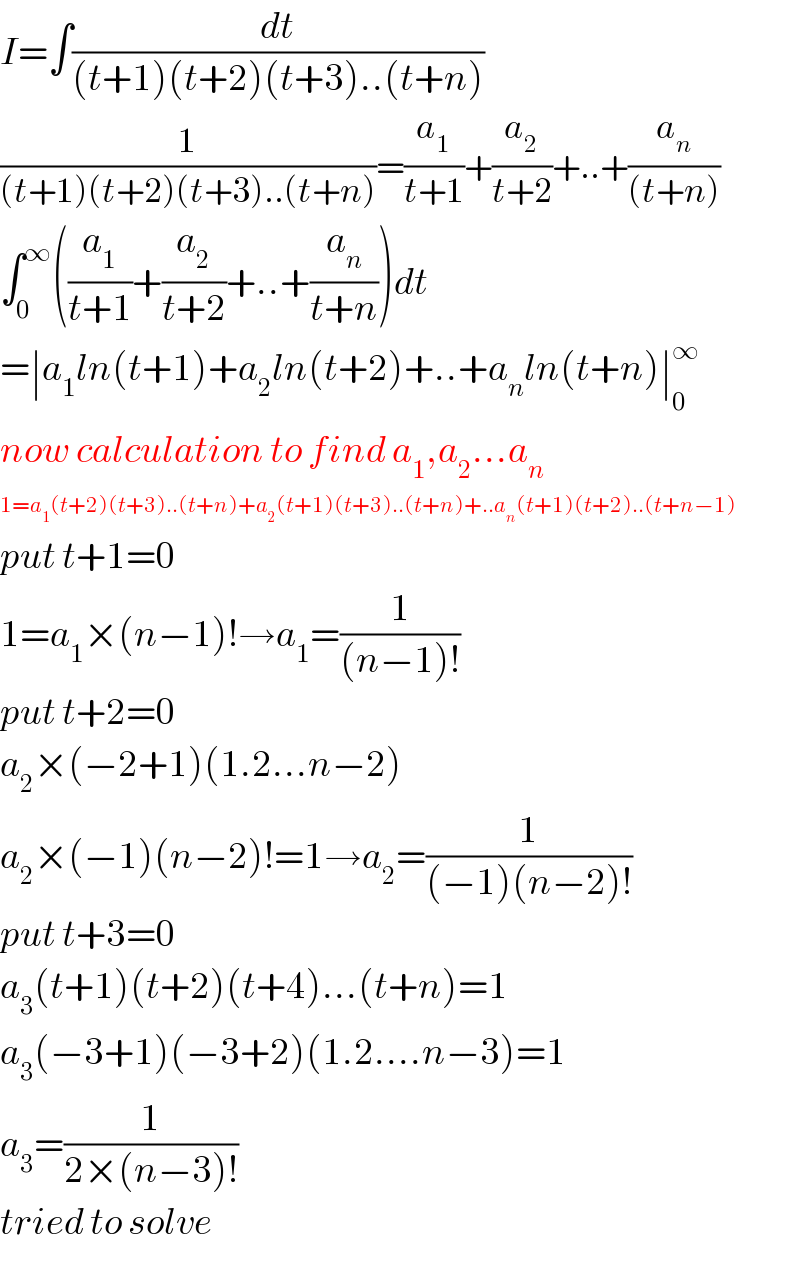
| ||
Question and Answers Forum | ||
Question Number 90135 by Ar Brandon last updated on 21/Apr/20 | ||
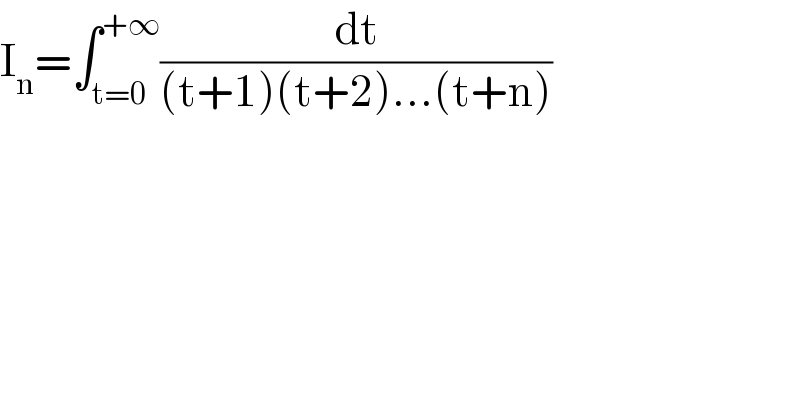 | ||
Answered by TANMAY PANACEA. last updated on 21/Apr/20 | ||
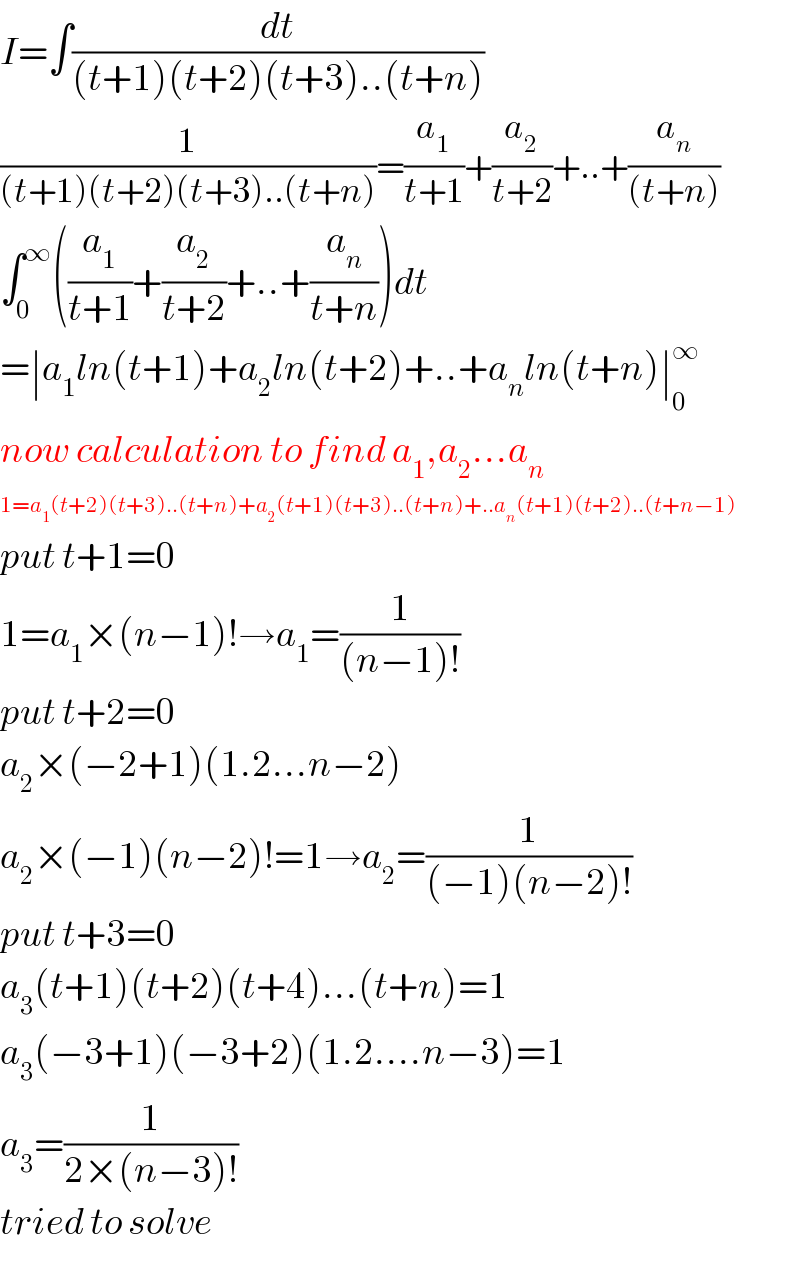 | ||
| ||