
Question and Answers Forum
Question Number 100675 by bobhans last updated on 28/Jun/20

Commented by bramlex last updated on 28/Jun/20
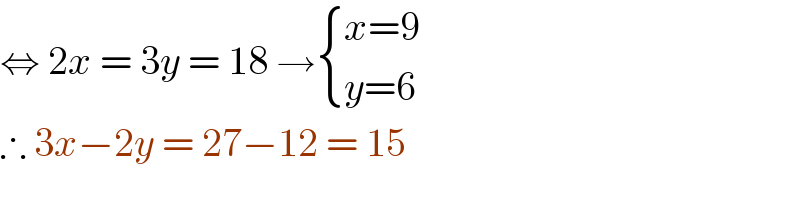
Answered by 1549442205 last updated on 28/Jun/20
![log_(2x) ((1/(18)))=log_(2x) (1)−log_(2x) 18=−log_(2x) 18 =log_(3y) ((1/(2x)))=log_(3y) 1−log_(3y) (2x)=−log_(3y) (2x) log_(18) ((1/(3y)))=log_(18) 1−log_(18) (3y)=−log_(18) (3y) ,so from the hypothesis we get: log_(2x) 18=log_(3y) (2x)=log_(18) (3y)=a.So { (((2x)^a =18(1))),(((3y)^a =2x(2) (∗))),((18^a =3y (3))) :} From (2)we get (2x)^a =[(3y)^a ]^a =(3y)^a^2 (4) From (3) we get (3y)^a^2 =(18^a )^a^2 =18^a^3 (5) From(4) ,(5) we get (2x)^a =18^a^3 (6) From (1) and (6) we obtain 18=18^a^3 ⇒a^3 =1⇔a=1.Replace into (∗) we get { ((3y=2x)),((2x=18)),((18=3y)) :} ⇔ { ((x=9)),((y=6)) :} Therefore,3x−2y=3×9−2×6=15](Q100719.png)
| ||
Question and Answers Forum | ||
Question Number 100675 by bobhans last updated on 28/Jun/20 | ||
 | ||
Commented by bramlex last updated on 28/Jun/20 | ||
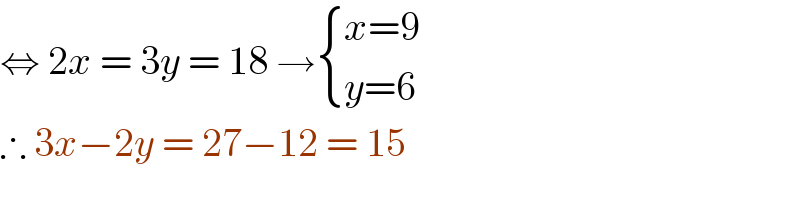 | ||
Answered by 1549442205 last updated on 28/Jun/20 | ||
![log_(2x) ((1/(18)))=log_(2x) (1)−log_(2x) 18=−log_(2x) 18 =log_(3y) ((1/(2x)))=log_(3y) 1−log_(3y) (2x)=−log_(3y) (2x) log_(18) ((1/(3y)))=log_(18) 1−log_(18) (3y)=−log_(18) (3y) ,so from the hypothesis we get: log_(2x) 18=log_(3y) (2x)=log_(18) (3y)=a.So { (((2x)^a =18(1))),(((3y)^a =2x(2) (∗))),((18^a =3y (3))) :} From (2)we get (2x)^a =[(3y)^a ]^a =(3y)^a^2 (4) From (3) we get (3y)^a^2 =(18^a )^a^2 =18^a^3 (5) From(4) ,(5) we get (2x)^a =18^a^3 (6) From (1) and (6) we obtain 18=18^a^3 ⇒a^3 =1⇔a=1.Replace into (∗) we get { ((3y=2x)),((2x=18)),((18=3y)) :} ⇔ { ((x=9)),((y=6)) :} Therefore,3x−2y=3×9−2×6=15](Q100719.png) | ||
| ||