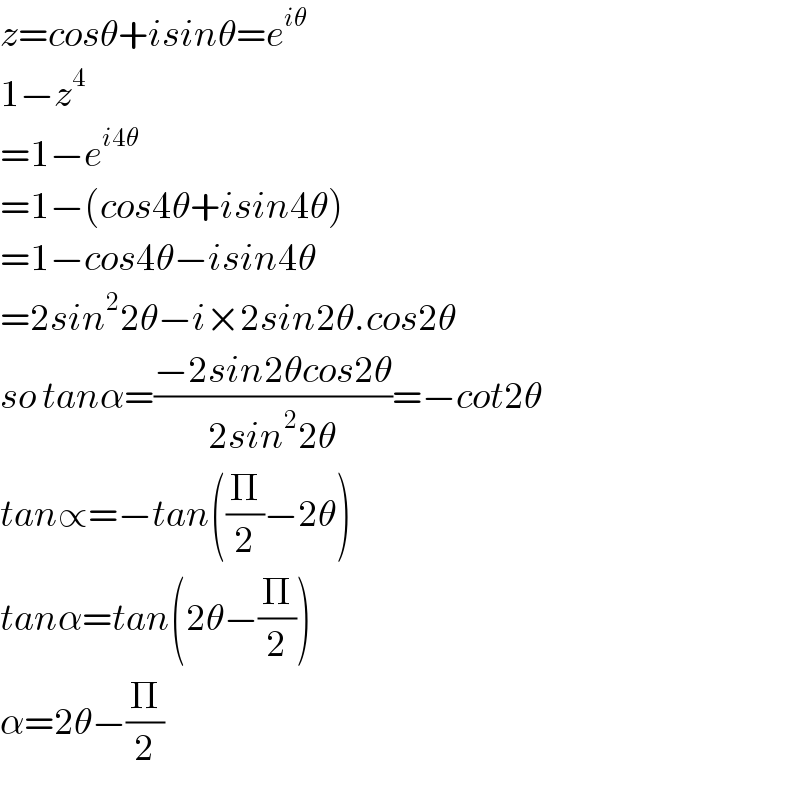Question and Answers Forum
Question Number 43363 by rahul 19 last updated on 10/Sep/18
Commented by maxmathsup by imad last updated on 10/Sep/18
![we have z =e^(iθ) ⇒1−z^4 =1−e^(4iθ) =1−cos(4θ)−isin(4θ) =2 sin^2 (2θ) −2isin(2θ)cos(2θ) =−2isin(2θ){cos(2θ) +isin(2θ)} =2 sin(2θ)(−i) e^(i(2θ)) we have 0<2θ<(π/3) ⇒sin(2θ)>0 ⇒∣1−z^4 ∣ =2 sin(2θ) and arg(1−z^4 ) ≡arg(−i) +2θ [2π] ⇒arg(1−z^4 )≡ −(π/2) +2θ[2π] .](Q43423.png)
Answered by ajfour last updated on 10/Sep/18
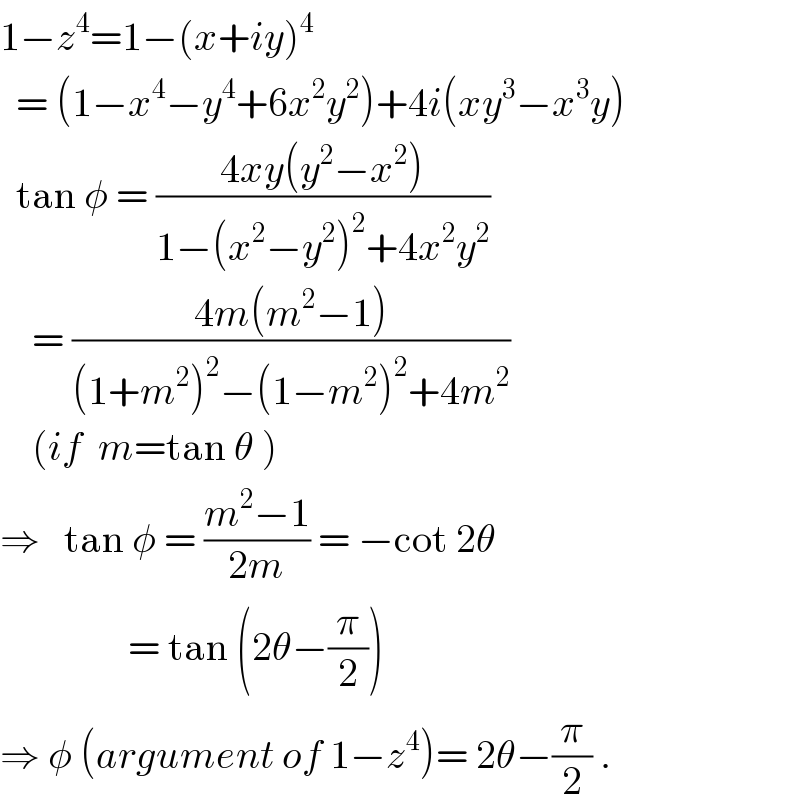
Commented by rahul 19 last updated on 10/Sep/18

Answered by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18
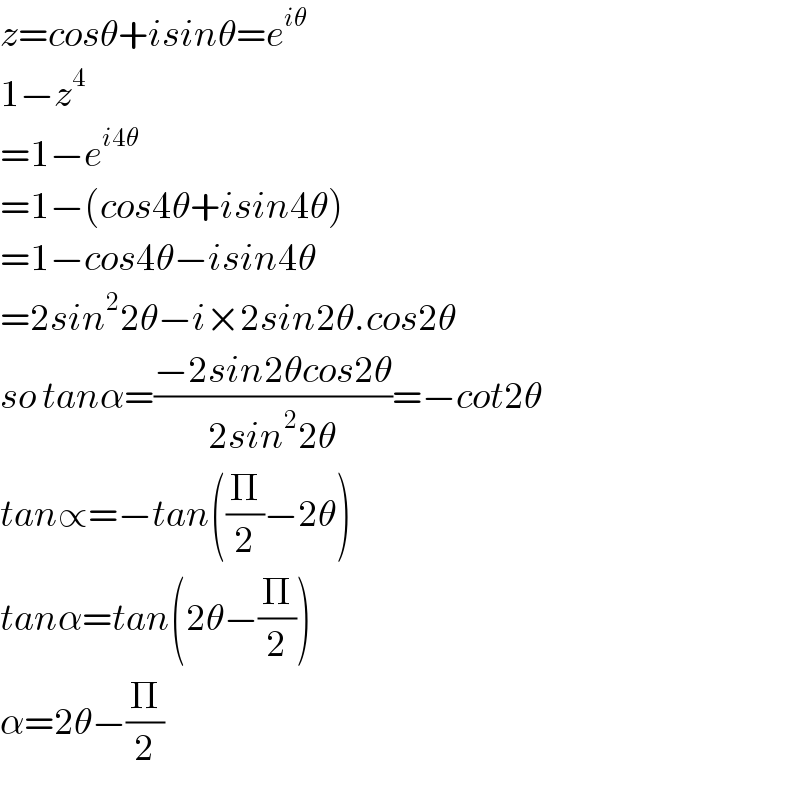
Commented by rahul 19 last updated on 10/Sep/18

Answered by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18