
Question and Answers Forum
IntegrationQuestion and Answers: Page 110




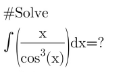
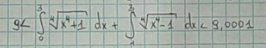
Pg 105 Pg 106 Pg 107 Pg 108 Pg 109 Pg 110 Pg 111 Pg 112 Pg 113 Pg 114
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 110 |

|
| H=∫ ((2017x^(2016) +2018x^(2017) )/(1+x^(4034) +2x^(4035) +x^(4036) )) dx |
| find u_n =∫_1 ^∞ (([ne^(−x) ])/n^3 )dx |
| find ∫_0 ^∞ ((sinx)/([x]))dx |
| ... calculus ... Φ=^? ∫_0 ^( 1) (ln(x))^2 ln((√(−ln(x))) dx |
| η = ∫_0 ^( 1) x^3 (1−x^3 )^(n−1) dx |
| ρ = ∫ ((sin (4x))/(sin^4 (x)+cos^4 (x))) dx |
| ∫_1 ^( π) determinant ((x^3 ,(lnx),(sinx)),((3x^2 ),(1/x),(cosx)),(6,(2x^(−3) ),(−cosx)))dx =? |
| ∫_0 ^1 x^(3/2) (1−x)^(1/2) dx |
| Ω = ∫ (x^2 /( (√((a+bx^2 )^5 )))) dx ; where : a; b >0 |

|
| nice calculus Ω= ∫_0 ^( ∞) ((sin^3 (x))/x^2 )dx=? |
| cos ((π/7))−cos (((2π)/7))+cos (((3π)/7)) =? |
| ∫_e^2 ^( ∞) (dx/(x^3 ln x)) ? |

|

|
| ...nice calculus... prove that ::Ω= ∫_0 ^(π/4) ln(sin(x))d=((−π)/4)log(2)−(G/2) log(2sin(x))=Σ_(n=1) ^∞ ((−1)/n)cos(2nx) Ω= ∫_0 ^( (π/4)) {−log(2)−Σ_(n=1) ^∞ ((cos(2nx))/n)}dx =((−π)/4)log(2)−Σ_(n=1) ^∞ ∫_0 ^( (π/4)) ((cos(2nx))/n)dx =((−π)/4)log(2)−Σ_(n=1) ^∞ [(1/(2n^2 ))sin(2nx)]_0 ^(π/4) =((−π)/4)log(2)−(1/2)Σ_(n=1) ^∞ ((sin(((nπ)/2)))/n^2 ) =((−π)/4)log(2)−(1/2){(1/1^2 )−(1/3^2 ) +(1/5^2 )−..} =((−π)/4)log(2)−(1/2)Σ_(n=1) ^∞ (((−1)^(n−1) )/((2n−1)^2 )) ∴ Ω =((−π)/4)log(2)−(G/2) ✓ G:= catalan constant... |
| Given f(x+(1/x)) = x^4 −(1/x^4 )+2 then ∫_1 ^( 2) (1−x^(−2) )f(x)dx= |
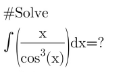
|
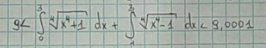
|
| ∫_0 ^( 1) ∫_0 ^( 1) ((dx dy)/(1−xy^3 )) ? |
| ∅ = ∫ (x−2) (√((x+1)/(x−1))) dx |
| β = ∫ ((1+ln (x))/(x.cos^2 (x))) dx |
| Ω = ∫ ln (x+(√(x^2 +a^2 )) )dx |
| ∫ ((sin(2x))/((1 − x)^3 )) dx |
| ∫_0 ^( 1) ∫_0 ^( 1) (1/(1−xy^2 )) dx dy =? |
Pg 105 Pg 106 Pg 107 Pg 108 Pg 109 Pg 110 Pg 111 Pg 112 Pg 113 Pg 114 |