Question and Answers Forum

All Questions Topic List

IntegrationQuestion and Answers: Page 118

Question Number 124059 Answers: 0 Comments: 0

find ∫_0 ^∞ ((x^3 sin(2x))/((x^2 +x+1)^3 ))dx

$f i n d \int_{0}^{\infty} \frac{x^{3} s i n (2 x)}{{(x^{2} + x + 1)}^{3}} d x$

Question Number 124046 Answers: 1 Comments: 0

φ(α) = ∫ ((6α^2 +30α+2)/(4α^2 +20α+25)) dα

$ϕ (α) = \int \frac{6 α^{2} + 30 α + 2}{4 α^{2} + 20 α + 25} d α$

Question Number 124043 Answers: 2 Comments: 0

∫ ((sin x cos x)/(1−2cos 2x)) dx

$\int \frac{\sin x \cos x}{1 - 2 \cos 2 x} d x$

Question Number 124061 Answers: 2 Comments: 0

find ∫_0 ^∞ ((xarctanx)/((x^(2 ) +1)^2 ))dx

$f i n d \int_{0}^{\infty} \frac{x a r c t a n x}{{(x^{2} + 1)}^{2}} d x$

Question Number 124024 Answers: 1 Comments: 0

Question Number 124004 Answers: 1 Comments: 0

∫ (dx/( ((tan x))^(1/3) )) ?

$\int \frac{d x}{\sqrt[3]{\tan x}} ?$

Question Number 123998 Answers: 4 Comments: 0

∫_( 0) ^( ∞) (x^2 /((1+x^2 )^2 )) dx

$\int_{0}^{\infty} \frac{x^{2}}{{(1 + x^{2})}^{2}} d x$

Question Number 123977 Answers: 2 Comments: 0

Question Number 123964 Answers: 0 Comments: 4

∫(√(x^3 +ax+b))dx

$\int \sqrt{x^{3} + a x + b} d x$

Question Number 123967 Answers: 1 Comments: 0

Question Number 123937 Answers: 2 Comments: 0

...nice calculus... prove that:: ∫_((−π)/4) ^(π/4) (((π−4x)tan(x))/(1−tan(x)))dx=^(???) πln(2)−(π^2 /4)

$. . . n i c e c a l c u l u s . . .$ $p r o v e t h a t ::$ $\int_{\frac{- π}{4}}^{\frac{π}{4}} \frac{(π - 4 x) t a n (x)}{1 - t a n (x)} d x \overset{? ? ?}{=} π l n (2) - \frac{π^{2}}{4}$

Question Number 123921 Answers: 3 Comments: 0

∫ (1/( (√x) (x+1)((tan^(−1) (√x))^2 +9)))dx

$\int \frac{1}{\sqrt{x} (x + 1) ({(\tan^{- 1} \sqrt{x})}^{2} + 9)} d x$

Question Number 123900 Answers: 2 Comments: 0

... advanced integral... prove that :: Ω=∫_0 ^( ∞) ((sin^2 (x))/(x^2 (1+x^2 )))dx =(π/4)(1+(π/e^2 ))

$. . . a d v a n c e d i n t e g r a l . . .$ $p r o v e t h a t ::$ $Ω = \int_{0}^{\infty} \frac{{s i n}^{2} (x)}{x^{2} (1 + x^{2})} d x = \frac{π}{4} (1 + \frac{π}{e^{2}})$

Question Number 123896 Answers: 1 Comments: 0

∫_0 ^π ((sin^2 x)/( (√x))) dx

$\underset{0}{\int^{π}} \frac{\sin^{2} x}{\sqrt{x}} d x$

Question Number 123920 Answers: 2 Comments: 0

∫ (((x−1)(√(x^4 +2x^3 −x^2 +2x+1)))/(x^2 (x+1))) dx ?

$\int \frac{(x - 1) \sqrt{x^{4} + 2 x^{3} - x^{2} + 2 x + 1}}{x^{2} (x + 1)} d x ?$

Question Number 123866 Answers: 2 Comments: 0

To mr mjs sir. integral lover ∫_0 ^(π/4) ((tan ((π/4)−x))/(cos^2 x (√(tan^3 x+tan^2 x+tan x)))) dx =?

$T o m r m j s s i r .$ $i n t e g r a l l o v e r$ $\underset{0}{\int^{π / 4}} \frac{\tan (\frac{π}{4} - x)}{\cos^{2} x \sqrt{\tan^{3} x + \tan^{2} x + \tan x}} d x = ?$

Question Number 123859 Answers: 1 Comments: 0

∫sect e^t dt

$\int s e c t e^{t} d t$

Question Number 123793 Answers: 2 Comments: 0

∫ ((4x−1)/(2x^2 −3x+2)) dx ?

$\int \frac{4 x - 1}{2 x^{2} - 3 x + 2} d x ?$

Question Number 123769 Answers: 0 Comments: 1

Question Number 123768 Answers: 0 Comments: 0

Question Number 123767 Answers: 1 Comments: 0

Question Number 123764 Answers: 1 Comments: 0

.... advanced calculus ... prove that:::: Σ_(n=1) ^∞ {((ζ(2n+1))/(4^(n ) (2n+1)))}=ln(2)−γ γ:: euler−mascheroni constant

$. . . . a d v a n c e d c a l c u l u s . . .$ $p r o v e t h a t ::::$ $\underset{n = 1}{\sum^{\infty}} {\frac{ζ (2 n + 1)}{4^{n} (2 n + 1)}} = l n (2) - γ$ $γ :: e u l e r - m a s c h e r o n i$ $c o n s t a n t$

Question Number 123745 Answers: 0 Comments: 0

...advanced calculus... evaluation of: Ω=∫_0 ^( (π/2)) sin(x)log(sin(x))dx by using the euler beta and gamma function: β(p,(1/2))=2∫_0 ^(π/2) sin^(2p−1) (x)dx ((dβ(p,(1/2)))/dp) =2∫_0 ^(π/2) 2sin^(2p−1) (x)ln(sin(x))dx =4∫_0 ^(π/2) sin^(2p−1) (x)ln(sin(x))dx Ω=(1/4)[((dβ(p,(1/2)))/dp)]_(p=1) ((d(β(p,(1/2))))/dp)=(√π)[((Γ′(p)Γ(p+(1/2))−Γ′(p+(1/2))Γ(p))/(Γ^2 (p+(1/2))))]_(p=1) Ω=(1/4)((√π)[((Γ^′ (1)Γ((3/2))−Γ′((3/2))Γ(1))/(Γ^2 ((3/2))))]) =((√π)/4)[((−γ(((√π)/2))−((√π)/2)(2−γ−2ln(2)))/(π/4))] =((√π)/4)∗((√π)/2)(((−γ−2+γ+2ln(2))/(π/4))) =ln(2)−1 ... m.n.july.1970...

$. . . a d v a n c e d c a l c u l u s . . .$ $e v a l u a t i o n o f :$ $Ω = \int_{0}^{\frac{π}{2}} s i n (x) l o g (s i n (x)) d x$ $b y u s i n g t h e e u l e r b e t a a n d g a m m a f u n c t i o n :$ $β (p, \frac{1}{2}) = 2 \int_{0}^{\frac{π}{2}} {s i n}^{2 p - 1} (x) d x$ $\frac{d β (p, \frac{1}{2})}{d p} = 2 \int_{0}^{\frac{π}{2}} 2 {s i n}^{2 p - 1} (x) l n (s i n (x)) d x$ $= 4 \int_{0}^{\frac{π}{2}} {s i n}^{2 p - 1} (x) l n (s i n (x)) d x$ $Ω = \frac{1}{4} {[\frac{d β (p, \frac{1}{2})}{d p}]}_{p = 1}$ $\frac{d (β (p, \frac{1}{2}))}{d p} = \sqrt{π} {[\frac{Γ^{'} (p) Γ (p + \frac{1}{2}) - Γ^{'} (p + \frac{1}{2}) Γ (p)}{Γ^{2} (p + \frac{1}{2})}]}_{p = 1}$ $Ω = \frac{1}{4} (\sqrt{π} [\frac{Γ^{^{'}} (1) Γ (\frac{3}{2}) - Γ^{'} (\frac{3}{2}) Γ (1)}{Γ^{2} (\frac{3}{2})}])$ $= \frac{\sqrt{π}}{4} [\frac{- γ (\frac{\sqrt{π}}{2}) - \frac{\sqrt{π}}{2} (2 - γ - 2 l n (2))}{\frac{π}{4}}]$ $= \frac{\sqrt{π}}{4} * \frac{\sqrt{π}}{2} (\frac{- γ - 2 + γ + 2 l n (2)}{\frac{π}{4}})$ $= l n (2) - 1$ $. . . m . n . j u l y . 1970 . . .$

Question Number 123710 Answers: 2 Comments: 0

calculate ∫_1 ^(√3) (dx/((x^2 +1)^2 (x+2)^5 ))

$c a l c u l a t e \int_{1}^{\sqrt{3}} \frac{d x}{{(x^{2} + 1)}^{2} {(x + 2)}^{5}}$

Question Number 123703 Answers: 1 Comments: 0

Question Number 123676 Answers: 2 Comments: 0

find ∫_0 ^∞ e^(−x) ln(1+e^(2x) )dx

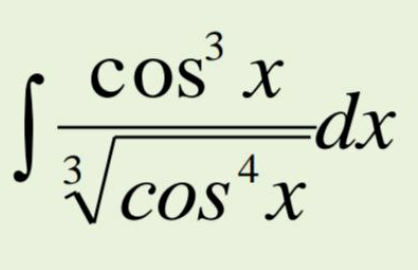
$find \int_{0}^{\infty} e^{- x} \ln (1 + e^{2 x}) dx$

Pg 113 Pg 114 Pg 115 Pg 116 Pg 117 Pg 118 Pg 119 Pg 120 Pg 121 Pg 122

Contact: info@tinkutara.com