Question and Answers Forum

IntegrationQuestion and Answers: Page 134

Question Number 115592 Answers: 0 Comments: 1

Question Number 115558 Answers: 2 Comments: 2

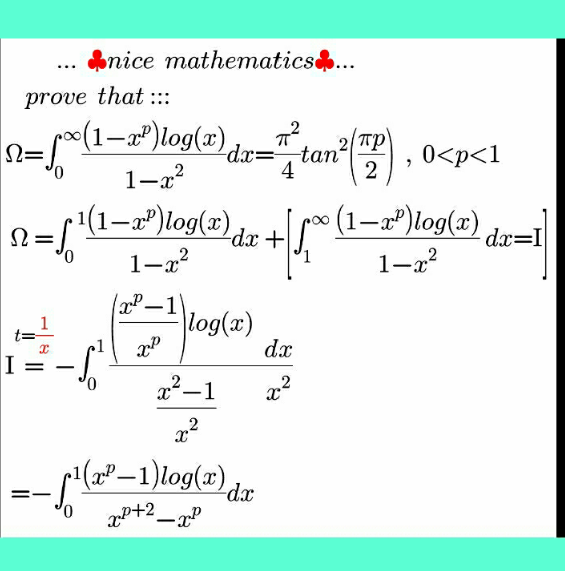
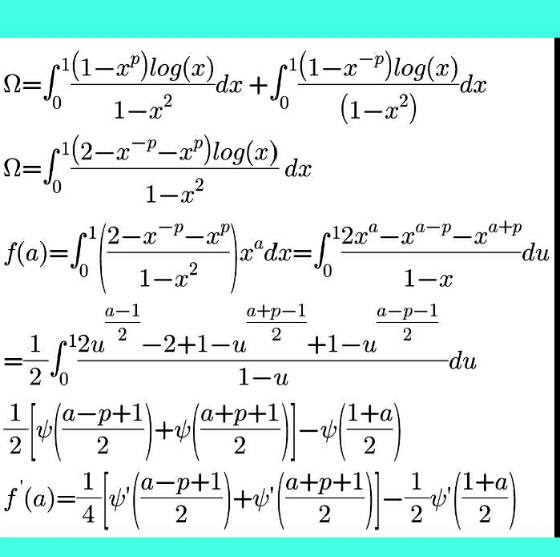
... advanced calculus... evaluate :: ∫_0 ^( ∞) ln(1+ax^2 )ln(1+(b/x^2 ))dx m.n.july

$. . . a d v a n c e d c a l c u l u s . . .$ $e v a l u a t e ::$ $\int_{0}^{\infty} l n (1 + {a x}^{2}) l n (1 + \frac{b}{x^{2}}) d x$ $m . n . j u l y$

Question Number 115533 Answers: 0 Comments: 0

Question Number 115531 Answers: 0 Comments: 0

Question Number 115532 Answers: 0 Comments: 0

Question Number 115507 Answers: 2 Comments: 0

.... ...matematical analysis... prove that ::: a>0 :: [((i : ∫_(0 ) ^( ∞) ((sin^2 (ax))/x^(3/2) ) dx= (√(πa)))),((ii: ∫_0 ^( ∞) ((sin^3 (ax))/( (√x))) dx = ((−1+3(√(3 )))/4) (√((π/(6a)) )) )) ] ...m.n.july.1970...

$. . . . . . . m a t e m a t i c a l a n a l y s i s . . .$ $p r o v e t h a t :::$ $a > 0 :: [\begin{matrix} i : \int_{0}^{\infty} \frac{{s i n}^{2} (a x)}{x^{\frac{3}{2}}} d x = \sqrt{π a} \\ i i : \int_{0}^{\infty} \frac{{s i n}^{3} (a x)}{\sqrt{x}} d x = \frac{- 1 + 3 \sqrt{3}}{4} \sqrt{\frac{π}{6 a}} \end{matrix}]$ $. . . m . n . j u l y . 1970 . . .$

Question Number 115459 Answers: 3 Comments: 0

I= ∫_0 ^1 (dx/((1+x^3 )((1+x^3 ))^(1/(3 )) )) ? I=∫_0 ^(π/2) cos^2 x cos^2 (2x) dx = ?

$I = \underset{0}{\int^{1}} \frac{d x}{(1 + x^{3}) \sqrt[3]{1 + x^{3}}} ?$ $I = \underset{0}{\int^{\frac{π}{2}}} \cos^{2} x \cos^{2} (2 x) d x = ?$

Question Number 115449 Answers: 2 Comments: 0

calculate ∫_(−∞) ^∞ (x^2 /((x^2 −x+1)^3 ))dx

$calculate \int_{- \infty}^{\infty} \frac{x^{2}}{{(x^{2} - x + 1)}^{3}} dx$

Question Number 115464 Answers: 5 Comments: 0

I= ∫_(0 ) ^1 x ln (1+x^2 ) dx ? I=∫ (√(sin x)) .cos^3 x dx ?

$I = \underset{0}{\int^{1}} x \ln (1 + x^{2}) d x ?$ $I = \int \sqrt{\sin x} . \cos^{3} x d x ?$

Question Number 115367 Answers: 1 Comments: 0

solve xy^(′′) −(x^2 +1)y^′ =x^2 sin(2x)

$s o l v e {x y}^{^{″}} - (x^{2} + 1) y^{^{'}} = x^{2} s i n (2 x)$

Question Number 115366 Answers: 2 Comments: 0

calculate ∫_(−1) ^2 (dx/(ch^2 x +sh^2 x))

$c a l c u l a t e \int_{- 1}^{2} \frac{d x}{{c h}^{2} x + {s h}^{2} x}$

Question Number 115365 Answers: 0 Comments: 0

calculate ∫∫_([0,1]^2 ) ((arctan(xy))/( (√(x^2 +y^2 ))))dxdy

$c a l c u l a t e \int \int_{{[0, 1]}^{2}} \frac{a r c t a n (x y)}{\sqrt{x^{2} + y^{2}}} d x d y$

Question Number 115364 Answers: 1 Comments: 0

calculate ∫∫_([0,1]^2 ) (√(xy))(x^2 +y^2 )dxdy

$c a l c u l a t e \int \int_{{[0, 1]}^{2}} \sqrt{x y} (x^{2} + y^{2}) d x d y$

Question Number 115362 Answers: 1 Comments: 0

calculate ∫_0 ^1 ((sinx)/(1+x^2 ))dx

$c a l c u l a t e \int_{0}^{1} \frac{s i n x}{1 + x^{2}} d x$

Question Number 115361 Answers: 1 Comments: 0

find ∫_0 ^∞ ((cos(πx^2 ))/((x^2 +3)^2 ))dx

$f i n d \int_{0}^{\infty} \frac{c o s (π x^{2})}{{(x^{2} + 3)}^{2}} d x$

Question Number 115267 Answers: 1 Comments: 0

If f(x) is a differentiable function defined ∀x∈R such that (f(x))^3 −x+f(x)=0 then ∫_0 ^(√2) f^(−1) (x) dx =

$I f f (x) i s a d i f f e r e n t i a b l e f u n c t i o n$ $d e f i n e d \forall x \in R s u c h t h a t {(f (x))}^{3} - x + f (x) = 0$ $t h e n \underset{0}{\int^{\sqrt{2}}} f^{- 1} (x) d x =$

Question Number 115193 Answers: 1 Comments: 1

...advanced mathematics... :: digamma limit :: if k>0 then prove that lim_(x→0) (1/x)(ψ(((k+x)/(2x))) − ψ((k/(2x)))) =(1/k) ✓ m.n.july.1970...

$. . . a d v a n c e d m a t h e m a t i c s . . .$ $:: d i g a m m a l i m i t ::$ $i f k > 0 t h e n$ $p r o v e t h a t$ ${l i m}_{x \to 0} \frac{1}{x} (ψ (\frac{k + x}{2 x}) - ψ (\frac{k}{2 x})) = \frac{1}{k} ✓$ $m . n . j u l y . 1970 . . .$

Question Number 115169 Answers: 3 Comments: 0

∫ sin^2 (ln x) dx

$\int \sin^{2} (\ln x) d x$

Question Number 115111 Answers: 2 Comments: 0

...mathematical analysis... prove that: Ω=∫_0 ^( 1) (((x^8 +1)ln(x))/(x^(10) −1)) dx=((π^2 ϕ^2 )/(25)) ✓ m.n.july 1970

$. . . m a t h e m a t i c a l a n a l y s i s . . .$ $p r o v e t h a t :$ $Ω = \int_{0}^{1} \frac{(x^{8} + 1) l n (x)}{x^{10} - 1} d x = \frac{π^{2} φ^{2}}{25} ✓$ $m . n . j u l y 1970$

Question Number 115071 Answers: 1 Comments: 0

∫_0 ^(π/2) ((cos x)/( (√(1−sin x)))) dx ?

$\underset{0}{\int^{\frac{π}{2}}} \frac{\cos x}{\sqrt{1 - \sin x}} d x ?$

Question Number 115058 Answers: 1 Comments: 0

.... nice calculus ... a , b , c , d ∈N and (1/a)+(1/b)+(1/c)+(1/d)=(1/2) find max(a+b+c+d) =??? ...m.n.july.1970...

$. . . . n i c e c a l c u l u s . . .$ $a, b, c, d \in N a n d$ $\frac{1}{a} + \frac{1}{b} + \frac{1}{c} + \frac{1}{d} = \frac{1}{2}$ $f i n d$ $m a x (a + b + c + d) = ? ? ?$ $. . . m . n . j u l y . 1970 . . .$

Question Number 115055 Answers: 2 Comments: 0

... nice calculus... evaluation : χ=∫_0 ^( 1) log(1−x).log(1+x) =??? ...m.n.july.197o...

$. . . n i c e c a l c u l u s . . .$ $e v a l u a t i o n :$ $χ = \int_{0}^{1} l o g (1 - x) . l o g (1 + x) = ? ? ?$ $. . . m . n . j u l y . 197 o . . .$

Question Number 115051 Answers: 2 Comments: 6

∫x^2 (√(x^2 −2))dx

$\int x^{2} \sqrt{x^{2} - 2} d x$

Question Number 115030 Answers: 2 Comments: 0

∫_(−(π/2)) ^(π/2) (√(sec x−cos x)) dx =?

$\underset{- \frac{π}{2}}{\int^{\frac{π}{2}}} \sqrt{\sec x - \cos x} d x = ?$

Question Number 115026 Answers: 2 Comments: 0

Solve: ∫_(1/π) ^(1/2) ln ⌊(1/x)⌋dx

$Solve : \int_{1 / π}^{1 / 2} \ln ⌊ \frac{1}{x} ⌋ d x$

Question Number 115009 Answers: 2 Comments: 0

∫_C (e^z /(1−cos z))dz ; C:∣z∣=1

$\int_{C} \frac{e^{z}}{1 - \cos z} d z; C :∣ z ∣= 1$

Pg 129 Pg 130 Pg 131 Pg 132 Pg 133 Pg 134 Pg 135 Pg 136 Pg 137 Pg 138

Contact: info@tinkutara.com