
Question and Answers Forum
IntegrationQuestion and Answers: Page 139



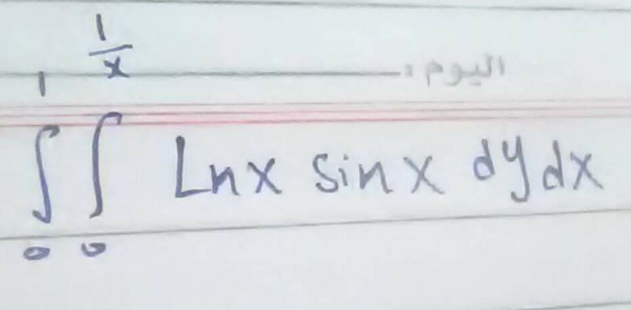
Pg 134 Pg 135 Pg 136 Pg 137 Pg 138 Pg 139 Pg 140 Pg 141 Pg 142 Pg 143
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 139 |
| ∫ln(sin(x)) dx |
| prove that ∫_0 ^∞ (1/(cos(x)+sinh(x)))dx=1.4917. |
| ∫ tan^3 x sec^3 x dx ? |
| ....calculus.... prove that::: if Ω =∫_(0 ) ^( 1) ln(ln(1−(√x) ))dx then Re(Ω) := −γ + ln(2).... m.n. july 1970# |
| please solve : I=∫_0 ^( 1) xlog^2 (((1−x)/(1+x)))dx =??? ...m.n.july 1970.... good luck . |

|
| (√(bemath)) ∫ ((2−cos x)/(2+cos x)) dx |
| I=∫(dx/((x^2 +2x+3)(√(x^2 +x+3)))) = ? my try.. |
| (√(bemath )) (1)∫ ((cos x)/(2−cos x)) dx (2) f(x) = ∣x^3 ∣ ⇒ f ′(x) ? |
| calculate lim_(n→+∞) a_n ∫_0 ^1 x^(2n) sin(((πx)/2))dx with a_n =Σ_(k=1) ^n sin(((πk)/(2n))) |
| f function continue on [0,1] find lim_(n→+∞) (1/n)Σ_(k=0) ^n (n−k)∫_(k/n) ^((k+1)/n) f(x)dx |
| explicite f(x) =∫_0 ^(2π) ln(x^2 −2xcosθ +1)dθ (x≠+^− 1) |
| caoculate ∫_0 ^(π/4) ln(1+2tanx)dx |
| find lim_(x→1^+ ) ∫_x ^x^2 ((ln(t))/((t−1)^2 ))dx |
| find I_n =∫_0 ^(π/4) (du/(cos^n u)) |
| ....advanced mathematics.... please demonstrate that:: Φ =∫_0 ^( 1) xlog(1−x).log(1+x)= (1/4) − log(2) ... m.n.july 1970 # |
| ∫(x)^(1/x) dx=? |
| please evaluate : .... I=∫_0 ^( (π/2)) ((1/(ln(tan(x)))) + (1/(1−tan(x))))dx =??? ::: M. N.july 1970 ::: |
| ∫_0 ^1 ((tan^(−1) x)/(1+x^3 ))dx |
| (√(bemath)) ∫ (dx/( ((x−1))^(1/(3 )) (((x+1)^2 ))^(1/(3 )) )) ? |
| (1) ∫ (((x+1)dx)/(x^4 (x−1))) ? (2) (dy/dx) + (y/(x−2)) = 5(x−2)(√y) |
| following the newest trend − what do I say!? − ahead of it, of course! I post this answer to one of the next questions, look out for it so you won′t miss it! I=I_1 −2I_2 =ξ(5)+Γ(7/3)−2/(√π)+C |

|

|
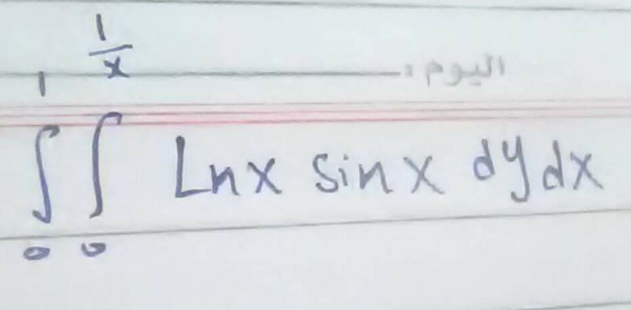
|
Pg 134 Pg 135 Pg 136 Pg 137 Pg 138 Pg 139 Pg 140 Pg 141 Pg 142 Pg 143 |