
Question and Answers Forum
IntegrationQuestion and Answers: Page 206


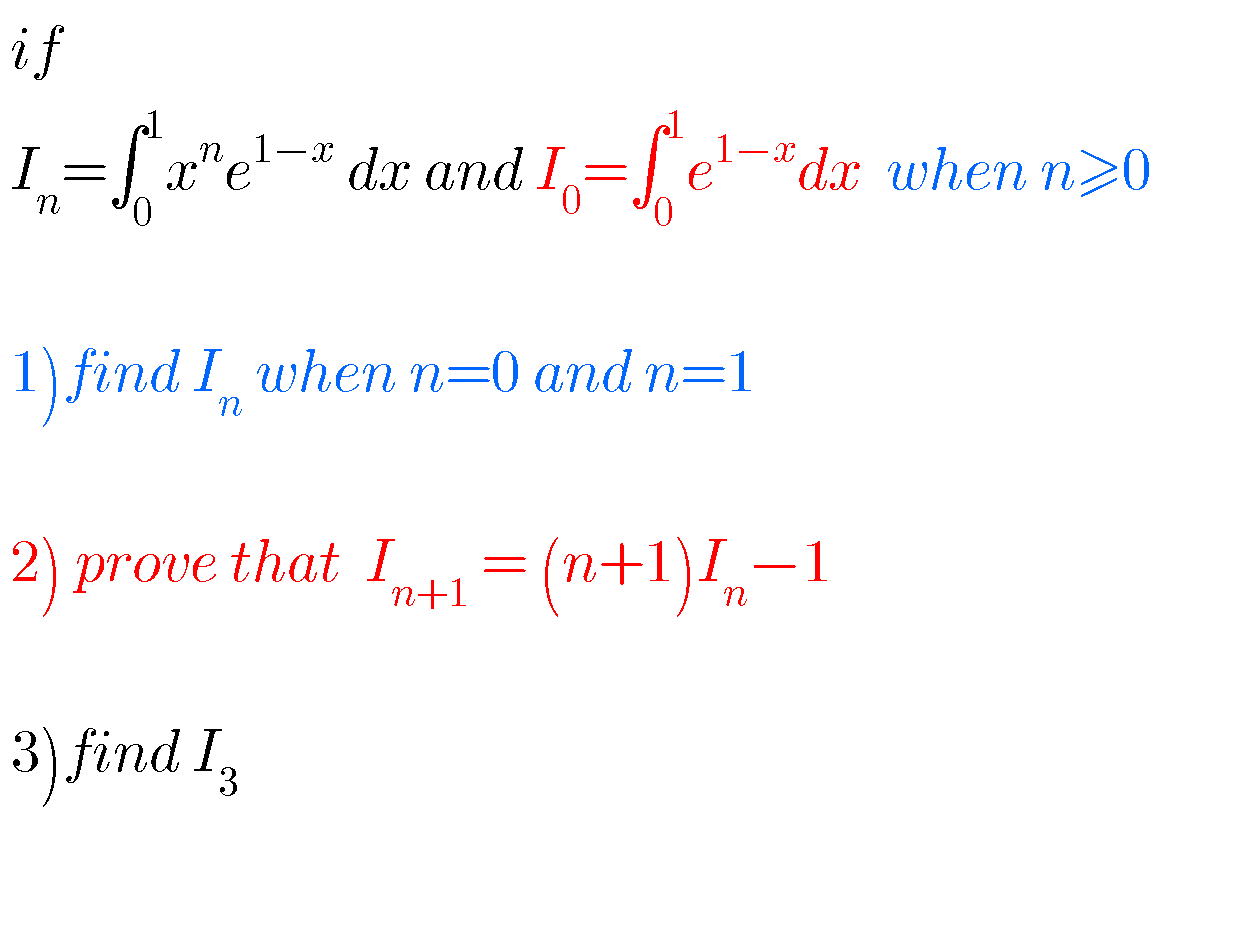
Pg 201 Pg 202 Pg 203 Pg 204 Pg 205 Pg 206 Pg 207 Pg 208 Pg 209 Pg 210
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 206 |
| find f(x)=∫_0 ^1 e^(−t) ln(1−xt^2 )dt with ∣x∣<1 2)calculate ∫_0 ^1 e^(−t) ln(1−(t^2 /2))dt |
| calculate ∫_0 ^∞ ((arctan(2cosx))/(3+x^2 ))dx |
| calculate ∫_0 ^∞ ((cos(artan(2x)))/((3+x^2 )^2 ))dx |
| find ∫_0 ^∞ e^(−t) ln(1+e^t )dt |
| eplcit f(x)=∫_0 ^1 ln(x+t+t^2 )dt with x>(1/4) 2)calculate ∫_0 ^1 ln(t^2 +t +(√2))dt |
| calculate ∫_0 ^∞ ((cos(π +2x^2 ))/((x^2 +4)^2 ))dx |
| calculate ∫_0 ^∞ ((ln(1+e^(−3x^2 ) ))/(3+x^2 ))dx |
| Explicit f(x)= ∫_1 ^∞ ((lnt)/((x^2 +t^2 )^2 )) dt |

|
| ∫(4/(x^2 (√(4−xδϰ)))) ? |
| let 0<a<1 calculate ∫_0 ^∞ ((ln(t)t^(a−1) )/(1+t))dt and ∫_0 ^∞ ((ln^2 (t)t^(a−1) )/(1+t))dt |
| find the sum of Σ_(n=0) ^∞ (n^2 −3n+1)e^(−n) |
| calculate A_n =∫_0 ^∞ ((1+x^n )/(2+x^(2n) ))dx and J_n =∫_0 ^∞ ((2+x^(3n) )/(5+x^(7n) ))dx with n integr natural not 0 |
| calculate f(x)=∫_0 ^π ln(x^2 −2xcosθ +1)dθ with x real. |
| ∫((2x^2 −1+2x(√(x^2 −1)))/(x^2 −x+(x−1)(√(x^2 −1))))dx=? ∫(dx/(x(√(x+1))(√((1−x)^3 ))))=? |

|
| calculate ∫_0 ^∞ xe^(−x^2 ) arctan(x−(1/x))dx |
| calculate ∫_1 ^(3 ) ((x−2)/(√(x^2 +x+1)))dx |
| calculate ∫_0 ^∞ ((lnx)/((x+1)^3 ))dx |
| caoculate ∫_0 ^∞ ((arctan(x^2 −1))/(2x^2 +1))dx |
| calculate ∫_0 ^∞ ((ln(2+x^2 ))/(x^2 −x+1))dx |
| reposting a former question... ∫(((x)^(1/5) −1)/((√x)+1))dx= [t=(x)^(1/(10)) → dx=10(x^9 )^(1/(10)) dx] =10∫((t^9 (t−1))/(t^4 −t^3 +t^2 −t+1))dt= =10∫(t^6 −t^4 −t)dt+10∫((t(t^2 −t+1))/(t^4 −t^3 +t^2 −t+1))dt= =((10)/7)t^7 −2t^5 −5t^2 +(5+(√5))∫(t/(t^2 −((1−(√5))/3)t+1))dt+(5−(√5))∫(t/(t^2 −((1+(√5))/2)t+1))dt= and it′s easy to solve these |
| ∫((x−6)/(x^3 +1))dx |
| calculte ∫ ((x+(√(2+x^2 )))/(x+1−(√(2+x^2 ))))dx |
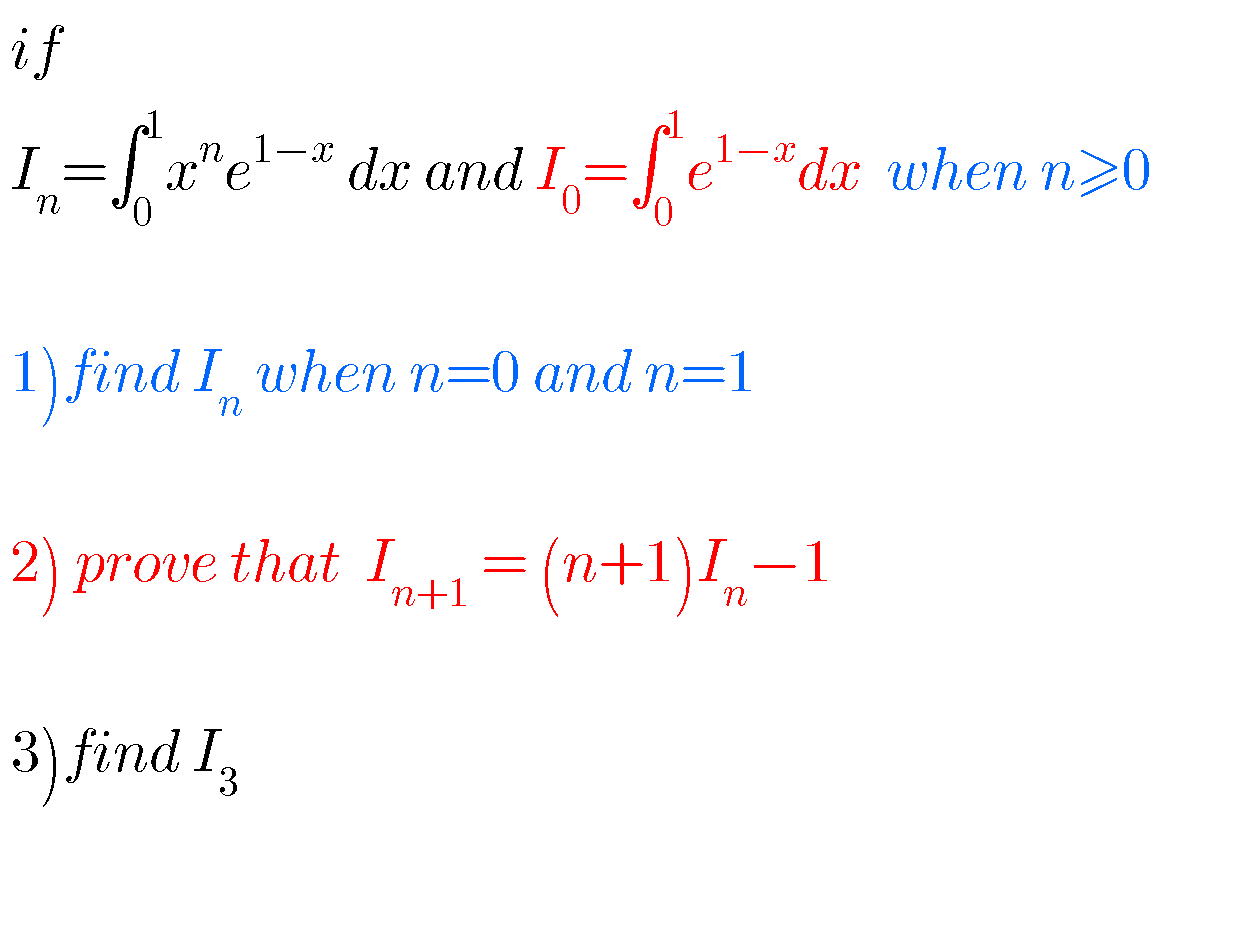
|
| calculate f(x)=∫_0 ^∞ (e^(−xt^2 ) /(4+t^2 ))dt with x>0 |
Pg 201 Pg 202 Pg 203 Pg 204 Pg 205 Pg 206 Pg 207 Pg 208 Pg 209 Pg 210 |