
Question and Answers Forum
IntegrationQuestion and Answers: Page 221







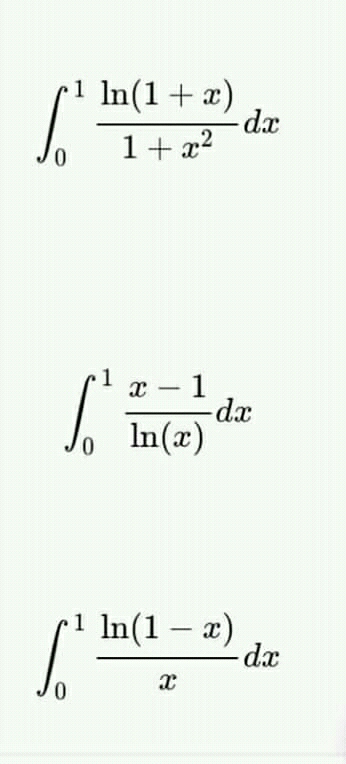
Pg 216 Pg 217 Pg 218 Pg 219 Pg 220 Pg 221 Pg 222 Pg 223 Pg 224 Pg 225
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 221 |
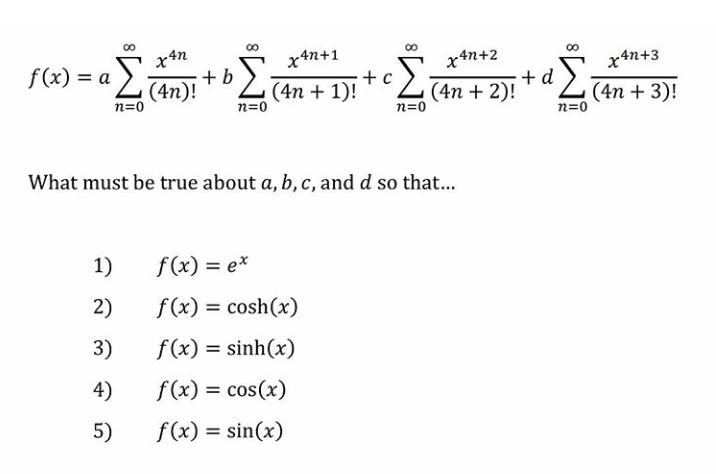
| ∫e^(cos^(−1) (x)) dx |

|
| find U_n = ∫_0 ^(+∞) (((−1)^x )/(2^x^2 (x^2 +4n^2 )))dx (n from N and n≥1) study nature of the serie Σ 2^n^2 U_n |

|
| find f(x) =∫_0 ^(π/4) ln(cost +xsint)dt |

|

|
| let f(x,y)=(x+y)(√(x+y−1)) calculate ∫∫_D f(x,y)dxdy with D ={(x,y)∈R^2 / 1≤x≤2 and 1≤y≤(√3)} |
| find f(α) =∫_1 ^(+∞) ((arctan((α/x)))/(1+x^2 )) dx with α≥0 |
| 1) calculate A_n =∫∫_([1,n[^2 ) sin(x^2 +3y^2 ) e^(−x^2 −3y^2 ) dxdy 2) determine lim_(n→+∞) A_n |
| 1) calculate A_n = ∫∫_([0,n[^2 ) ((dxdy)/(√(x^2 +y^2 +4))) 2)find lim_(n→+∞) A_n |

|
| calculate ∫_0 ^∞ ((arctan(2x)−arctanx)/x)dx |
| I=∫_1 ^e (dx/(x(1+ln^2 x))) |
| find ∫ (dx/(√((x+1)(x+2)(x+3)))) |
| find ∫ (dx/(√(x^2 +x−2))) |
| give the integralA_n = ∫_1 ^(+∞) (dt/(1+x^n )) with n integr and n≥2 at form of serie. |

|
| ∫(((4x+3)dx)/(√(2x^2 +2x−3))) = ? |

|

|
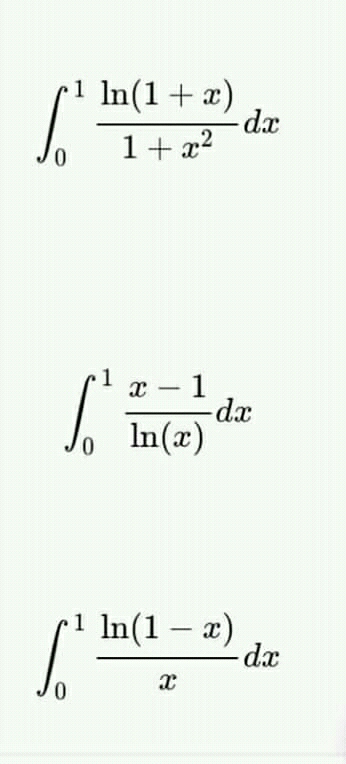
|
| 1) calculate ∫_(−∞) ^(+∞) (dx/(x−a)) with a ∈C 2) find the values of ∫_0 ^∞ (dx/(x^4 +1)) and ∫_0 ^∞ (dx/(x^6 +1)) by using the decomposition inside C(x). |
| f(x) =∫_0 ^1 (dt/(x+e^t )) with 0≤x≤1 1) find aexplicit form of f(x) 2) calculate ∫_0 ^1 (dt/(2+e^t )) 3) find g(x) =∫_0 ^1 (dt/((x+e^t )^2 )) 4) calculate ∫_0 ^1 (dt/((1+e^t )^2 )) 5) give f^((n)) (x) at form of integrals 6) developp f at integr serie. |
| 1) let f(x) =∫_0 ^(+∞) (dt/(t^3 +x^3 )) with x>0 calculate f(x) 2) find also g(x) =∫_0 ^∞ (dt/((t^3 +x^3 )^2 )) 3) find the values of integrals ∫_0 ^∞ (dt/(t^3 +1)) and ∫_0 ^∞ (dt/((t^3 +1)^2 )) 4) give f^((n)) (x) at form of integrals. |
| let f(x) =x∣x∣ 2π periodic odd developp f at fourier series |
Pg 216 Pg 217 Pg 218 Pg 219 Pg 220 Pg 221 Pg 222 Pg 223 Pg 224 Pg 225 |