
Question and Answers Forum
IntegrationQuestion and Answers: Page 226
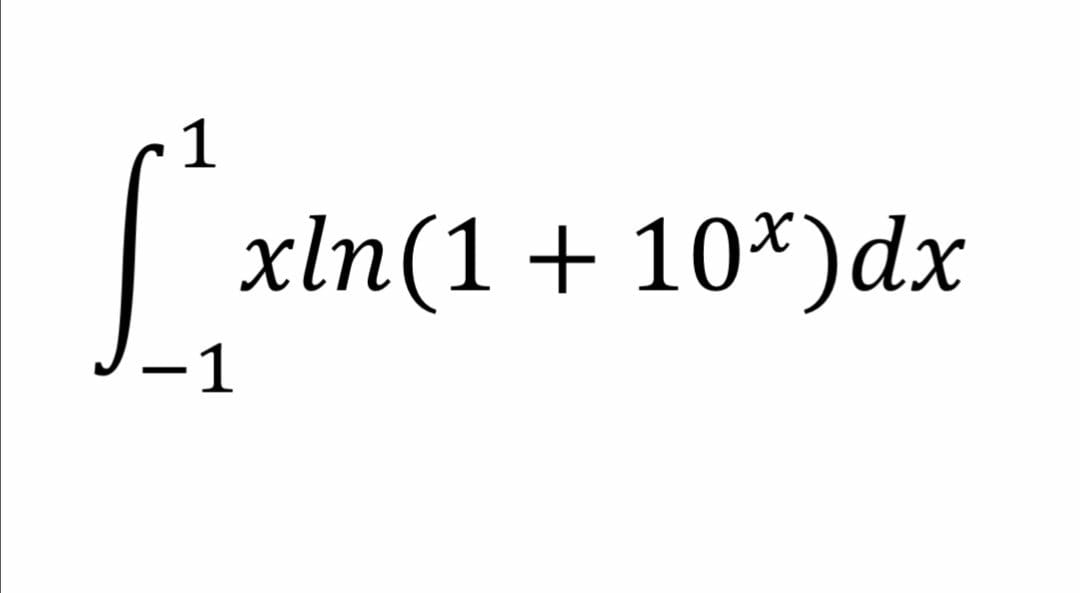

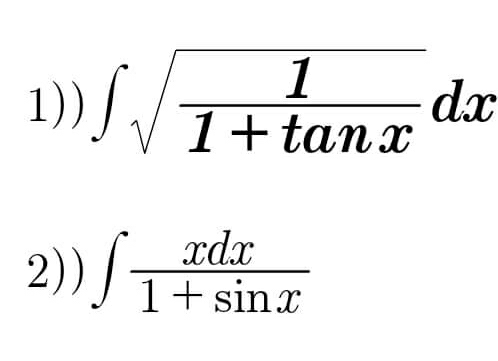
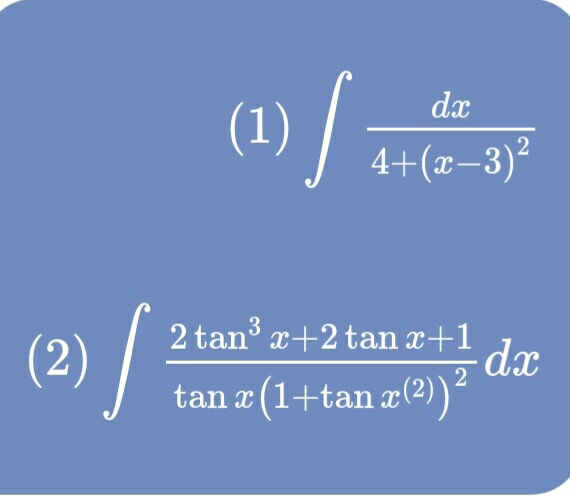

Pg 221 Pg 222 Pg 223 Pg 224 Pg 225 Pg 226 Pg 227 Pg 228 Pg 229 Pg 230
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 226 |
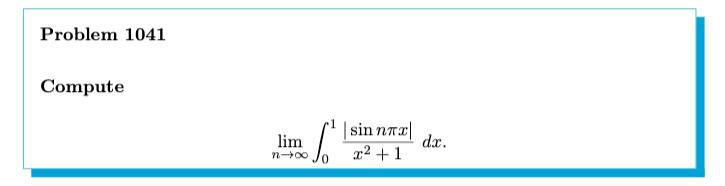
| 1) calculate ∫_0 ^(2π) (dt/(cost +x sint)) wih x from R. 2) calculate ∫_0 ^(2π) ((sint)/((cost +xsint)^2 ))dt 3) find[the value of ∫_0 ^(2π) (dt/(cos(2t)+2sin(2t))) |
| calculate ∫_0 ^(2π) (dx/(2sinx +cosx)) |
| let f(x)=∫_0 ^∞ (t^(a−1) /(x+t^n )) dt with 0<a<1 and x>0 and n≥2 1) determine a explicit form of f(x) 2) calculate g(x) =∫_0 ^∞ (t^(a−1) /((x+t^n )^2 )) dt 3) find f^((k)) (x) at form of integrals 4) calculate ∫_0 ^∞ (t^(a−1) /(9+t^2 )) dt and ∫_0 ^∞ (t^(a−1) /((9+t^2 )^2 )) 5) calculate U_n =∫_0 ^∞ (t^((1/n)−1) /(2^n +t^n )) dt and study the convergence of Σ U_n |
| let A_n =∫_0 ^∞ (x^(a−1) /(1+x^n ))dx with n integr and n≥2 and 0<a<1 1) calculate A_n 2) find the values of ∫_0 ^∞ (x^(a−1) /(1+x^2 ))dx and ∫_0 ^∞ (x^(a−1) /(1+x^3 ))dx 3)calculate ∫_0 ^∞ (dx/((√x)(1+x^4 ))) and ∫_0 ^∞ (dx/((^3 (√x^2 ))(1+x^4 ))) |
| let 0<a<1 find the valueof ∫_0 ^∞ (t^(a−1) /(1+t^2 ))dt |

|
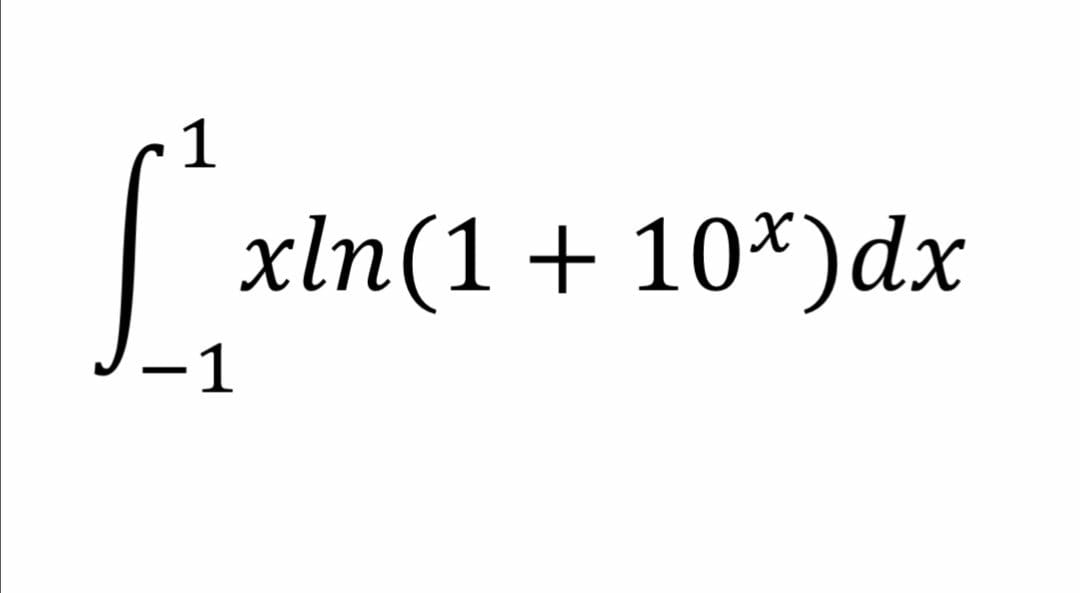
|

|
| prove that ∫sin^n (x) dx , p∈n , p≥2 =− (1/n)cos(x) sin^(n−1) (x) + (p−1)∫sin^(n−2) (x) dx |
| consider the general definite intergral I_n =∫_0 ^(π/2) sin^n xdx a) prove that for n≥2, nI_n =(n−1)I_(n−2) . b) Find the values of i)∫_0 ^(π/2) sin^5 dx ii) ∫_0 ^(π/2) sin^6 dx |
| let f(x)=∫_0 ^∞ (t^(a−1) /(x+t)) dt with x>0 and 0<a<1 1)calculate f(x) 2)calculate g(x)=∫_0 ^∞ (t^(a−1) /((x+t)^2 ))dt 3)find the value of∫_0 ^∞ (t^(a−1) /((1+t)^2 ))dt |
| calculate ∫_(−1) ^1 ((√(1+x^2 )) −(√(1−x^2 )))dx |
| let f(x) =∫_(−∞) ^(+∞) (dt/((t^2 +ixt −1))) with ∣x∣>2 (i^2 =−1) 1) extract Re(f(x)) and Im(f(x)) 2) calculate f(x) 3) find olso g(x) =∫_(−∞) ^(+∞) (t/((t^2 +ixt −1)^2 ))dt 4) find values of integrals ∫_(−∞) ^(+∞) (dt/((t^2 +3it −1))) and ∫_(−∞) ^(+∞) ((tdt)/((t^2 +3it −1)^2 )) 5) give f^((n)) (x) at form of integrals. |
| ∫_1 ^x x^2 −3x(√x)dx =((−716)/(15)) then calculate ∫_x ^(x+1) (1/(x+3))dx |
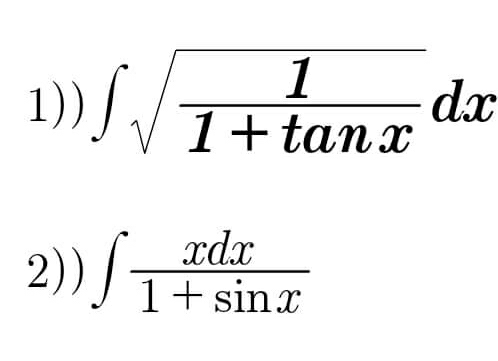
|
| find ∫ (√((x^2 −4x+1)/(x+2)))dx |
| 1) find ∫ ((x+1)/(x^3 −3x −2))dx 2) calculate ∫_4 ^(+∞) ((x+1)/(x^3 −3x +2))dx |
| let f(t) =∫_0 ^∞ ((ln(1+tx))/(1+x^2 ))dx with ∣t∣<1 1) determine a explicit form of f(t) 2) find the value of ∫_0 ^∞ ((ln(1+x))/(1+x^2 ))dx |
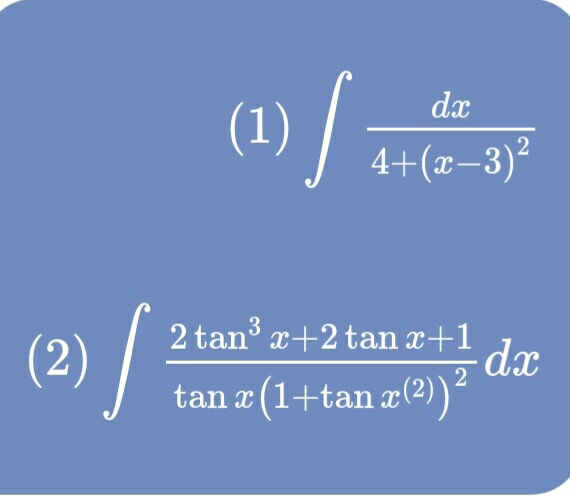
|
| For what values of a and b will the integral ∫_a ^b (√(10−x−x^2 ))dx be at maximum |
| let F(x) =∫_x^2 ^x^3 ((sin(t))/(t+x)) dt 1) calculate lim_(x→0) F(x) and lim_(x→+∞) F(x) 2)calculste lim_(x→0) F^′ (x) and lim_(x→+∞) F^′ (x) |
| ∫x tan(x) dx |
| let B(x,y) =∫_0 ^1 (1−t)^(x−1) t^(y−1) dt 1) study the convergence of B(x,y) 1) prove that B(x,y)=B(y,x) prove that B(x,y) =∫_0 ^∞ (t^(x−1) /((1+t)^(x+y) )) dt 2) prove that B(x,y) =((Γ(x).Γ(y))/(Γ(x+y))) 3) prove that Γ(x).Γ(1−x) =(π/(sin(πx))) for allx ∈]0,1[ |
| ∫_( 0) ^( (π/2)) sin^(−1) (m cosθ) dθ |
| calculate ∫_0 ^∞ x e^(−(x^2 /a^2 )) sin(bx)dx with a>0 and b>0 |

|
Pg 221 Pg 222 Pg 223 Pg 224 Pg 225 Pg 226 Pg 227 Pg 228 Pg 229 Pg 230 |