
Question and Answers Forum
IntegrationQuestion and Answers: Page 239
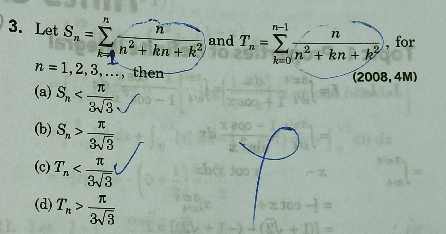
Pg 234 Pg 235 Pg 236 Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242 Pg 243
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 239 |
| let A(ξ) =∫_ξ ^ξ^2 ((arctan(1+ξt)−(π/4))/((√(2+ξt))−(√(2−ξt)))) dt find lim_(ξ →0) A(ξ) . |
| let f(x) =∫_0 ^∞ ((cos(πxt))/((t^2 +3x^2 )^2 )) dt with x>0 1) find a explicit form for f(x) 2) find the value of ∫_0 ^∞ ((cos(πt))/((t^2 +3)^2 ))dt 3) let U_n =f(n) find nature of Σ U_n |
| let f(x) =∫_0 ^(+∞) (dt/((t^2 +x^2 )^3 )) with x>0 1) find a explicit form off (x) 1) calculate ∫_0 ^∞ (dx/((t^2 +3)^3 )) and ∫_0 ^∞ (dt/((t^2 +4)^3 )) 2) find the value of A(θ) =∫_0 ^∞ (dt/((t^2 +sin^2 θ)^3 )) with 0<θ<π. |
| calculate ∫_0 ^π ((2xsinx)/(3 +cos(2x)))dx . |
| find the common area of: { (((x^2 /3)+y^2 =1)),((x^2 +(y^2 /3)=1)) :} |
| a. ∫ [((1−e^x )/(1+e^x ))]^(1/2) dx=? b. ∫ ((lnx)/(√(1+x)))=? c. ∫_( (√e)) ^( e) sin(lnx)dx=? |
| find the value of ∫_(π/3) ^(π/2) (dx/(√(2cos^2 x +3sin^2 x))) |
| find ∫ x^2 (√(25−x^2 ))dx |
| find ∫ (dx/(x^2 (√(9+x^2 )))) |
| find ∫ x^2 (√(4+x^2 ))dx |
| let f(x)=∫_(−∞) ^(+∞) (dt/((t^2 −2xt +1)^2 )) with ∣x∣<1 (x real) 1) determine a explicit form for f(x) 2) find also g(x) =∫_(−∞) ^(+∞) ((tdt)/((t^2 −2xt +1)^3 )) 3) calculate ∫_(−∞) ^(+∞) (dt/((t^2 −(√2)t +1)^2 )) and ∫_(−∞) ^(+∞) ((tdt)/((t^2 −(√2)t +1)^3 )) 4) calculate A(θ) =∫_(−∞) ^(+∞) (dt/((t^2 −2cosθ t+1)^2 )) and B(θ) =∫_(−∞) ^(+∞) ((tdt)/((t^2 −2cosθ t +1)^3 )) with 0<θ <(π/2) . |
| let V_n = ∫_0 ^(1+(1/n)) ((x+1)/(√(2x^2 +3))) dx 1) calculate lim_(n→+∞) V_n 2) find nature of the serie Σ V_n |
| calculate U_n =∫_(π/n) ^((2π)/n) (dx/(2 +sinx)) 1) calculate U_n and lim_(n→+∞) nU_n 2) find nature of Σ U_n |
| 1) calculate f(θ) =∫_0 ^1 (√(t^2 +2sinθt +1))dt with 0≤θ≤(π/2) 2) calculate g(t) =∫_0 ^1 (√(t^2 +2(sinθ)t +1))dθ 3) find also h(θ) =∫_0 ^1 (t/(√(t^2 +2(sinθ)t +1)))dt |
| let f(a) =∫_(π/4) ^(π/3) (√(a+tan^2 x))dx with a>0 1) find a explicit form of f(a) 2) find also g(a) =∫_(π/4) ^(π/3) (dx/(√(a+tan^2 x))) 3) find the values of ∫_(π/4) ^(π/3) (√(2+tan^2 x))dx and ∫_(π/4) ^(π/3) (dx/(√(3+tan^2 x))) |
| is it possible to find the exact value of I? I=∫_0 ^π sin (sin x) dx |
| 1)findF(a)= ∫_0 ^∞ ((cos(ln(2+x^2 )))/(a^2 +x^2 ))dx witha>0 2) find the value of ∫_0 ^∞ ((cos(ln(2+x^2 )))/(4+x^2 ))dx. |
| calculate lim_(x→1) ∫_x ^x^2 ((arctan(t))/(sint))dt . |
| let A_n =∫_0 ^∞ (dt/((e^t +e^(−t) )^n )) calculate A_n interms of n |
| calculate ∫_(−1) ^1 (((x^4 +x^2 +1)^2 +e^x )/(e^x +1))dx |
| let J(x)=∫_0 ^x (t^2 /((√(t+1)) +(√(t+4))))dt find a explicit form of J(x) |
| find ∫_0 ^1 (x+1) ln(x+(√(1+x^2 )))dx |
| calculate ∫_(−1) ^4 ((∣x−1∣+∣x−2∣)/(∣x^2 −9∣ +x^2 +16))dx |
| let F(x) =∫_0 ^x ((1+sint)/(2+cost))dt 1) find a explicite form of f(x) 2) calculate ∫_0 ^π ((1+sint)/(2+cost))dt |
| Given f(x) = f(x + 2016), ∀x ∈ R If ∫_0 ^3 f(x) = 30, then ∫_3 ^5 f(x + 2016) = ... |
|
Pg 234 Pg 235 Pg 236 Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242 Pg 243 |