
Question and Answers Forum
IntegrationQuestion and Answers: Page 246
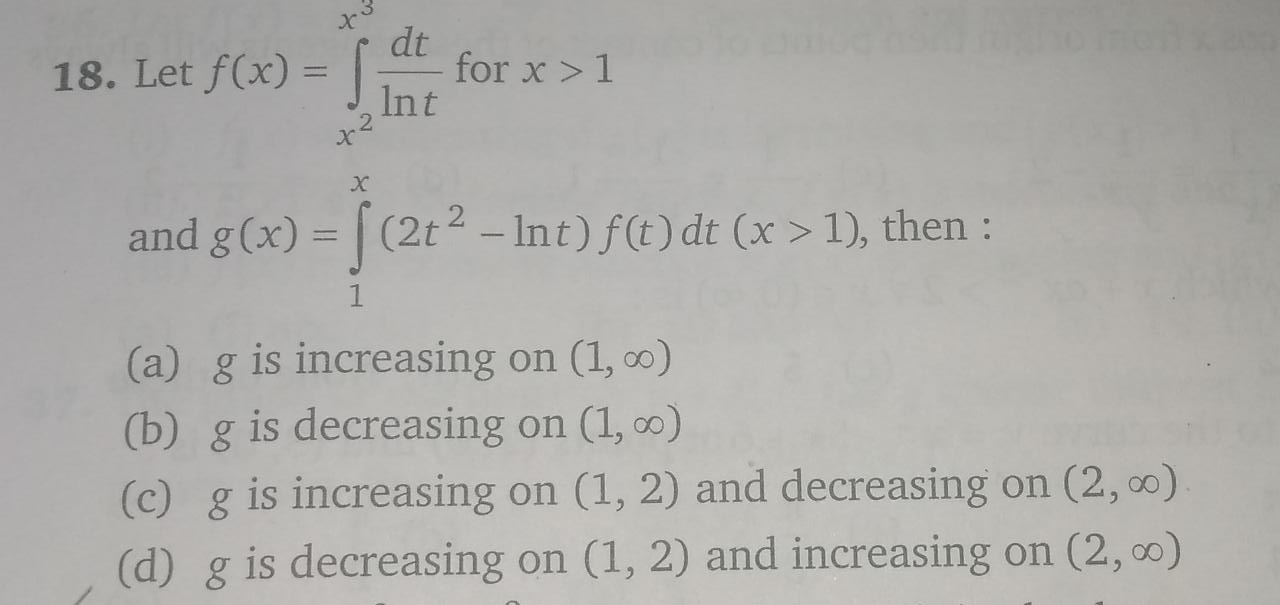

Pg 241 Pg 242 Pg 243 Pg 244 Pg 245 Pg 246 Pg 247 Pg 248 Pg 249 Pg 250
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 246 |
| let f(x) = x∣x∣ , 2π periodic odd developp f at fourier serie . |
| ∫x!dx |
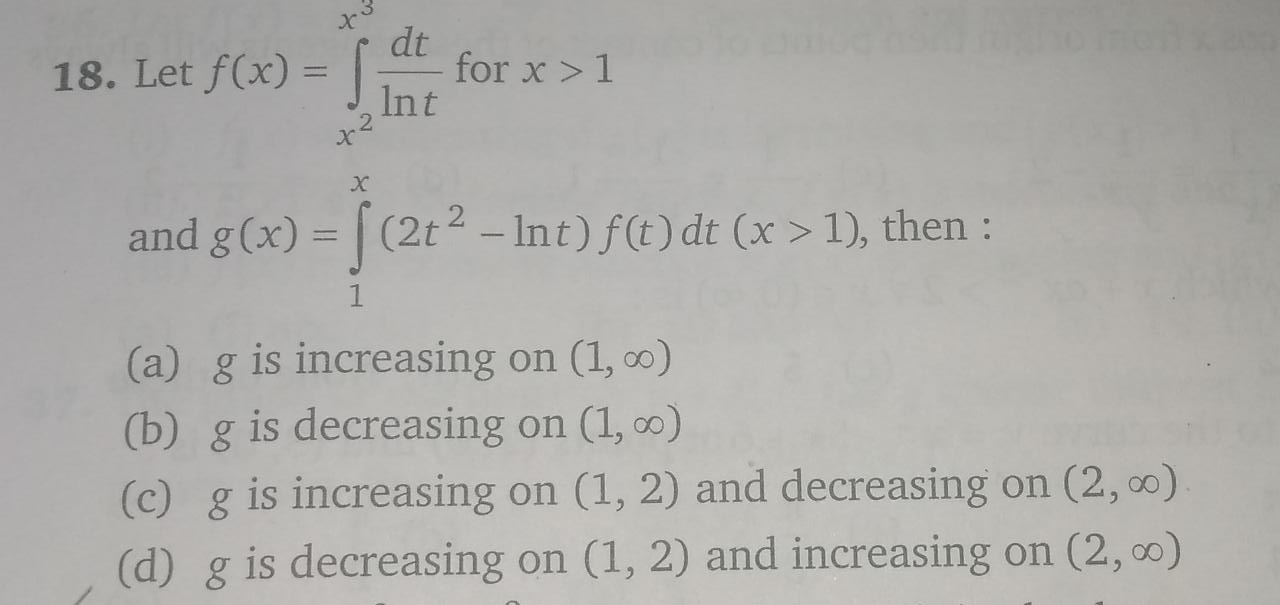
|
| let f(x)=∫_0 ^∞ ((tsin(tx))/(1+t^4 ))dt with x>0 1) find a explicit form of f(x) 2) find the value of ∫_0 ^∞ ((tsin(2t))/(1+t^4 ))dt. |
| calculate ∫_0 ^∞ (t^2 /(e^t −1))dt interms of ξ(3) |
| calculate ∫_0 ^1 (t^2 /(1+t^3 ))dt |
| find ∫_(−∞) ^(+∞) e^(−x^2 ) (√(1+2x^2 ))dx |
| 1) study the function f(x)=ln(x+1−(√x)) 2) determine f^(−1) (x) 3) cslculate ∫ f(x)dx snd ∫ f^(−1) (x)dx 4) dtermine ∫ f^(−1) (x^2 +f(x))dx |
| calculate ∫_0 ^∞ ((e^(−x^2 ) −e^(−x) )/x) dx . |
| calculate A_m =∫_0 ^∞ ((sin(mx))/(e^(2πx) −1)) dx with m>0 |
| 1) calculate A_n =∫_0 ^∞ (x^(n−1) /(e^x +1)) dx with n integr natural (n≥2) 2) find the value of ∫_0 ^∞ (x/(e^x +1))dx |

|
| let f(a)=∫_0 ^1 (dt/((√(x+a)) +3)) 1) calculate f(a) 2) find also ∫_0 ^1 (dt/((√(x+a))((√(x+a)) +3)^2 )) 3) find the values of integrals ∫_0 ^1 (dt/((√(x+1))+3)) and ∫_0 ^1 (dt/((√(x+1))((√(x+1))+3)^2 )) |
| let f(x)=∫_0 ^x t(√(2t−1))dt calculate ∣sup_(1≤x≤2) f(x) −inf_(1≤x≤2) f(x)∣ |
| calculate ∫_0 ^1 ((5^(2x+1) −2^(2x−1) )/(10^x )) dx |
| 1)find U_n = ∫_0 ^(π/4) tan^n tdt with n integr . 2) find lim_(n→+∞) U_n 3) calculate Σ_(n=0) ^∞ U_n |
| find Vn=∫_(1/n) ^((an−1)/n) ((√x)/(√(a−(√x)+x)))dx |
| let A_(n m) =∫_0 ^1 x^n (1−x)^m dx with n and n integrs naturals 1) calculate A_(n m) by using factoriels 2) find Σ_(n,m) A_(nm) |
| find ∫_(−(π/2)) ^(π/2) (√(cosx −cos^3 x))dx |
| let U_n = (((∫_0 ^n e^(−x^2 ) dx)^2 )/(∫_0 ^n e^(−nx^2 ) dx)) 1) calculate lim_(n→+∞) U_n 2) determne nature of Σ U_n and Σ U_n ^3 . |
| 1)let 0<θ<(π/2) and A(θ) =∫_0 ^(π/2) (dx/(√(x^2 +2sinθ x +1))) calculate A(θ) 2) calculate ∫_0 ^(π/2) (dx/(√(x^2 +(√2)x +1))) |
| find ∫_(−(π/4)) ^(π/4) ((xsinx)/(cos^2 x))dx |
| If [x] stands for the gratest integer function the value of ∫_4 ^(10) (([x^2 ])/([x^2 −28x+196]+[x^2 ])) dx is |
| find ∫_0 ^π (x/(2+cosx sinx))dx |
| if u=e^(xyz) then u_(xyx) =? a)u((xyz)^2 +3xyz+1) b)u(3(xyz)^2 +1) c)u((xyz)^2 +2yz+1) please help |
| ∫_0 ^(π/2) (1/(2+cos x)) dx=... |
Pg 241 Pg 242 Pg 243 Pg 244 Pg 245 Pg 246 Pg 247 Pg 248 Pg 249 Pg 250 |