
Question and Answers Forum
IntegrationQuestion and Answers: Page 248


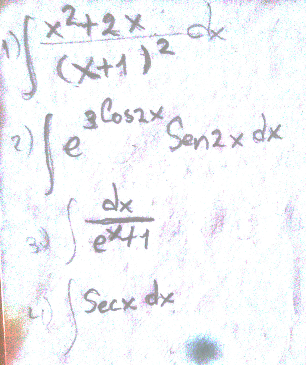
Pg 243 Pg 244 Pg 245 Pg 246 Pg 247 Pg 248 Pg 249 Pg 250 Pg 251 Pg 252
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 248 |
| ∫_(0 ) ^( ∞) (dx/(4−x^2 )) = ? |
| please help integrate ((x+sin x)/(1+cos x))dx |
| find all function f C^2 onR / f(x)+∫_0 ^x (x−t)f(t)dt =1 ∀ x∈R . |
| let I_n (λ) =∫_0 ^π ((vos(nt))/(1−2λcost +λ^2 ))dt 1)calculate I_0 (λ) and I_1 (λ) 2) find relation between I_(n−1) ,I_n and I_(n+1) 3) calculate I_n (λ). |
| find ∫_0 ^∞ ((sin^4 (t))/t^3 ) dt |
| find ∫_0 ^1 ((ln(x))/((√x)(1−x)^(3/2) ))dx |
| calculate A =∫_0 ^(π/3) (du/((1+cos^2 u)^3 )) |
| find ∫_0 ^(π/6) cosx ln(cosx)dx |
| calculate ∫_0 ^(lln(3)) ((sh^2 (x)dx)/(ch^3 (x))) |
| find ∫_0 ^1 arctan(√(1−(x^2 /2)))dx |
| calculate ∫_0 ^1 ^3 (√(x^2 (1−x^3 )))dx |
| calculate ∫_0 ^(π/2) (dt/(1+cosθ cost)) |
| calculate ∫_0 ^(π/2) ((x sinx cosx)/(tan^2 x +cotan^2 x))dx ctanx =(1/(tanx)) |
| let f ∈C^0 (R,R) / ∀ x∈R f(a+b−x)=f(x) 1) find ∫_a ^b xf(x)dx interms of ∫_a ^b f(x)dx 2) calculate ∫_0 ^π ((xdx)/(1+sinx)) |
| 1) calculate U_n =∫_0 ^π (dx/(1+cos^2 (nx))) with n from N 2) f continue from [0,π] to R find lim_(n→+∞) ∫_0 ^π ((f(x))/(1+cos^2 (nx)))dx |
| determine all functions f ∈C^0 (R,R) / ∫_0 ^x f(x)dx =(2/3)xf(x) . |
| determine f ∈C^0 ([0,1],R) verifying ∫_0 ^1 f(x)dx =(1/3) +∫_0 ^1 (f(x^2 ))^2 dx |
| 1) decompose at simple elements U_n = ((n x^(n−1) )/(x^n −1)) 2) calculste ∫_0 ^(2π) (dt/(x−e^(it) )) |
| find inf_((a,b)∈R^2 ) ∫_0 ^1 x^2 (ln(x)−ax−b)^2 dx |
| find ∫ (dx/((1−x^2 )(1−x^3 ))) 2) calculate ∫_2 ^(√5) (dx/((1−x^2 )(1−x^3 ))) |

|
| find f(a) =∫_a ^(+∞) (dx/((1+x^2 )(√(x^2 −a^2 )))) with a>0 |
| Let f(x)= ∫_2 ^( x) (dt/(1+t^6 )). Prove that : (1/(730))<f(3)<(1/(65)). |
| Find the function whose first derivative is 8−(5/(x^2 )^(1/3) ) the initial conditions f(8)=−20 |

|
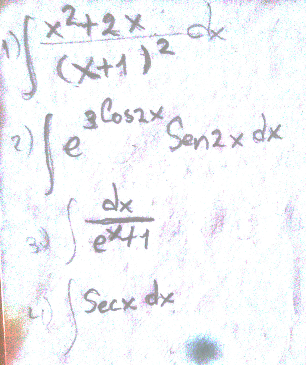
|
Pg 243 Pg 244 Pg 245 Pg 246 Pg 247 Pg 248 Pg 249 Pg 250 Pg 251 Pg 252 |