
Question and Answers Forum
IntegrationQuestion and Answers: Page 252
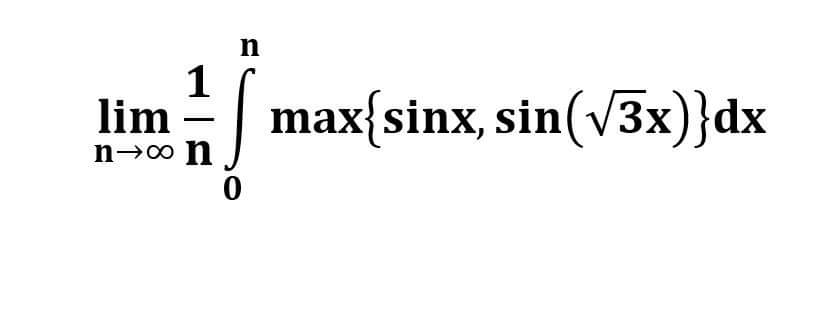
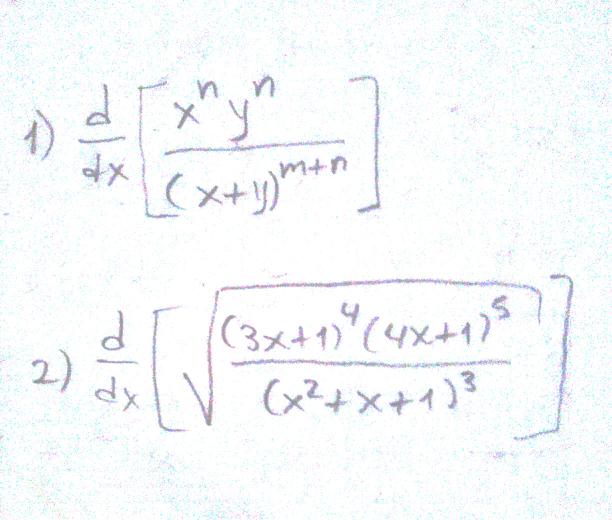


Pg 247 Pg 248 Pg 249 Pg 250 Pg 251 Pg 252 Pg 253 Pg 254 Pg 255 Pg 256
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 252 |
| find A_n =∫_0 ^(π/2) ((1−cos(n+1)x)/(2sin((x/2))))dx . |
| prove that ∫_0 ^∞ (((1+t)^(−(3/4)) −(1+t)^(−(1/4)) )/t)dt is convergent and find its value . |
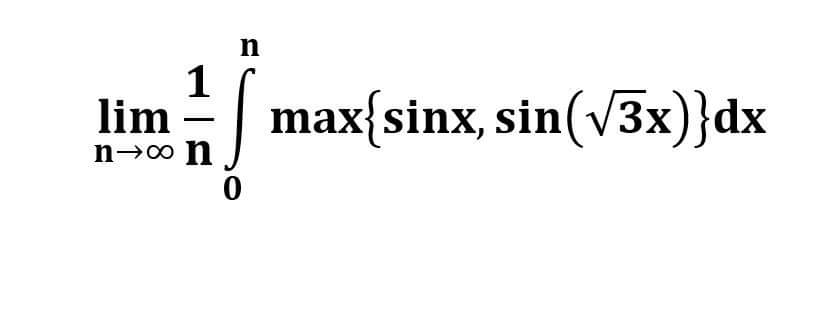
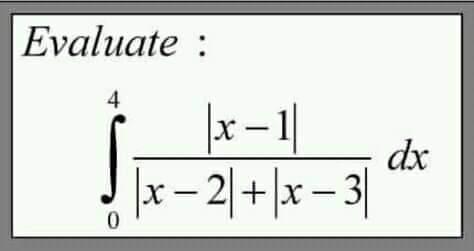
|
| Evaluate ∫_0 ^1 ((Log(x))/(x^2 +2x+3)) dx |
| let f(x)=∫_0 ^(2π) ((sin(2t))/(1+x cos(t)))dt 1) find a explicit form of f(x) 2) find also g(x)=∫_0 ^(2π) ((sin(2t)cost)/((1+xcost)^2 ))dt 3)find the value of ∫_0 ^(2π) ((sin(2t))/(1+3 cos(t)))dt and ∫_0 ^(2π) ((cost sin(2t))/((1+3cost)^2 ))dt . |
| let f(x) =∫_(1/2) ^1 (dt/(2+ch(xt))) 1) find a explicit form of f(x) 2) calculate g(x)=∫_(1/2) ^1 ((tsh(xt))/((2+ch(xt))^2 ))dt 3) find the value of ∫_(1/2) ^1 (dt/(2+ch(3t))) and ∫_(1/2) ^1 ((tsh(2t))/((2+ch(2t))^2 ))dt 4) let u_n =∫_(1/2) ^1 (dt/(2+ch(nt))) study the convergence of Σu_n and Σ(u_n /n) . |
| calculate A_λ =∫_0 ^∞ ((cos(λsinx)−sin(λcosx))/(x^2 +λ^2 ))dx λ from R. |
| q.....∫(dx/(sin x cos x+2cos^2 x)), please solve |
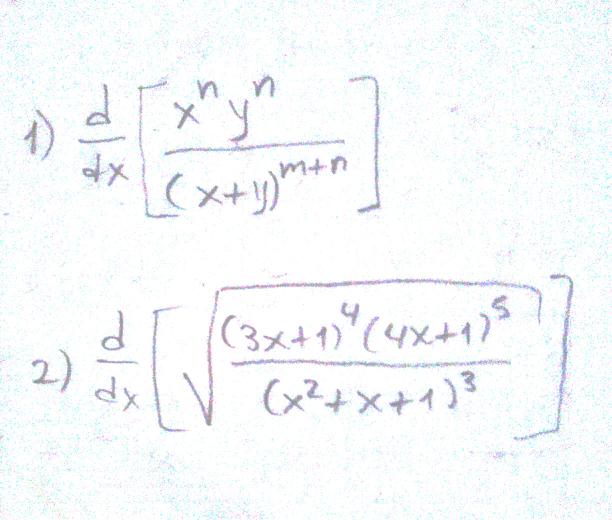
|
| find ∫ ((sin(πx))/(3 +cos(2πx)))dx |
| find lim_(x→0) ∫_(x+1) ^(2x+1) ((tarctan(t^2 +1))/(1+(1+t^2 )^2 ))dt |
| calculate lim_(x→0) ∫_x ^x^2 ((ln(1+t))/(sin(t)))dt |
| calculate ∫ ((arctan(x))/(√(1+x^2 )))dx |
| calculate ∫_0 ^∞ ((sin(2cos(x^2 +1)))/(1+x^2 ))dx |
| calculate ∫_0 ^∞ ((sin(cosx))/(x^2 +3))dx |
| calculate ∫_0 ^∞ ((cos(sin(x^2 )))/(1+2x^2 ))dx |

|
| solve this ∫(2 sinx+cosx)/(2+3sinx+sin^(2x) ) dx |

|
| let y>0 give ∫_0 ^∞ (x^y /(e^x −1))dx at form of series. |
| calculate A =∫_0 ^1 (1+x^2 )(√(1−x^2 ))dx −∫_0 ^1 (1−x^2 )(√(1+x^2 ))dx |
| let W(x) =∫_(−∞) ^(+∞) ((arctan(xt^2 ))/(2+t^2 ))dt 1) find a explicit form of f(x) 2) find the value of ∫_(−∞) ^(+∞) (t^2 /((2+t^2 )(1+x^2 t^4 )))dt . |
| calculate ∫_(−∞) ^(+∞) (((x^2 −3)sin(2x^2 ))/((x^2 +1)^3 ))dx |

|
| let f(α) =∫_(−∞) ^(+∞) ((cos(αx^3 ))/(x^2 +8)) dx 1)calculate f(α) 2) calculate ∫_(−∞) ^(+∞) ((cos(2x^3 ))/(x^2 +8))dx . |
| find the value of ∫_(−∞) ^(+∞) ((2x+1)/((x^2 +i)(x^2 +4)))dx (i^2 =−1) |
Pg 247 Pg 248 Pg 249 Pg 250 Pg 251 Pg 252 Pg 253 Pg 254 Pg 255 Pg 256 |