
Question and Answers Forum
IntegrationQuestion and Answers: Page 300

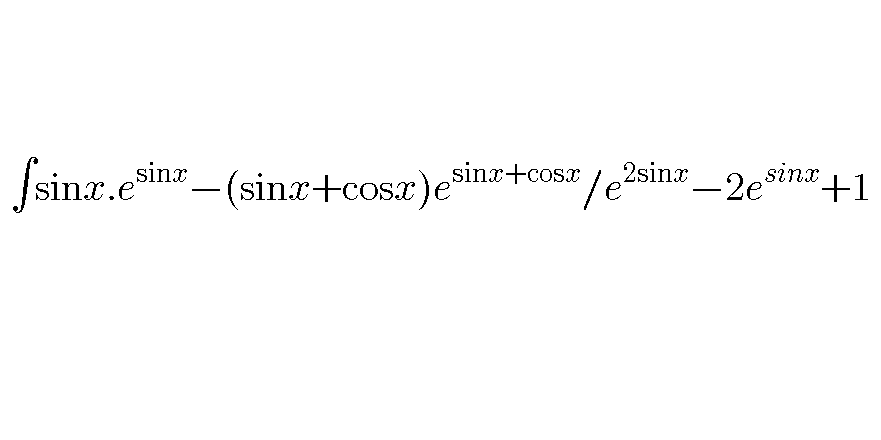
Pg 295 Pg 296 Pg 297 Pg 298 Pg 299 Pg 300 Pg 301 Pg 302 Pg 303 Pg 304
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 300 |
| find ∫_(−∞) ^(+∞) (dt/(1 +(t+2i)^2 )) . |
| cslculate ∫_0 ^∞ (t −[t])e^(−3t) dt . |
| find ∫_0 ^∞ arctan(2x) (e^(−tx) /x) dc with t>0 2) calculate ∫_0 ^∞ ((arctan(2x))/x) e^(−x) dx. |
| finf ∫_0 ^(+∞) (dx/(1+x^2 +x^4 )) |
| find ∫_0 ^(2π) ((cos^2 x)/(1+3sin^2 x))dx . |
| calculate ∫_(−∞) ^(+∞) (dt/((1+it)(1+it^2 ))) . |
| calculate ∫_1 ^(+∞) (dt/(t^2 (√(1+t^2 )))) . |
| calculate ∫_0 ^(π/2) (dt/(1+a cos^2 t)) . |
| find ∫_0 ^∞ (dx/(1+x^3 )) . |
| let give f(x)= ∫_0 ^∞ ln(1 +(x/t^2 ))dt with ∣x∣<1 find a simple form of f(x). |
| find ∫_0 ^∞ (((x+1)(√x))/(2+x^2 ))dx. |

|
| let give f(x)=∫_0 ^(π/2) ((ln(1+xtant))/(tant))dt find a simple form of f(x) 2)calculate ∫_0 ^(π/2) ((ln(1+2tant))/(tant))dt . |
| plzz help ne differentiate between ∫sin(2x)= −(1/2)cox(2x)+c is not change to ∫2sin(x)cos(x) but ∫_b ^a sin(2x)= is change to ∫_b ^a 2sin(x)cos(x) |
| ∫_1 ^2 ∫_0 ^1 ((ln(x+y))/((x+y))) dx dy |
| calculate ∫_0 ^(2π) (dx/(1+2cosx)) . |
| find f(x)= ∫_0 ^π ((sin^2 t)/(1−2xcost +x^2 ))dt with ∣x∣<1 . |
| find ∫_0 ^∞ ((√(t )) −2(√(t+1)) +(√(t+2))) dt |
| find ∫_0 ^α (√(tanx)) dx with 0<α<(π/2) . |
| find ∫_0 ^∞ (dx/((x^2 +1)(x^2 +3x +1))) |
| find ∫_0 ^∞ ln(((1+t^2 )/t^2 ))dt |
| calcilate ∫_0 ^1 ((ln(1−x^2 ))/x^2 )dx |
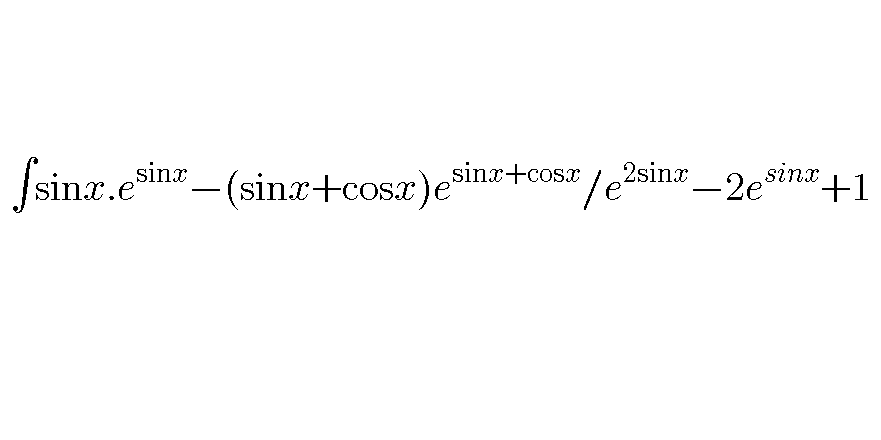
|
| let α∈R and x^2 ≠1 find the value of f(x) = ∫_0 ^π ln(x^2 −2x cost +1)dt calculate f(x). |
| let F(x) = ∫_0 ^π ln(1+xcosθ)dθ .with ∣x∣<1 find F(x) . |
Pg 295 Pg 296 Pg 297 Pg 298 Pg 299 Pg 300 Pg 301 Pg 302 Pg 303 Pg 304 |